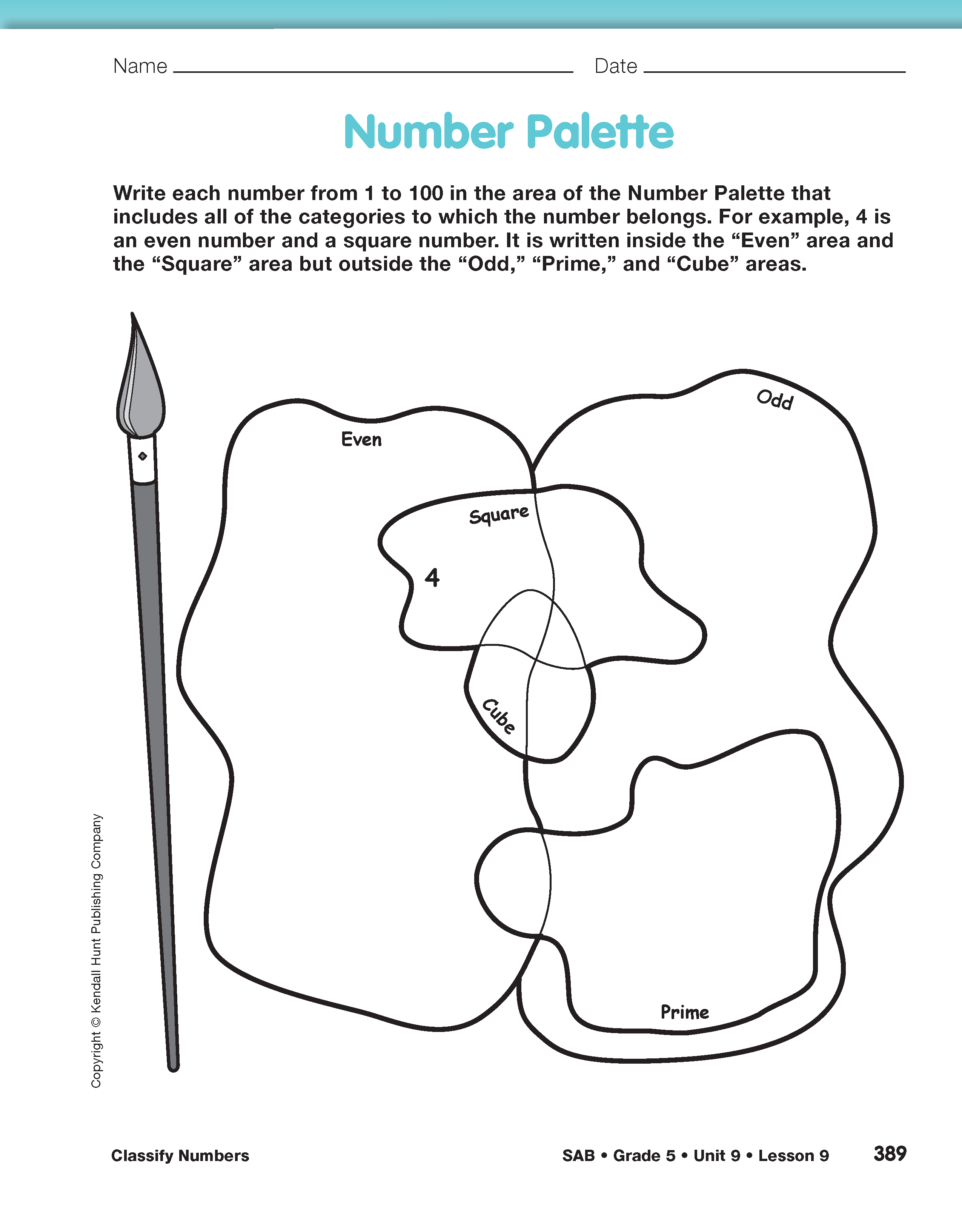
Introduce the Number Palette page in the Student Activity Book. Begin by reading the instructions at the top of the page.
- Could 4 be written in any other area of the diagram? (no)
Students may suggest other areas that might work. Show that any other area would either exclude one of the categories to which 4 belongs, or else would include 4 in a category to which it does not belong.
- Where would 10 go? (10 is an even number, but 10 is neither a square, a cube, nor a prime, so it should be written in the part of the Even area that is not also in the Square, Cube, or Prime area.)
- Where would 81 go? (81 is an odd number and a square number. It should be written in the part of the Square area that is also in the Odd area, but outside of the Cube and Prime areas.)
Direct students to complete the Number Palette page for all of the other numbers between 1 and 100. Students may complete this page independently or with a partner.
Use the Number Palette page with Feedback Box in the Student Activity Book to assess students' abilities to identify and categorize prime, composite, and square numbers [E1] and multiples of three [E2].
Students can play Number Bingo for targeted practice categorizing prime, composite, and square numbers.

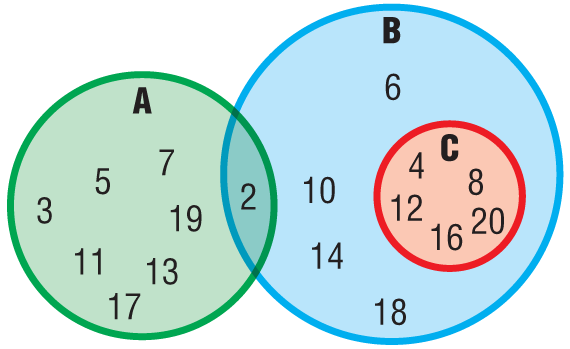
The diagram shown in the Number Palette page is an informal version of a Venn diagram. A Venn diagram can be used to show how different groups, or sets, of numbers relate to one another. These diagrams typically use circles to show sets of numbers or objects. For example, in Figure 1, the circle marked A contains the set of all prime numbers from 1 to 20. The circle marked B contains the set of all even numbers from 1 to 20. Any numbers that are both prime and even are shown in the space that is both inside A and inside B. In this case, 2 is the only number that is both prime and even, so it is shown inside the intersection of A and B. The circle marked C contains the set of all multiples of 4 from 1 to 20. Notice that C is entirely inside B, since all multiples of 4 are also even numbers.