Unit 11 Contents

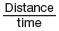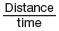Since the ratios for are equivalent (even though Distance and time can be different), the variables distance and time are in proportion. We can write proportions using the ratios in the table. For example,

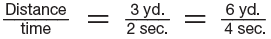
Answer the following questions about the variables if the ratio of distance to time is 3 yards to 2 seconds.

1. Show how you know that all the ratios in the table are equivalent to one another.
1. Graph the variables in the data table. Put time (t) on the horizontal axis and distance (D) on the vertical axis.
2. Describe your graph. Tell where it meets the vertical axis. Is it a straight line or a curve? Does it go up or down as you read from left to right?
3. Choose two points from your graph that lie on grid lines. Write the ratio of distance to time for each point. Are the two ratios equivalent?
1. If you double the time (t), what happens to the distance (D)? Give an example.
2. If you triple the time (t), what happens to the distance (D)? Give an example.
3. If you multiply the time by any number, what happens to the distance traveled? For example, if a student walks 3 yards in 2 seconds, how far will the student walk in 8 sec. (4 2 sec.)?
5. How far does the walker travel in 1 second?
6. If you know the time, how can you find the distance?