Lesson 1

Working with Groups

Est. Class Sessions: 2

Developing the Lesson

Part 2: Even and Odd Numbers

Distribute tiles or other counters to students. Ask students to grab a handful of counters and determine if the number of counters is odd or even.

Ask:

X
  • How many counters did you grab?
  • Is the number in your handful an even or odd number?
  • How do you know? (See the Sample Dialog for possible responses.)
  • Write a number sentence for your groupings.

Explain that the numbers added together are addends and the answer is the sum.

As students express the number of counters as an even or odd number, write the number sentences on the chart that you prepared earlier. See Materials Preparation.

Ask:

X
  • What do you notice about the number sentences under the even and odd columns? (The odd number sentences are the sum of 2 equal addends plus one and the even number sentences are the sum of 2 equal addends.)
  • What are some other number sentences with sums to 20 that we can add to the chart?

Add number sentences with sums to 20 that were not on the chart. See Figure 4 for a sample chart.

Help students understand that when they place objects in pairs, it will always make two equal groups. Have students place
10 tiles into rows of two.

Ask:

X
  • How many rows of 2 do you have? (5 rows of 2)
  • What is your number sentence?
    (2 + 2 + 2 + 2 + 2 = 10)
  • Turn your tiles so you can see 2 rows of 5.
  • What is your number sentence? (5 + 5 = 10)
  • Is 10 an even number? How do you know? (Yes; I have two equal addends 5 + 5.)
X

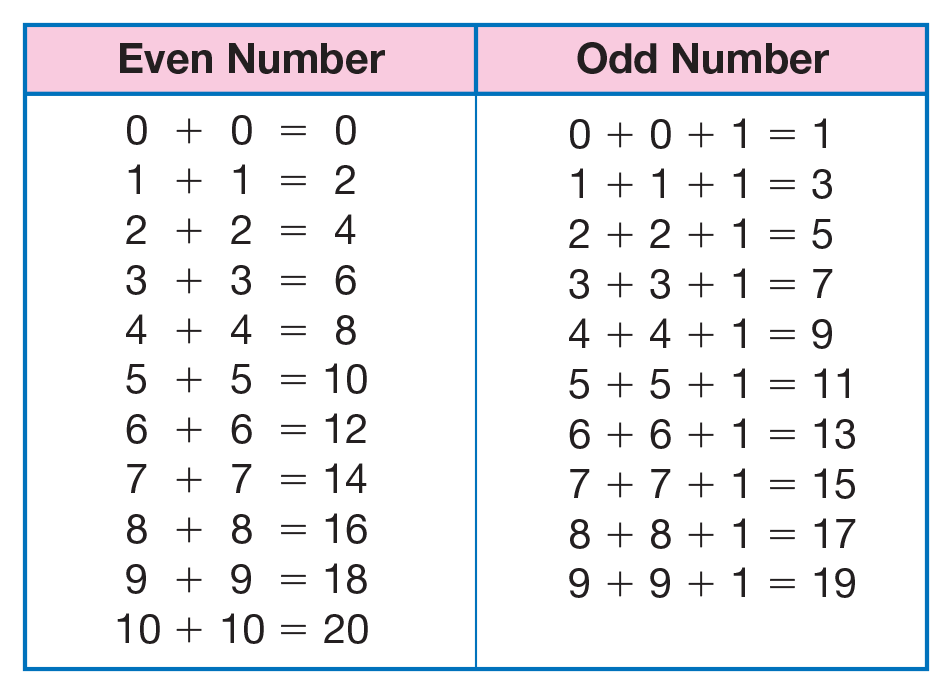
Use this sample dialog to guide your discussion of odd and even numbers.

Teacher: How many counters did you grab?

Lily: I have 20 in my handful.

Teacher: Is 20 an even or odd number? How do you know?

Lily: Twenty is an even number. I know because if I put them into two equal groups, there are no leftovers: 10 + 10 = 20.

Teacher: Did anyone grab a different number of counters?

Chloe: I grabbed 19. My number is odd. I know because I made pairs. There were 9 pairs but one didn’t have a partner, so I know that 19 is odd.

Teacher: What number sentence did you write to represent your problem?

Chloe: I wrote 2 + 2 + 2 + 2 + 2 + 2 + 2 + 2 + 2 + 1 = 19.

Teacher: If you made two equal groups, what would your number sentence be?

Chloe: I could make two groups of 9 and I would have 1 left over. That’s 9 + 9 + 1 = 19.

Teacher: Who discovered a different way to figure out if your number is odd or even?

Brandon: I used connecting cubes. I only grabbed 8. Eight is an even number. I know because I made two columns of connecting cubes that were the same height.

Teacher: How could you represent your two columns with numbers?

Brandon: There’s 4 in one column and 4 in the other, so that’s 4 + 4 = 8.

Teacher: Who has a different strategy?

Max: I grabbed 12. I know that if I double 6, I’ll get 12. My number sentence is 6 + 6 = 12.

Teacher: Let’s write all your number sentences on a chart with two columns: one for even numbers and one for odd numbers. What do you notice about the number sentences for the even numbers and the ones for the odd numbers?

Mark: I notice that the number sentences under the even numbers have 2 equal addends and the ones under the odd numbers have one leftover.

Teacher: Great! Mark noticed that an even number is the sum of two equal addends.

X

Students often learn that even numbers have the digits 0, 2, 4, 6, and 8 in the ones column and odd numbers have the digits 1, 3, 5, 7, and 9 in the ones column without really understanding why this is true. Giving students opportunities to group objects in pairs, skip count objects by twos, and work with doubles will help students understand why an even number is the sum of two equal addends.

ADVENTURE BOOK:
STUDENT ACTIVITY BOOK:
Master:
Number sentences for even and odd numbers
X
+