Introduce the Multiplication Sentence.
Present the following problem to students:
- Each day the zookeeper needs to give four bunches of carrots to each of the three monkey
habitats. How many bunches of carrots does he need to order?
Have students use connecting cubes to model the problem. As they share answers, point to the connecting cubes to show what each number means.
- What is an addition number sentence that describes this problem? (4 + 4 + 4 = 12)
Rotate the cubes to demonstrate the related number sentence.
- What is a related addition number sentence for
4 + 4 + 4 = 12? (3 + 3 + 3 + 3 = 12)
Introduce the multiplication number sentences 3 × 4 = 12 and
4 × 3 = 12.
- For the number sentence 4 + 4 + 4 = 12, how many fours are there? (3)
- For the number sentence 3 + 3 + 3 + 3 = 12, how many threes are there? (4)
- We can write the multiplication number sentences
3 × 4 = 12 and 4 × 3 = 12 to represent 3 fours or
4 threes.
Explain that the x sign is read as times. Have students use cubes to show what each number in the number sentence means. Emphasize that in a multiplication
problem, each group must have the same numbers. Explain that 3 × 4 = 12 and
4 × 3 = 12 are turn-around facts for multiplication, similar to
turn-around facts for addition (e.g., 3 + 4 = 7 and 4 + 3 = 7).
Continue to tell multiplication stories for students to
model with their connecting cubes (e.g., 5 monkeys
each eat 3 bananas;
3 frogs are in each of the
6 aquariums, etc.) Each time, ask students to explain
their number sentences and strategies for solving the
problem. Compare addition and multiplication number
sentences and have students explain each number
in the sentence.
Distinguish Between Addition and Multiplication
Situations. Conduct a discussion with students
about the differences between addition and multiplication
situations. Focus on the fact that there are
equal groups in multiplication.
Display the chart with the four problems you prepared prior to the lesson. See Materials Preparation.
Read Questions 1–2 on the chart.
- Sara has 4 pencils and Jason has 3 pens. How many writing tools are there altogether?
- There are 4 students. Each student has 3 pencils. How many writing tools are there altogether?
- Are the numbers the same in each problem? (yes)
- What is the question for each problem? (How many writing tools are there altogether?)
- How can you tell whether to add or multiply for each problem?
Demonstrate the differences between the problems by acting out each situation. Have 2 students come
to the front of the class. Give one student 4 pencils and the other student 3 pens.
- How many writing tools do they have altogether?
(7 writing tools)
- What number sentence represents this situation?
(4 + 3 = 7)
- What do the numbers 4 and 3 represent? What labels would you use? (4 pencils + 3 pens = 7 writing tools)
Next, ask 4 students to come to the front of the class. Give each student 3 pencils.
- How many writing tools do they have altogether? (12)
- What number sentence represents this situation?
(3 + 3 + 3 + 3 = 12)
- What is a multiplication number sentence for this problem? (4 × 3 = 12 or 3 × 4 = 12)
- How is this problem different from the first problem? (Possible response: For the first problem, there are 2 different numbers: 4 and 3. For the
second problem, each student has the same number: 3 pencils.)
- When can you use multiplication? (When you are adding the same number over and over you can use multiplication.)
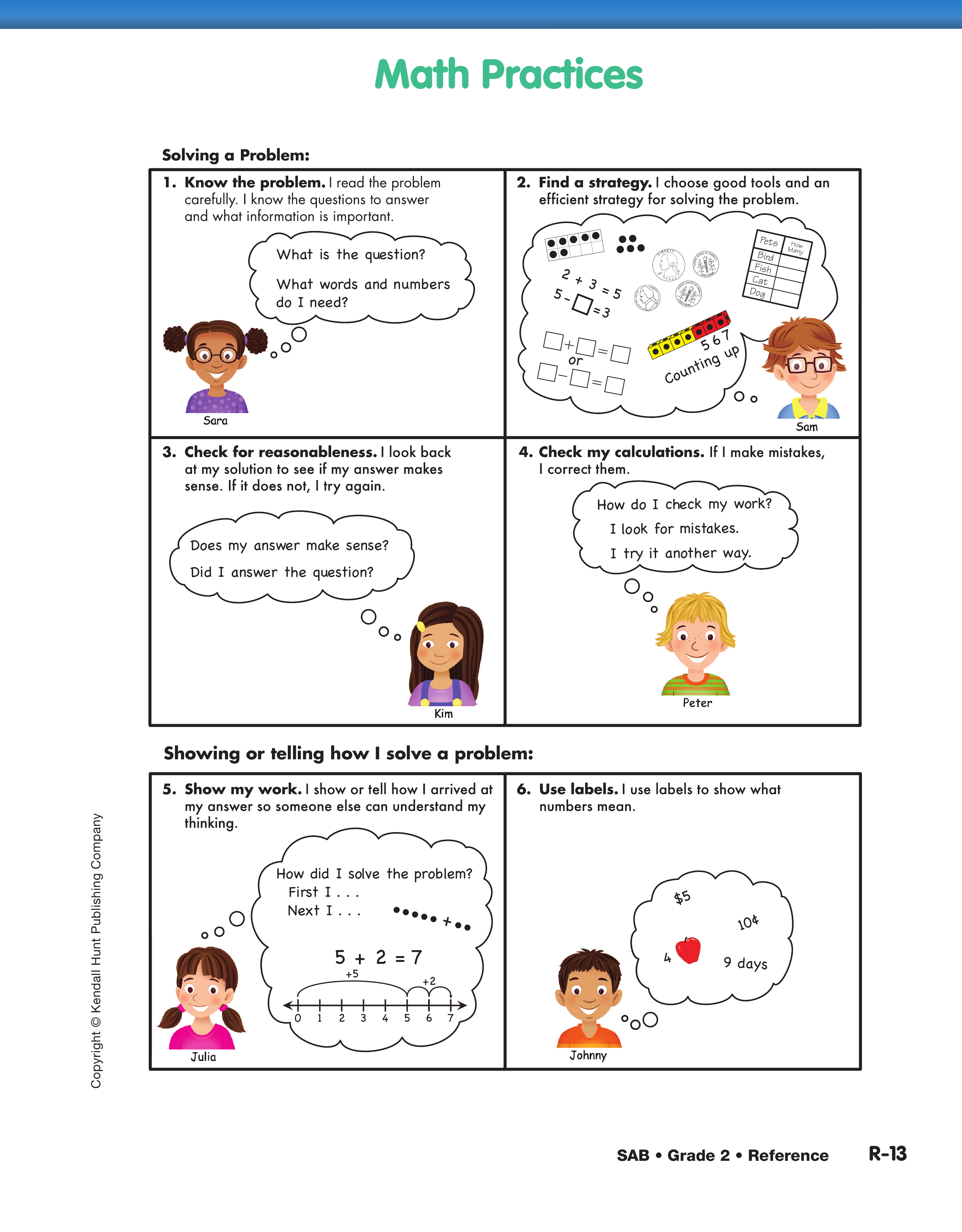
Display the Math Practices page in the Student Activity Book Reference section. Have students use
the Math Practices as a guide when solving problems. Emphasize MPE1, MPE2, MPE3, MPE5, and MPE6.
- For Math Practice 1, think about what the question is asking you to do and what information is important to solve the problem.
- For Math Practice 2, find an efficient strategy for solving the problem. Should you add or multiply the numbers in the problem?
- For Math Practice 3, check your solution for reasonableness. Does your answer make sense? Did you answer the question?
- For Math Practice 5, show or tell how you solved the problem. Write a number sentence.
- For Math Practice 6, use labels. The labels will help you see what the numbers in the problem mean.
Read Questions 3 and 4 to students:
- I have 3 blue crayons and 5 red crayons. How many crayons do I have altogether?
- I have 3 boxes. Each box has 5 crayons. How many crayons do I have altogether?
Ask student pairs to draw their solutions and write number sentences. Remind them to use the Math Practices to guide them as they solve the problems.
Upon completion, ask the following questions for each problem:
- What did you have to find? (the total number of crayons)
- What do the numbers 3 and 5 represent? What labels did you use? (Question 3: 3 blue crayons
and
5 red crayons; Question 4: 3 boxes with 5 crayons in each)
- What did you draw? (Question 3: 3 blue crayons and 5 red crayons; Question 4: 3 boxes with 5 in each)
- What is a number sentence that shows this problem?
(Question 3: 3 blue crayons + 5 red crayons = 8 crayons; Question 4: 5 + 5 + 5 = 15 or 3 × 5 = 15)
- Does your answer make sense? How do you
know? (Possible response for Question 3: Yes. If
you add 3 blue crayons and 5 red crayons, there
are 8 crayons altogether. Possible response for
Question 4: Yes, I knew there was the same number
in each of the 3 boxes so that makes 15 altogether.)
Discuss the difference between the two problems.
- Are the groups equal in Question 3? (No, they were different. One had 3 blue crayons and the other had 5 red crayons.)
- Are the groups equal in Question 4? (Yes, the boxes are the groups. Each group has 5 crayons.)
- For which problem did you write a multiplication sentence? (Question 4)
- For which problem did you write an addition problem? (Question 3)
- How would you describe what is different about these two problems? (Possible response:
Question 3 had groups that were not the same. Question 4 had groups that were all the same.)
Have students work independently on the problems on the Know the Problem pages in the Student Activity Book.
Encourage them to use the strategies
displayed on the Repeated Addition Strategies chart. Monitor students as they solve the problems to see if
they understand the difference between addition and multiplication situations.