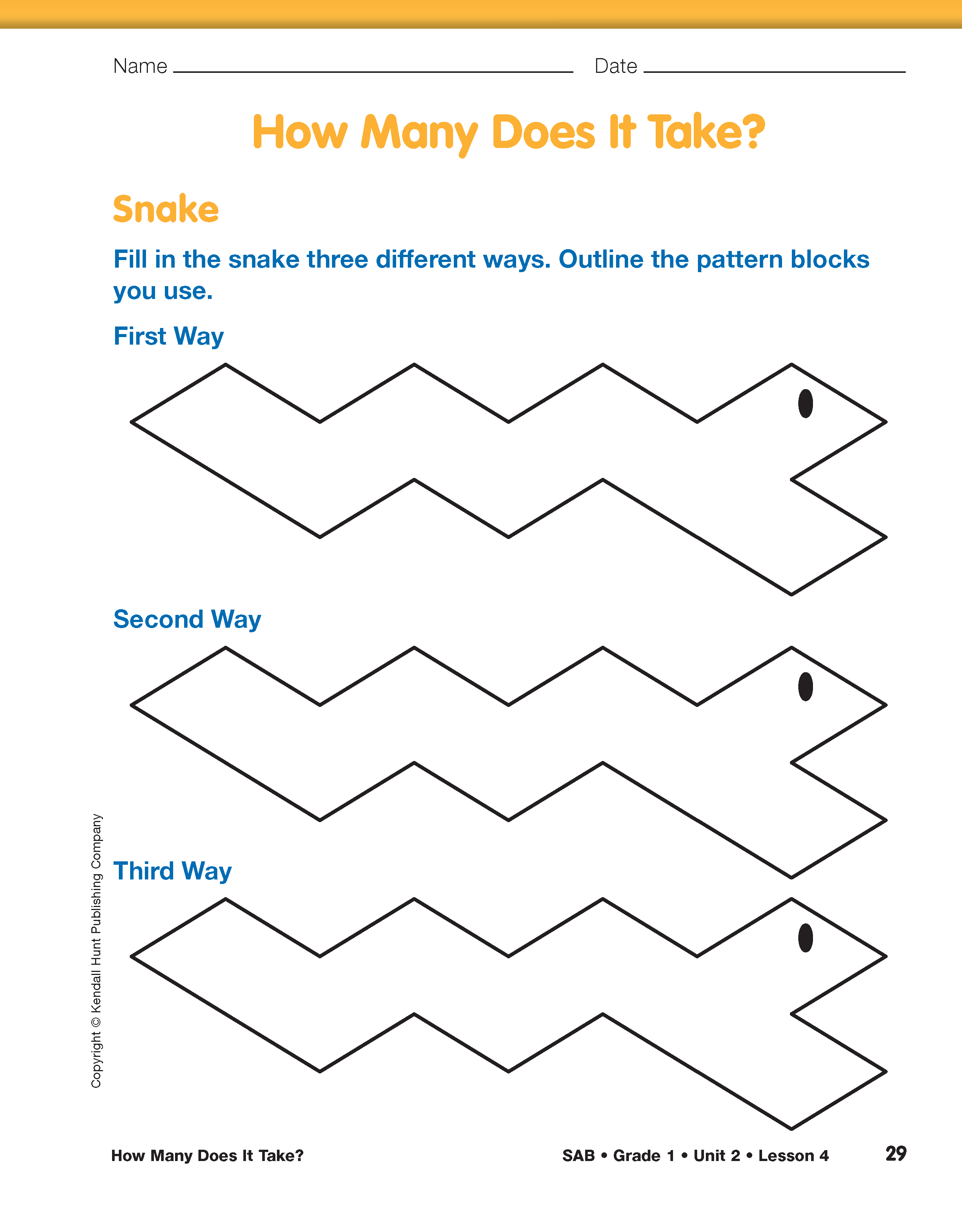
Use Pattern Blocks to Fill in
the Snake Outline. Ask students to remove the How Many
Does It Take? pages from their Student Activity Book.
Display the shape outlines in the Snake section of these pages
and an assortment of pattern blocks. Explain to students that
they are going to use pattern blocks to fill in the snake outline
and record their solutions. Remind students of their work in
Lesson 3 in which they found partitions of the hexagon pattern
block using other pattern blocks.
- In Lesson 3 we found many ways to make
hexagons. How did you find different ways to make the
hexagon? (Possible responses: trial and error or trying to
match sides on the hexagon with other blocks)
- How did you know which pattern blocks to use? (I
had to match sides and corners.)
- How can you use any of those ways to help you
find ways to make the snake? (Possible response: I just try
it out by matching sides on the blocks with the sides on the
snake. When I find one way I just switch other pattern blocks
that fit the same shape like when three triangles make one
trapezoid.)
- Let's start one. Where should I start? At the
tail? At the head? In the middle? Skip around? (I think it's
good to start at the head or the tail, but not skip around.)
- If I start with the head (or tail) which
block(s) should I try? (Possible responses: Try the blue
rhombus by the mouth. Try the red trapezoid for the tail.)
- Do you think you can use all the different
pattern blocks? (Answers may vary.)
- Which shape should I try first? (Possible
response: Try a green triangle at the tail.)
Continue by demonstrating with a few more pieces building
on the display. Ask students to find one solution and show it on
the first snake on the page.
As students work, take the opportunity to talk with them
about the shapes to probe their thinking.
Use prompts similar to the following:
- Name the shapes that you used to fill the
outline.
- How did you decide to use this [name shape]?
- How are these shapes alike? How are they
different?
- How many of this shape do you think it will
take to fill the outline?
- If you had to use as many different shapes as
possible, what shapes would you choose? Why?
- If you could use only one type of pattern block
to fill the outline, which would you choose? Why?
Record Snake Solutions. After
adequate time has been provided, discuss solutions.
- Can someone name some of the shapes you used?
- Did anyone else use any of these shapes?
- Did anyone use other shapes not mentioned?
Responses should include use of red trapezoids, green
triangles, and blue rhombuses. Blocks that cannot be used for the
snake (the yellow hexagon, orange square, and tan rhombus) will
be discussed later in the lesson. Display the data table from the
Snake Table section of the pages. Point out the three columns
where students will record the number of each block used for each
solution. Have students fill in the data for their first
solution.
Briefly discuss the first column of the data table
using prompts similar to the following:
- Look at your data table. Did anyone write a
number for every shape? (No.) What does that mean? (It means
some shapes might not work.)
Discuss the different combinations of blocks
students used to fill the snake outline.
- If you used just one kind of block, what kind
did you use? (Possible responses: green triangles or blue
rhombuses.)
- If you used two kinds of blocks, what kinds did
you use? (Possible responses: green triangles and blue
rhombuses, or blue rhombuses and red trapezoids, or green
triangles and blue rhombuses.)
- Did anyone use fewer than five blocks? Or more
than ten blocks?
Demonstrate how to record the solution by starting at one
end of the snake, removing one block, and tracing the exposed
edge of the next block. Once all the blocks are traced, count the
different shapes to verify the information recorded in the table.
Ask students to repeat this process to find two other ways
to fill the snake with pattern blocks. See Meeting Individual
Needs.
- To facilitate success with tracing the blocks, have
students fill in the shape, then complete the table, then trace
the solution before going on to the next solution. This will
reduce the number of blocks on the page at one time, preventing
accidental displacement of blocks that may otherwise be in the
way.
- Another method is to have students find a solution then
complete the data table, repeating this process until all three
solutions are found and displayed. Students may then select at
least one solution to trace rather than all three.
- A third method would be to take a photograph of
students' solutions.
As students work, circulate throughout the room.
- How is your second way different from your
first way?
- If you had to use fewer blocks, what might you
do? (Possible response: Trade some of the smaller blocks for
larger blocks. For example, 3 triangles for one trapezoid.)
- If you had to use more blocks, what might you
do? (Possible response: Trade some of the larger blocks for
smaller blocks. For example, 1 blue rhombus for 2 triangles.)