The equal sign. The equal sign signifies a relation between two numbers. Understanding that the equal sign means that expressions on the two sides of the equal sign represent the same numerical value is an important mathematical concept. Experiences that allow students to explore these ideas now will help them avoid common misconceptions later. For example, students who only see number sentences of the form 3 + 2 = 5 often come to the erroneous conclusion that the equal sign means, “The answer comes next” or “Do the operation.”
Students need many experiences using the equal sign flexibly. Early experiences include seeing problems as both 5 + 6 =  and
and  = 5 + 6. Other experiences can come in the form of identifying whether a number sentence is true or false or finding the missing number in an open number sentence (5 + 6 = 6 +
= 5 + 6. Other experiences can come in the form of identifying whether a number sentence is true or false or finding the missing number in an open number sentence (5 + 6 = 6 +  ). At first, students may need to find the value of the expression on each side of the equal sign to solve these types of problems. With experience, students will develop the capability of reasoning such as, “I know that 9 + 4 = 10 + 3 because I take one from the four to make ten and I have three more to add.” Using the equal sign flexibly will allow students to represent their thinking more easily. For example, students can say that they know that 19 + 4 is 23 because 19 + 4 = 20 + 3.
). At first, students may need to find the value of the expression on each side of the equal sign to solve these types of problems. With experience, students will develop the capability of reasoning such as, “I know that 9 + 4 = 10 + 3 because I take one from the four to make ten and I have three more to add.” Using the equal sign flexibly will allow students to represent their thinking more easily. For example, students can say that they know that 19 + 4 is 23 because 19 + 4 = 20 + 3.
What to avoid. Researchers caution against using the equal sign in ways in which it does not represent a relation between numbers. For example, avoid expressions such as “Jerome = 3 feet” or “Sara = 4.” Similarly, it is not appropriate to draw a picture of three objects and then write “

 = 3”. Save the equal sign for representing relations between numbers.
= 3”. Save the equal sign for representing relations between numbers.
It is also important to avoid writing number sentences that chain calculations together incorrectly. For example, to show a partitioning strategy for adding 10 + 17 = 27, avoid writing 10 + 10 = 20 + 7 = 27, since 10 + 10 does not equal 20 + 7. (Carpenter, et al., 2003; NCTM, 2000)
For more information, see the Background of this unit.
Use Connecting Cubes. Review the different pairs of addends that make a ten: 1 + 9, 11 + 9, 9 + 21, 8 + 2, 8 + 12, 32 + 8, 3 + 7, 7 + 23, 63 + 7, and so on. Use this as an opportunity to discuss patterns and turn-around facts. For example, 2 + 8 and 8 + 2 both equal 10 and are called turn-around facts.
Give each pair of students twenty blue and twenty red connecting cubes. Ask students to build a train with seven blue cubes and another train with eight red cubes.
- What can you do with the cubes to make a train of ten? (Split the 7 train into a train of 2 cubes and 5 cubes and move 2 blue cubes to the train of eight. Or split the train of 8 cubes into two trains of 3 and 5 cubes and move 3 red cubes to the train of seven.)
- You now have a train of ten cubes and a train of five cubes. What is the sum? (15)
- Why is this problem (10 + 5) easier to solve than the first one (7 + 8)? (Possible response: 10 + 5 = 15 is easy to add because adding any number to ten is just the one digit for the ten and then the number.)
- Does someone have another mental math strategy for solving 7 + 8? (Possible response: I think of doubles. 7 + 7 = 14 and 1 more is 15.)
Ask students to build a train with 17 blue cubes and another train with 8 red cubes.
- What can you do with the cubes to make a train of 20? (Move three red cubes from the train of 8 to the train of 17.)
- What trains do you have now? What number sentence will match the total number of cubes in the two trains now? (I have a train of 20 and a train of 5. 20 + 5 = 25)
- Is 20 + 5 easier to solve than 17 + 8? Why or why not? (Possible response: It is easier to add a number to a ten. It is easier to solve 20 + 5 than 17 + 8 because you do not have to regroup the ones into another ten as you do when adding 7 + 8.)
- Is there another mental math strategy that you can use to solve 17 + 8? (Possible response: Another strategy could be to break apart 17 into 10 + 7. Add 7 + 8 to make 15, and then add the 10 to make 25.)
At this point in the lesson, pause to make sure students are able to model the addition problems with connecting cubes and provide assistance to those who are having difficulty. Repeat the discussion above for the following pairs of addition problems:
6 + 8 and 16 + 8
8 + 5 and 18 + 5
Ask students to re-count the numbers of blue and red cubes after they have made a ten so they can see that the original quantities have moved but have not changed.
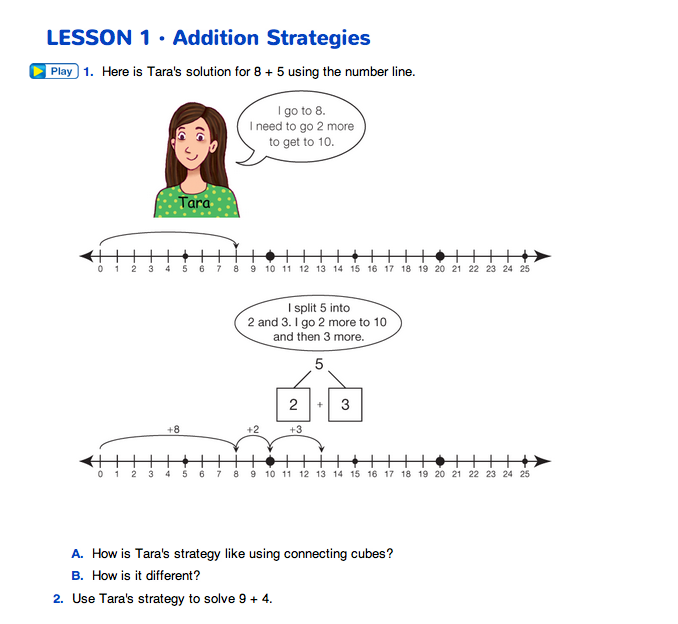
Use the Number Line. Ask students to read and discuss Tara's strategy for solving 8 + 5 using Question 1 on the Addition Strategies page in the Student Guide.
- Compare the making-tens strategy on the number line to using cubes (Questions 1A and B). (Possible student response: We both made tens. We made a ten with the cubes by splitting the 5 train into 2 cubes and 3 cubes and putting the 2 cubes onto the 8 train. Then we had a 10 train and a 3 train. Tara made ten by hopping to 8 then 2 more to get to 10. Then she had 3 more to go to get to 13. They are different because the numbers are on the number line and can help you know how far to go.)
Students can use the Number Lines 0–30 page in the Student Activity Book or their desk number lines to solve Question 2. Ask one student to demonstrate on the class number line. Students will use the Number Lines 0–30 page for homework, too.
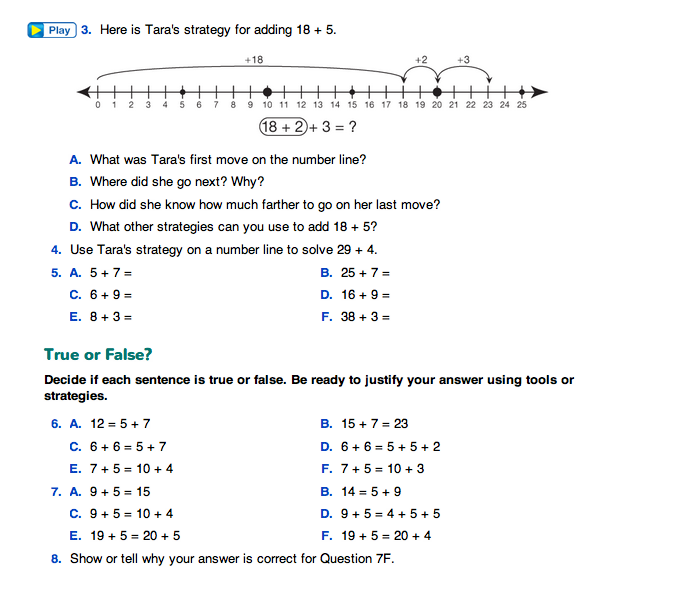
Ask students to discuss Questions 3 and 4 in pairs and be ready to explain their thinking and strategies to the class using the class number line. Question 5 provides practice with addition strategies.