Use Addition Strategies. Students can continue their exploration of magic squares by completing the Discuss and the Use Strategies and Patterns sections in the Student Guide. Use Questions 1–2 to review the information about magic squares from the story, Yu the Great: A Chinese Legend.
Questions 1 and 2 involve the magic square from the story. Question 2 asks students to find the sum of each row, column, and main diagonal of the magic square on the turtle's back. See Figure 1.
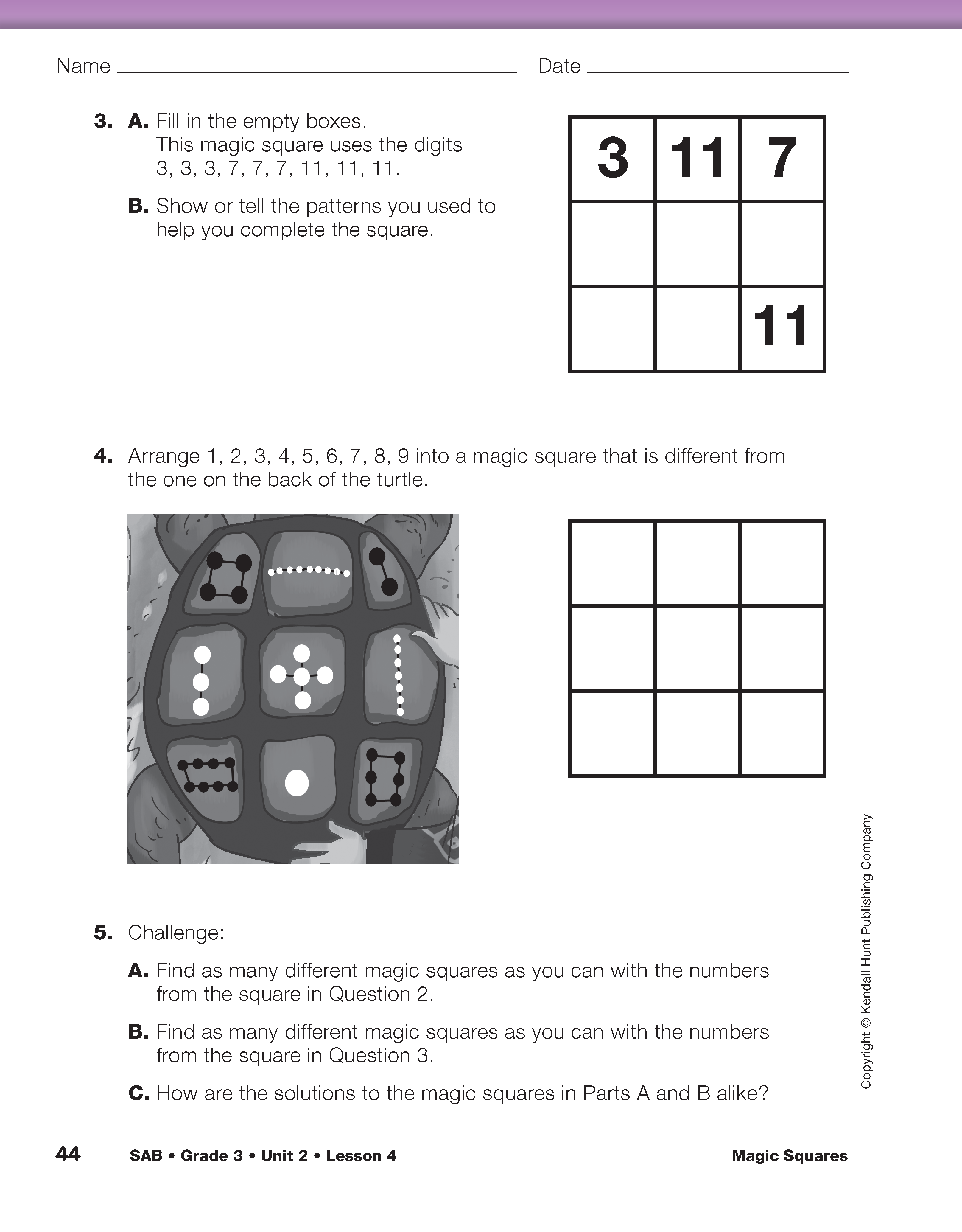
Questions 3–5 ask students to verify and identify magic squares by adding the rows, columns, and diagonals. These problems lend themselves to the strategies discussed in Lessons 1 and 2. Students can complete the questions independently, with a partner, or in a small group before discussing them with the whole class. The following prompts will guide a discussion about the strategies and patterns students used.
- In Question 3, what is sum of each row, column, and diagonal? (The sum of each is 21.)
- What strategies did you use to find the sum of each row, column, and diagonal? (Two possible responses: I used the making-tens strategy to add the column 6 + 11 + 4. First I added 6 + 4 because I know that equals 10. Then I added 10 + 11 to get the answer 21. Or, I used doubles to solve 10 + 3 + 8. First I broke 3 into 2 + 1. Then I added 2 + 8 because I know that is 10. Now I have 10 + 10 + 1. I know that when you double 10 the answer is 20, and 20 + 1 = 21, so the answer is 21.)
- Yu discovered that Luo Shu, the design on the turtle's back, had several patterns. What patterns do you see in the magic square in Question 3? (Some of the patterns that students may discover include: The number 7 is the middle number in the list of numbers used and is also in the middle square. The numbers in one of the diagonals are in order. In this magic square, there are five numbers that are odd and four that are even. The even numbers are in the corners and the odd numbers are in the middle spaces.)
- In Question 4, what strategy did you use to find out which one was the magic square? (I added up the rows, columns, and diagonals in each square. In the first square, all of the rows, columns, and diagonals added up to 30, so it is the magic square. In the second square, I added the first column and got 6 + 3 + 4 = 13. Then I added the second column and got 1 + 5 + 9 = 15, so I knew right away it was not a magic square.)
- Look at the magic square in Question 4A. What strategies did you use to find the sum of each row, column, and diagonal? (Two possible responses: When I added 13 + 6 + 11, first I changed the 11 to 7 + 4. I added 13 + 7 = 20. I knew that I could make a ten from the 6 and the 4, so then I could add 20 + 10 = 30. I used a double to add 11 + 12 + 7. First I changed the 12 into 11 + 1. I doubled the 11 to make 22. Then I added 22 + 1 + 7. I knew 7 + 1 = 8 and 8 + 22 = 30, so the answer is 30.)
- What patterns do you see in the magic square in Question 4A? (The list of numbers used goes from 6 to 14. The middle number in the list is 10, and it is in the middle square. There are five even numbers and four odd numbers in the list, so the odd numbers are in the corners and the even numbers are in the middle squares. One of the diagonals has the numbers 9, 10, and 11 in order.)
Question 5 shows another type of magic square. In this square, while all the numbers are odd, the square is still balanced and has many patterns. Use the discussion prompts to help students apply what they have already learned to this new square.
- What is the sum of each row, column, and diagonal in this magic square? (The sum is 15 in each.)
- How is this magic square different from the others we have done? (All of the numbers in this magic square are odd. You use only three numbers in this square instead of nine different numbers.)
- How is this magic square similar to the others we have done? (Responses should include: Even though the numbers are all odd, the numbers on the diagonal are in order: 3, 5, 7. The middle number, 5, is still in the center square.)
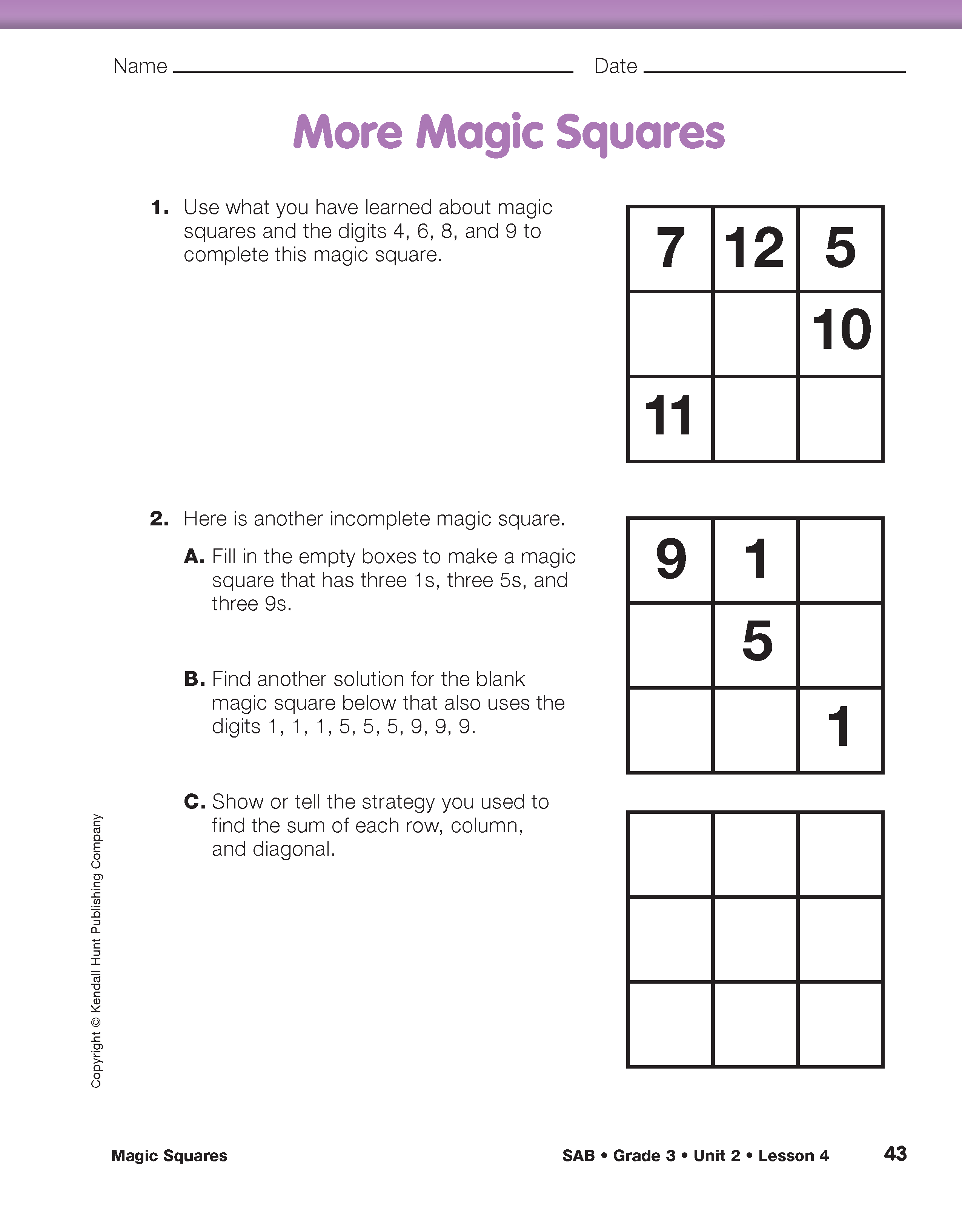
Use Patterns to Solve Magic Squares. Direct students to the More Magic Squares pages in the Student Activity Book.
Before students begin their work on these pages ask:
- What have we learned about magic squares that might help you as you work on these questions? (The sums of all the rows, columns, and diagonals have to be the same. The middle number of the list of numbers used is in the middle square. If there are five even numbers in sequence, the middle number and all the numbers in the middle squares will be even and the corners will be odd numbers. If there are five odd numbers in the sequence of numbers, the middle number and all the numbers in the middle spaces will be odd and the corners will be even. Sometimes the numbers could be all odd or all even but they still follow the patterns.)
Direct students to work with a partner or in a small group to complete Questions 1–5. As they work, encourage them to use the patterns they have learned to complete each square.
The blank magic square in Question 4 of the More Magic Squares page in the Student Activity Book provides students the opportunity to apply the patterns they discovered through their previous work. If a student continues to have difficulty with this problem after reviewing the patterns, you may want to provide the center number for the square.
Question 5 provides additional practice with blank magic squares. Students begin with squares they have already completed but try to find additional solution paths. Question 5C asks students to use the patterns they have discovered to compare their solutions.