Lesson 3

Strategies to Find Volume

Est. Class Sessions: 2

Developing the Lesson

Part 1: Cube Models with All Cubes Visible

Find Strategies for Tall Tower. Students work with a partner to complete this lesson. Each student pair will need
60 connecting cubes.

X

Multiplicative Reasoning. Repeated addition is one of the most familiar structures of multiplication. As students experience the need to add a number repeatedly, they develop a sense of what is happening mathematically in what they will eventually know as multiplication. As they work a problem such as 5 + 5 + 5 + 5, they begin to understand it as a group of 5 added four times. With experience, they become comfortable thinking of four 5s and eventually 5 four times. Later, when they are formally introduced to multiplication and its terminology, students already will have a strong base for understanding the meaning of the multiplication operation. This conceptual underpinning is essential for acquisition of fluency with the basic multiplication facts as well as with computation with two-digit numbers.

Display the TIMS Towers 1 Master and ask:

X
  • What do you notice about the two buildings pictured on this display? (Possible response: One building is skinny and tall and one is shorter and wide. Both buildings are shaped like rectangles.)
  • What do you notice about the number of cubes used for the floors in each building? (Possible response: One building has two cubes on each floor and the other has 6 cubes on each floor.)
  • If you were going to build these towers with your cubes, what are some things that you would need to know before you begin? (Possible response: You need to know how many cubes are on each floor of the building and how many floors in all.)

Direct students to the TIMS Towers pages in the Student Activity Book. Ask students to work with their partner to complete Questions 1–3. For Question 1, students are asked to build a cube model of the Tall Tower and to record the floors, number of cubes on each floor, and volume for this building. Ask students to think about ways they can group the cubes and use addition strategies to find the volume rather than counting each cube by ones.

As students work, use these or similar discussion prompts to guide them:

X
  • How can you use the drawing to make sure your model is accurate? (Possible response: Since you can see all the cubes in the buildings, you can compare your building to the drawing to make sure it has the same number of floors and the correct number of cubes on each floor.)
  • What label do you need to use for the floors in this building? (floors or units)
  • What label do you need to use for the number of cubes in each floor? (cubes)
  • What label do you need to use for the volume for the Tall Tower? (cubic units)

After most students have finished Questions 1–3, display the Tall Tower Volume chart created before the lesson. See Materials Preparation.

Use this chart to record student responses to the following prompts:

X
  • What is the volume of the Tall Tower? (22 cubic units)
  • What strategy did you use to group the cubes and use repeated addition to find the volume for the Tall Tower?

Encourage students to share their solution paths. As students share ways the cubes can be grouped and counted, write the different ideas on the chart. Some possible strategies include:

  • Grouping by floors and skip counting by twos from two to twenty-two.
  • Grouping by columns and doubling eleven.
  • Grouping by columns by doubling ten and counting on two more.
Ask students to share the number sentence they used to represent their strategy and add them to the Tall Tower Volume chart. Figure 2 shows a possible completed chart for the Tall Tower.

X

Writing Number Sentences. The equal sign in a number sentence shows that both sides are the same or equivalent. Be careful to not string together quantities that are not equal as number sentences are used to show solutions. For example, 6 + 6 + 6 = 18 + 18 = 36 is not a true statement. Rather record 6 + 6 + 6 = 18 and 18 + 18 = 36.

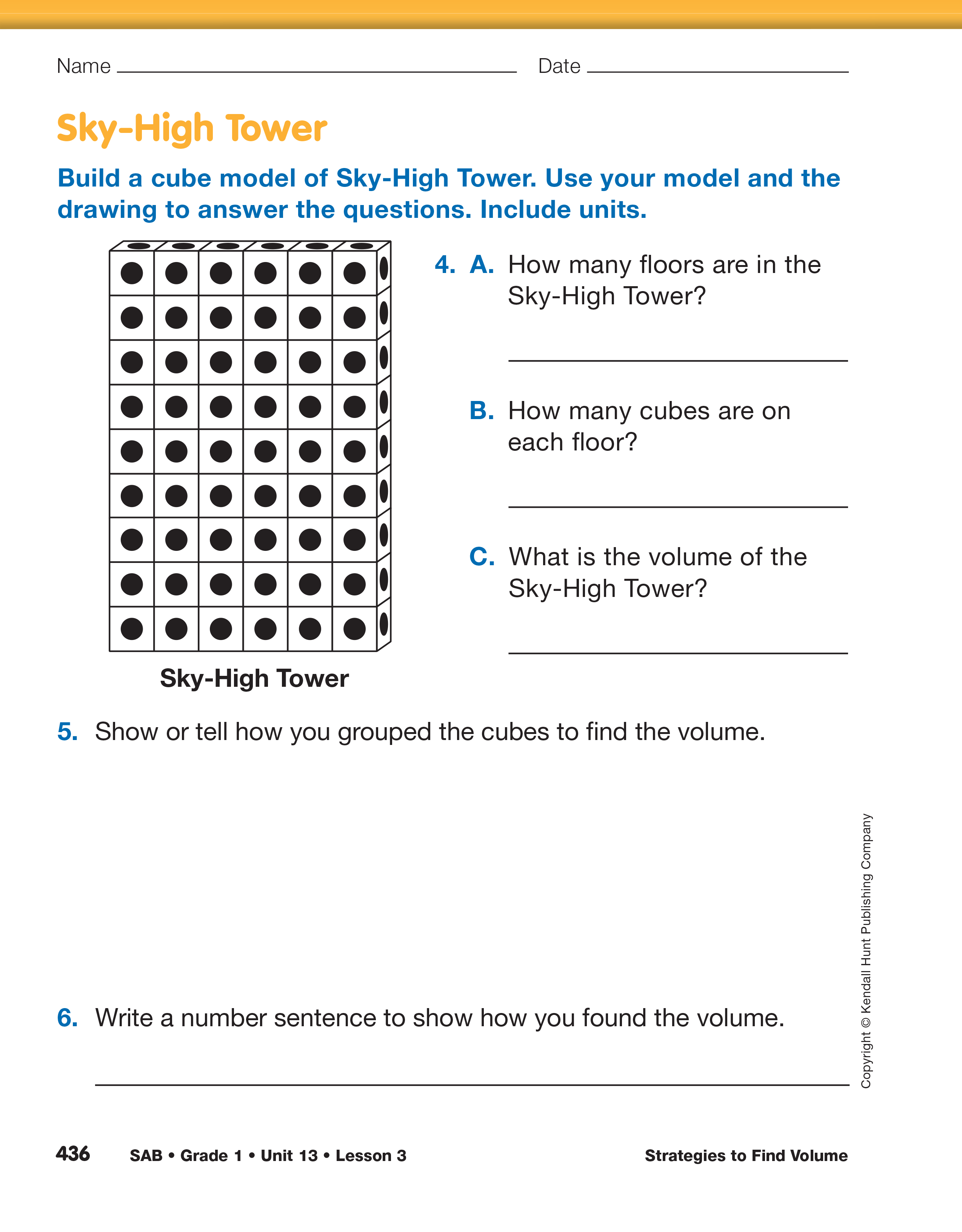
Find Strategy for Sky-High Tower. Direct students to the Sky-High Tower section on the TIMS Towers pages. Students work with their partner to complete Questions 4–6. To complete these questions, students replicate the work they did to find the volume of the Tall Tower for Sky-High Tower. Display the Sky-High Tower Volume chart. Ask students to share the strategies and number sentences they used to find the volume of Sky-High Tower as you record them on the chart. Since they have never skip counted by either 6s or 9s, students may want to count the cubes in this tower by ones.

Encourage them to look for and share more efficient ways to count, such as partitioning each row of 6 into 5 + 1. They can then skip count by fives and count on by ones (5, 10, 15, 20, 25, 30, 35, 40, 45, 46, 47, 48, 49, 50, 51, 52, 53, 54). Students can also use their 100 Chart in the Reference section of the Student Activity Book. Students can think of each column of nine in the Sky-High Tower as 10 – 1. They can then use the 100 Chart to count by 10s and then count back 6 (10, 20, 30, 40, 50, 60, 59, 58, 57, 56, 55, 54). Continue to share strategies until someone suggests using the calculator to repeatedly add 6 or 9. Ask all students to work with their partner and use their calculator to find the volume of Sky-High Tower. One student should key in the number 6 or 9, while the other keeps track of how many times it is added. Students can then change roles and use the calculator to find the volume using the other number.

Assign the Two Towers Homework Master.

Adventure Book:
Student Activity Book: 435 436
Answers
X
SG_Mini
+
X
SG_Mini
+
Master:
A possible completed chart for the Tall Tower
X
+