Multidigit Addition and Subtraction
DEVELOPING COMPUTATION METHODS

Build on Invention and Understanding
In this unit, students explore several methods for adding and subtracting multidigit … read more
Build on Invention and Understanding
In this
unit, students explore several methods for adding and
subtracting multidigit numbers by building on the
strategies and representations already developed for
place value. Students use some of these representations
to support their mental math strategies and to
connect to other methods. The goal is for students to
be proficient with multiple methods, so they can analyze
the efficiency and usefulness of the methods in
different situations (NCTM, 2000, p. 153).
Eventually students will be able to select appropriately
among mental math, estimation, and
The development of computation methods in Math Trailblazers is based on the research of others in the field of mathematics education and our research in Math Trailblazers classrooms. Research by others corroborates the relationship between conceptual understanding and procedural fluency (Fuson & Burghardt, 2003; Fuson, 2003; Verschaffel, et al., 2007; National Research Council, 2001; NCTM, 2000; Hiebert & Wearne, 1996). For this reason, students using Math Trailblazers first develop their own methods for each operation. With these opportunities, students make connections between their understanding of place value and procedures for computation. As stated in Adding It Up: Helping Children Learn Mathematics, "Opportunities to construct their own procedures provide students with opportunities to make connections between the strands of proficiency. Procedural fluency is built directly on their understanding. The invention itself is a kind of problem solving, and they must use reasoning to justify their invented procedure. Students who have invented their own correct procedures also approach mathematics with confidence rather than fear and hesitation" (Kamii and Dominick, 1998, quoted in National Research Council, 2001, p. 197).
Use Representations
Conceptual understanding supports procedural fluency with standard procedures and algorithms as … read more
Use Representations
Conceptual understanding supports procedural fluency with standard procedures and algorithms as well. Therefore, students learn to represent operations with base-ten pieces, number lines, or pictorial models. For the representations to be effective, students need sufficient opportunity and time to make connections between the models and numerical procedures. In summarizing findings of several researchers, the National Research Council reported, "Research indicates that students' experiences using physical models to represent hundreds, tens, and ones can be effective if the materials help them think about how to combine quantities and, eventually, how these processes connect with written procedures. The models, however, are not automatically meaningful for students; the meaning must be constructed as they work with the materials. Given time to develop meaning for a model and connect it with the written procedure, students have shown high levels of performance using the written procedure and the ability to give good explanations for how they got their answers" (National Research Council, 2001).
Throughout the unit, students share their own strategies with their classmates and read about possible strategies in the student materials. Comparing the various strategies and trying them out allows students to analyze the efficiency and usefulness of the methods in different situations (NCTM, 2000, p. 153).
Support Use of Multiple Strategies
Research in Math Trailblazers classrooms using previous editions indicates that students need … read more
Support Use of Multiple Strategies
Research in Math Trailblazers classrooms using previous editions indicates that students need support developing and using multiple computation strategies, instead of relying heavily on one procedure. Therefore, we have developed strategy menus for each operation to help students select appropriate methods for solving problems based on the context of the problem or the numbers involved. See Figure 1 for a menu of subtraction strategies from Lessons 4 and 5.
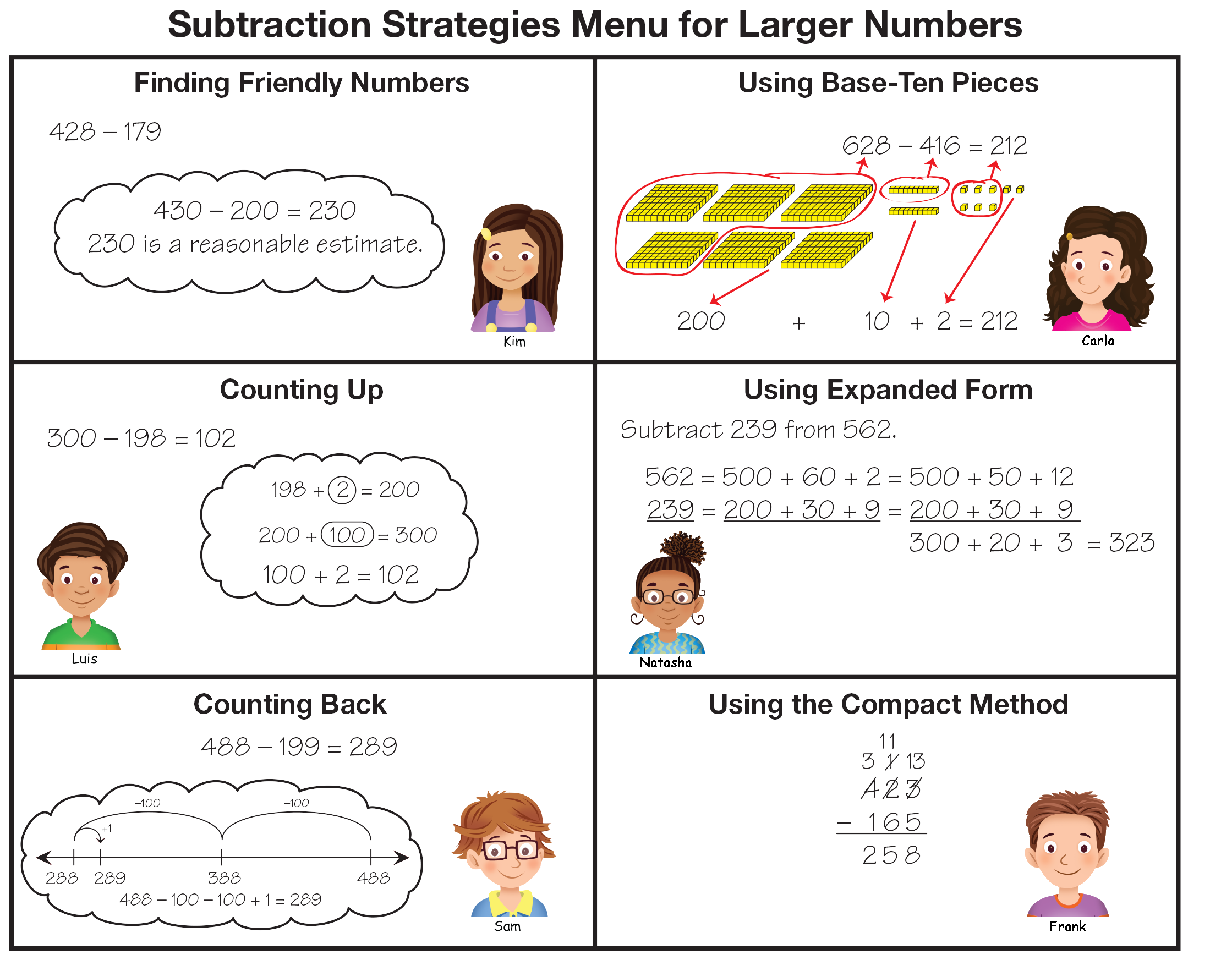
Figure 1: Subtraction methods students explore in this unit
Multiple Strategies to Support Conceptual Development
Students collect, compare, and then sort the variety of strategies they have invented and … read more
Multiple Strategies to Support Conceptual Development
Students collect, compare, and then sort the variety of strategies they have invented and experienced into a menu. The menus remind students of strategies and methods they are developing and help students make connections among the strategies. They choose strategies that best support their conceptual development. For example, students may find using expanded form similar to but more efficient than using base-ten pieces. See Figure 2.
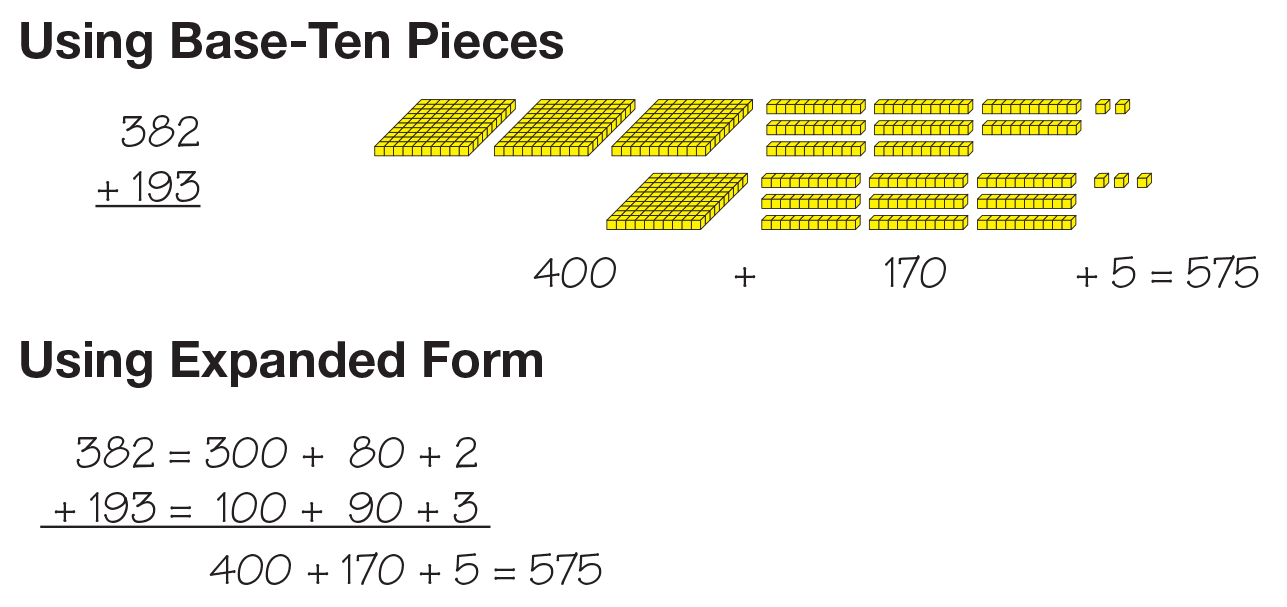
Figure 2: Connect adding with base-ten pieces and using expanded form
Choosing Appropriate Strategies
These menus will eventually help students choose appropriate methods for calculating based on the … read more
Choosing Appropriate Strategies
These menus will eventually help students choose appropriate methods for calculating based on the numbers in the problems. For example, students will arrive at accurate solutions more often if they have the flexibility to solve the addition problem 139 + 41 using a mental math strategy, than if they use the compact algorithm to regroup. On the other hand, it is more appropriate to use the algorithm to solve 9524 + 3679.
Checking for Reasonableness
Students also use the menus to check their work for accuracy by choosing a second method to solve a … read more
Checking for Reasonableness
Students also use the menus to check their work for accuracy by choosing a second method to solve a problem and comparing the two answers. To solve the problem in Figure 3 efficiently, students need to develop the ability to identify it as an estimation problem and then solve it mentally using convenient numbers.

Figure 3: Which two of the items above would provide a total of about 600 calories?
Mental Math Strategies
Development of mental math strategies is an important outcome of students' study of whole numbers for … read more
Mental Math Strategies
Development of mental math strategies is an important outcome of students' study of whole numbers for two reasons. First, use of these strategies helps students develop number sense and flexibility with numbers. Secondly, using mental math is often the most efficient means of solving problems, either for finding exact answers or for finding estimates when appropriate. Therefore, students need to have many opportunities to choose an efficient strategy for a given problem based on the numbers in the problems and the real-world context.
Students should have opportunities to develop strategies that make sense to them. Some strategies may include some mental calculations along with a few written notes. Representations may also support students as they develop these mental math strategies (e.g., coins, number lines, base-ten pieces). For example, to solve 345 + 205, students may visualize the number line but keep track of the hops by writing down numbers: 345 + 200 is 545 and 545 + 5 = 550.
Discussing their strategies and explaining them in writing will help students clarify their own thinking and provide new ideas for others. However, it is important that students have opportunities to practice strategies that emphasize their efficiency. That is, when they choose to solve a problem mentally, they should not have to explain their thinking every time. For these reasons, students will see different types of directions for solving mental math problems in the unit. In some cases they are asked to explain their thinking in writing. At other times they are asked to share their thinking with a partner in discussion or to choose one problem out of a set of problems to show their reasoning in writing.
Estimation Strategies
Estimation is found throughout the Math Trailblazer curriculum. In this unit, students discuss one tool … read more
Estimation Strategies
Estimation is found throughout the Math Trailblazer curriculum. In this unit, students discuss one tool that is frequently used when estimating the result of a computation—finding a "friendly" or "convenient" number that is close to a given number. For example, find the sum of 57 and 74 by thinking that 57 is close to 60 and 74 is close to 75, so the sum is close to 60 + 75. Students first work with base-ten pieces to develop their sense of numbers in relation to "friendly" numbers like multiples of 10 and 100. Estimation is discussed as a way of finding reasonable, close answers and a way of predicting or checking answers to computation problems. We introduce these ideas before students work on paper-and-pencil methods for exact answers so that they are encouraged to use mental strategies for estimates.
Paper-and-Pencil Strategies
In this varied collection
of strategies, three are considered
Paper-and-Pencil Strategies
In this varied collection of strategies, three are considered paper-and-pencil methods: expanded form, all-partials, and compact. All three algorithms are based on the organization of the base-ten number system. There are many opportunities for students to identify connections between these strategies. The compact method is the method traditionally taught in the United States. However, it is not the only efficient method and is sometimes not the most efficient.
In fact, the all-partials method is a very transparent method so it is easy to find errors. It is a method that helps support and develop number sense and therefore estimation strategies. The expanded form method helps students clearly keep track of the different partitions of numbers needed to solve a problem.
As students learn efficient paper-and-pencil algorithms, they should be encouraged to revisit base-ten pieces as necessary to establish the connection between the symbolic procedures and the concrete representation. While work with base-ten pieces should inspire work with symbols, the two processes are not identical in students' minds. One major difference between using the pieces and the algorithm is that with the pencil-and-paper approach, the original problem remains on the paper in some form, while with pieces, the original problem is not evident once the solution is found. It takes time to make connections between base-ten pieces and algorithms, and you will need to help students make the connections.
Because the link between the manipulative work and paper-and-pencil solutions is not always clear to students, some educators suggest that it is confusing to try to slavishly mimic manipulative work in a symbolic way, step-by-step, i.e., doing the two procedures in parallel. Rather, they recommend first working the problem with pieces and then working the problem symbolically. Parallels between the two methods can then be made.
MATH FACTS and MENTAL MATH

Subtraction Facts
Daily Practice and Problems items in this unit can be used to assess students' fluency with the … read more
Subtraction Facts
Daily Practice and Problems items in this unit can be used to assess students' fluency with the subtraction facts related to the addition facts in Group F (14 − 4, 14 − 6, 14 − 8, 14 − 10, 15 − 5, 15 − 6, 15 − 9, 15 − 10, 16 − 6, 16 − 7, 16 − 9, 16 − 10, 17 − 7, 17 − 8, 17 − 9, 17 − 10, 18 − 8, 18 − 9, 18 − 10). Counting-on, counting-up, and counting-back strategies are most commonly used to solve these facts.
ALGEBRA in the EARLY GRADES

"A fundamental goal of all our algebraic thinking work focuses on helping teachers … read more
"A fundamental goal of all our algebraic thinking work focuses on helping teachers understand and engage their students in relational thinking. By relational thinking we mean examining expressions and equations in their entirety rather than as a process to be carried out step by step. Doing so requires using fundamental properties of number and operation to transform the mathematical expression … Ideas of relational thinking fit well into the arithmetic curriculum because they often make computation easier and provide an opportunity to make explicit much of what students are doing when they operate on numbers using standard algorithms. We see this when students add multidigit numbers and use an invented algorithm, for instance, on the problem 25 + 37 a child may respond, "I know that 20 and 30 is 50. Then I added the 5 and the 7. That's like 5 and 5 is 10, and 2 more. So that's one more 10, so 60 and 2 is 62" (Franke, Carpenter, and Battey, 2008).
In this unit, students focus on just this type of relational thinking as they develop mental math strategies and multiple methods to add and subtract multidigit numbers.
Resources
- Franke, M., T. Carpenter, and D. Battey. "Content Matters: Algebraic Reasoning in Teacher Professional Development." In Algebra in the Early Years. James J. Kaput, D.W. Carraher, and M.L. Blanton, eds. Lawrence Erlbaum Associates, New York, NY, 2008.
- Fuson, K.C. "Developing Mathematical Power in Whole Number Operations." In A Research Companion to Principles and Standards for School Mathematics. J. Kilpatrick, W.G. Martin, and D. Schifter, eds. National Council of Teachers of Mathematics, Reston, VA, 2003.
- Fuson, K.C., and B.H. Burghardt. "Multidigit Addition and Subtraction Methods Invented in Small Groups and Teacher Support of Problem Solving and Reflection." In The Development of Arithmetic Concepts and Skills: Constructing Adaptive Expertise. A.J. Baroody and A. Dowker, eds. Lawrence Erlbaum Associates, Mahwah, NJ, 2003.
- Hiebert, J., and D. Wearne. "Instruction, Understanding, and Skill in Multidigit Addition and Subtraction." Cognition and Instruction, 14 (3), pp. 251–283, 1996.
- Hiebert, J. "Relationships between Research and the NCTM Standards." Journal for Research in
Mathematics Education, 30 (1),
pp. 3–19 , 1999. - Hiebert, J., and D. Wearne. "Instruction, Understanding, and Skill in Multidigit Addition and Subtraction."
Cognition and Instruction, 14 (3),
pp. 251–283 , 1996. - Kamii, C., and A. Dominick, "The Harmful Effects of Algorithms in Grades 1–4." In The Teaching and Learning of Algorithms in School Mathematics L.J. Morrow and M.J.
Kenney, eds. (1998 Yearbook of the National Council of Teachers of Mathematics,
pp. 130–140 ). Reston, VA, 1998. - National Research Council. "Developing Proficiency with Whole Numbers." In Adding It Up: Helping
Children Learn Mathematics.
J. Kilpatrick , J. Swafford, and B. Findell, eds. National Academy Press, Washington, DC, 2001. - National Research Council. "Teaching for Mathematical Proficiency." In Adding It Up: Helping
Children Learn Mathematics.
J. Kilpatrick , J. Swafford, and B. Findell, eds. National Academy Press, Washington, DC, 2001. - Principles and Standards for School Mathematics. The National Council of Teachers of Mathematics, Reston, VA, 2000.
- Verschaffel, L.B. Greer, and E. De Corte. "Whole Number Concepts and Operations." In Second Handbook of Research on Mathematics Teaching and Learning. F.K. Lester, Jr., ed. Information Age Publishing Inc., Charlotte, NC, 2007.
- Wearne, Diana, and James Hiebert. "Place Value and Addition and Subtraction," Arithmetic Teacher, 41 (5), pp. 272–274, 1994.












