Find Area with Pennies

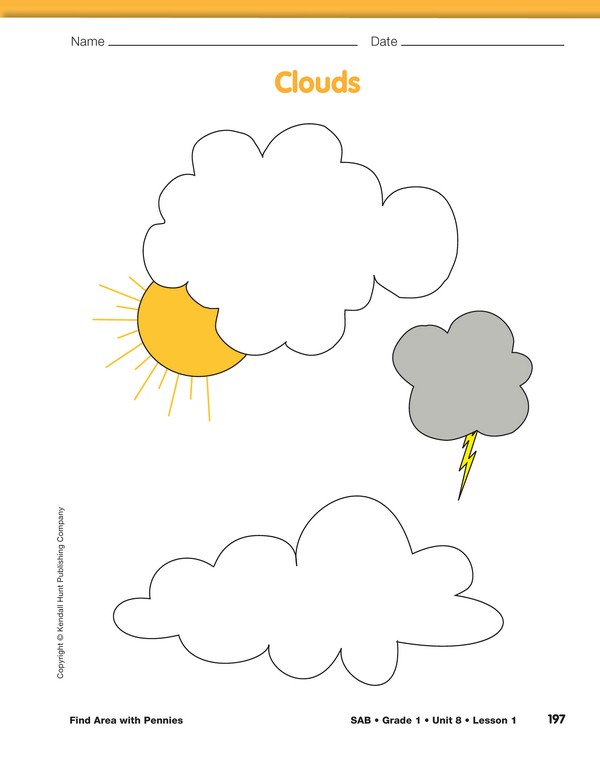
Identify Properties to Measure. Display the first Clouds page from the Student Activity Book and begin a discussion about the two white clouds.

Some students may interpret “larger” to mean “taller” while others may think “wider.” Point out to students that larger can have several different meanings. A third way to compare the size of two shapes is to see which one covers more space. Ask for suggestions on how to find which cloud covers more. Have students look at the Clouds page.

Students should be able to answer the first two questions just by looking at the pictures. The third question is trickier. Some students may suggest comparing how much space the clouds cover by placing one on top of the other. Demonstrate this approach using the three cutout clouds and placing one on top of the other. See Materials Preparation. The thunder cloud is clearly smallest, since it actually fits inside each of the other clouds. The two larger clouds can cover up the thunder cloud. It is difficult, however, to compare the two larger clouds since neither one fits on top of the other. Using this method, it is difficult to tell which cloud is larger.

Measure Area with Pennies. Encourage students to think of another way to find out which cloud is larger. Based on their experiences with measurement, some students may suggest covering the clouds with some unit. Suggest using a penny as a unit of measurement. Pennies have one desired property of a measurement unit—a uniform size and shape. Guide students to the idea of covering the clouds with pennies and counting how many fit inside each one. Students also may use pennies to compare how tall and wide the clouds are, but keep the activity focused on comparing the amount of space in each cloud.

Have students estimate the areas of the clouds by covering them with pennies. The cloud with the sun has an area of about 16 pennies. The thunder cloud has an area of about four pennies. The third cloud has an area of about 14 pennies.

When students find how many pennies fit in each whole cloud, explain that they have found an estimate of each cloud's area. Explain that the area is the entire space inside the cloud. The students have estimated the area by covering the cloud with identical objects (pennies.)

Estimate Quantities with Benchmarks. At this point, spend a moment discussing what the word estimate means. Write the word on the board.

From such a discussion, point out that when we estimate, we use what we know to make a smart guess about the number of objects or the size of something.

Explain to students that one way to use what we know to make a smart guess is to use benchmarks, such as 5 or ten, with which they are familiar. To facilitate using benchmarks, place 5 (or 10) pennies, beans, cubes or some small counters on a display and ask students to count them. Then place a larger number of the same object on the display in an arrangement that will make it hard for the students to count the objects exactly. Tell students to use the 5 (or 10) pennies as a benchmark and use them to make a good guess of the number of objects on the display. Hide the display. Write the numbers they guess under a display of the word estimate and explain to the students that their guesses are estimates.

Count out the exact number and compare it to the numbers the students guessed. Explain to the students the difference between guessing (estimating) and finding the actual number. Also mention that there are good guesses and bad guesses, good estimates and bad estimates. Give some examples of good guesses and bad guesses in the context of your demonstration with the pennies. For example, if you placed 20 pennies on the display, a good guess would be somewhere between 15 and 25. A bad guess could be 3, since it is clearly less than the benchmark number 5 (or 10). Another bad guess would be 100, since that would be an unreasonably large guess.

Practice using 5 (or 10) as a benchmark for estimating quantities by repeating the activity once or twice. Guide students in estimating the quantity first then counting to find the exact number. Discuss which estimates students think were good or bad and their reasons for their responses.

Estimate the Area with Pennies. Have students look at Clouds A and B on the second Clouds page. Before they measure the area using the pennies, ask them to think about the benchmark of 5 (or 10) pennies to estimate how many pennies they think will be needed to cover each cloud. Have them write their guesses on the spaces provided for each.

Then have the students find the area of each of the two clouds using the penny measure and write their measurement on the line provided for each. Tell students to compare their measurement with their estimates.

When students finish the Clouds page, have them share their results. Conduct a discussion of the activity as a whole. Focus on identifying strategies for making an estimate (a good guess) and on the difficulty of using the penny as a unit to measure area. See the Sample Dialog.