Review Estimation. Part 1 of this lesson is a review
of estimating quantities. Spend as much time on estimating
as is needed by the students. Students come
to school with an informal knowledge of numbers
and their relationships to one another. You may hear
students say that they are almost seven years old,
that they collected about 20 caps, or that it is close to
two o’clock. When students say that something is
almost, about, or close to something else they are
demonstrating number sense with estimation.
Read Shel Silverstein’s poem “Keepin’ Count” aloud
or simply have students imagine having to estimate
and count the number of flies flying around in a jar.
Display one of the estimation jars you prepared prior
to the lesson. See Materials Preparation.
-
When do you estimate? (Possible responses:
when I don’t need to have an exact amount or
actual sum; before I solve a problem to get an
idea of about how much the answer should be)
-
Do you think it would be easy or hard to estimate
the number of flies flying around in a jar? Why?
(Possible response: I think it would be hard
because they are moving around. It would be
hard to use a sample of ten flies to help estimate
like we have done with marshmallows and
cubes.)
-
Is it easier to estimate a number of moving flies or
the number of still [items] in my jar? (the still
items)
-
Is it easier to estimate when the items are all the
same size or different sizes? (all the same size)
-
Is it easier or harder to estimate if you can see
most of the items in the collection? (easier)
-
Would it be easier to make a good estimate of a
number of tiny grains of rice in my jar or the
number of [connecting cubes]? Why? (Possible
response: It would be easier to estimate the number
of connecting cubes and harder to estimate
the number of grains of rice because they are so
small and so many of them would fit in the jar.)
-
What if your estimate was 100 [connecting cubes]
more than there were in my jar? Would that estimate
be reasonable? (No, it is too far off when
there aren’t that many in the jar to begin with.)
-
What if you estimated there were 100 more tiny
grains of rice than there were in my jar? Might that
estimate be reasonable? (Yes, it could be a reasonable
estimate because there would be so many
grains of rice, it would be ok to be only 100 away
from the actual count.)
Show students the bag of 10 items.
-
How can you use bags of 10 and 100 to help you estimate the number of things in a collection?
(Possible response: I could see what 10 or 100 looked like. Then I guessed how many tens or hundreds there were in the collection.)
Set the estimation jar and the bag aside and tell students you will come back to them later in the lesson.
Use Benchmarks to Estimate Length. Display only
one of the long chains of connecting links you prepared
prior to the lesson. See Materials Preparation.
Keep the other long chain to the side.
- Talk with a partner. Estimate how many links are in
this chain without counting them.
Write students’ estimates on the board. Have them
help you place the estimates in order from smallest
to largest.
- Is there a cluster of estimates?
- Are the estimates spread out?
- What strategy did you use to make your estimate?
(Possible response: I tried to think about how
many groups of ten might be in the long chain.)
- Did anyone just guess? What is the difference
between a guess and an estimate? (Possible
response: A guess is just any number. I use strategies
that help me make an estimate that is more
accurate than just a guess.)
- Is there something that could help you to make a
better estimate? (Possible response: I’d like to
see how long 10 connecting links are.)
Remind students that using a benchmark is an excellent
way to refine guesses and make estimates. Hold
up the 10-link chain you prepared prior to the lesson.
Explain the meaning of the word benchmark. A
benchmark is a convenient or “friendly” number
like 10 or 100 that can be used to help make estimates
or compare numbers. Students can now make
improved estimates for the number of connecting
links in the long chain by comparing it with the
10-link chain benchmark.
- Now you know what a chain of ten links looks like.
If you would like to change your estimate, you
may.
- What made you think your estimate was too low or
too high?
Students might want to measure about how many ten-link chains are in the long chain. If they do so, they can skip count by tens.
If students choose to change their estimates, cross
out the original estimate and replace it with the new
one at or near the appropriate spot in the list on the
board.
- Now is there a cluster or is the list more spread
out than before?
If students have revised their estimates based on a
benchmark, the estimates should cluster around the
number of links. Ask a volunteer to group the links
by ten and count the actual number of links in the
long chain. Write this number on the board within
the list of estimates and circle it.
Estimate the Number of Links in Two Chains.
Display the second long chain of links. This chain
should be a different color from the first so that students
can see where one chain ends and the other
begins. Connect the chains.
- If we put these two long chains of links together,
what would be a “crazy” estimate for the total
number of links? How do you know? (Possible
response: 1000; It’s crazy because there were
only [number] links in the first chain and the second
chain is just a little bit longer, so 1000 is too
many.)
- What would be a “could be” estimate?
- What might help you make a good estimate rather
than just a guess? (Possible response: the 10-link
chain)
-
Talk with a partner. Estimate the total number of
links when we put the two long chains together.

Estimate other quantities in the classroom. Placing cubes,
pennies, or other objects in a jar for students to estimate is
a time-tested, engaging activity. Each time, record students’
estimates on the board and discuss the strategies they
used to make them. Have students help you place the
estimates in order.
- Is there a cluster of estimates? If so, circle the cluster.
- Are the estimates spread out?
- Of the estimates listed, which do you think is the smallest reasonable estimate?
- Which one would be the largest reasonable estimate?
- Describe the pattern of the estimates, if you see one.
Use benchmarks to find the number of counters in a jar.
Count the number of counters in about one-fourth of the jar
and estimate how many counters could be in the whole jar.
Or, count how many counters it will take to fill a smaller jar,
and estimate how many smaller jars it will take to fill the
larger jar. Discuss other strategies for making estimates.
This can also be used as a learning center activity.
Give students time to discuss how to estimate the
total number of links in the combined chains. Record
students’ estimates on the board in order from smallest
to largest as you did for the first chain. Then have
students share their estimation strategies.
- How did you estimate the total number of links?
(Possible responses: I compared the length of the
first chain and the second chain. I knew the total
was going to be more than [number] because that
is how many links the first chain had. The total
might be double the number in the first chain. Or,
the [second] chain had about [number] more
links than the first one, so I put those numbers
together to find a total.)
- Did anyone use the 10-link chain? How? (Possible
response: I thought about how many groups of
ten were in the long chain.)
- Did thinking about the “could be” and “crazy” estimates
help you make an estimate? If so, how?
(Possible response: I did not choose crazy numbers
that were too high or too low. I thought
about the could be numbers when I made my
estimate.)
- How can you find the total number of links?
(Possible responses: We could count all the links.
Or, since we know how many are in the first
chain, we could just count the links in the second
chain and add the two numbers together.)
- Would you expect the total number of links to be
somewhere in this range of estimates? (yes)
Add the Number of Links in Two Chains. Count
the actual number of links in the second chain and
add that number to the number of links in the first
chain. Encourage students to use number lines, the 200 Chart, or any strategy to find the sum. Write a number
sentence to show that the addition of one number
of links to the other equals the total number of links.
Ask a student volunteer to group and count the total
number of links in the combined chains to verify.
Add this number to the list on the board and circle it.
Compare the total number of links to students’ estimates.
- Does a total of [number of total links] sound reasonable?
How do you know? (Possible response:
Yes; We estimated the total would be about this
much. I looked at our list of estimates and this
total is within the list.)
- How does estimating help you decide whether
your answer is reasonable? (Possible response: If
my answer is far off from my estimate, I look
back and see if I might have made a mistake.)
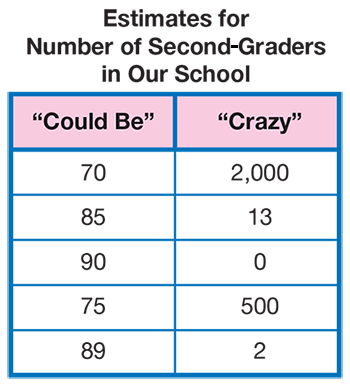
Use Could Be or Crazy Numbers to Estimate.
Challenge students to estimate even larger numbers.
As they make estimates, list them on the board in
two columns as shown in Figure 1.
- Think about how many students there are in the
entire second grade in our school. If we put all the
classes together, what would be some “crazy”
estimates? (Possible responses: 5 students or
5000 students)
- What would be some “could be” estimates? Why?
(Possible response: 90 students [depending on
the size of the school])
Encourage students to describe how they arrived at
their estimates and how they determined what made
a “could be” or a “crazy” estimate. Students might
say that they made their estimate based on the number
of students in their class and the number of
classes in the second grade.
- How does listing “could be” and “crazy” numbers
help to make a good estimate?
Students may notice that the numbers in the “could
be” column are all close to one another. These estimates
represent reasonable solutions to the problem.
Ask students to tell why each “could be” or
“crazy” estimate is reasonable or unreasonable. For
example, the “crazy” estimate of 5 is unreasonable
because there are more than 5 students in just one
class in the second grade. The “could be” estimate of
90 is reasonable because there are about thirty students
in each of the three second-grade classes.
- Look at the “could be” estimates. Use this information
to estimate the number of second graders
in our school.
Decide on an estimate as a class. Then tell students
the actual number of second-graders. Compare the
estimate to the actual number.
- Was our estimate reasonable? Why do you think so?
Discuss the accuracy of the estimate. Consider the
estimate based on the total number of second-graders. For example, if there were 100 students, an
estimate of 50 is inaccurate. However, an estimate of
90–110 would be very reasonable.
Assign Home Practice Parts 1 and 2 for homework.