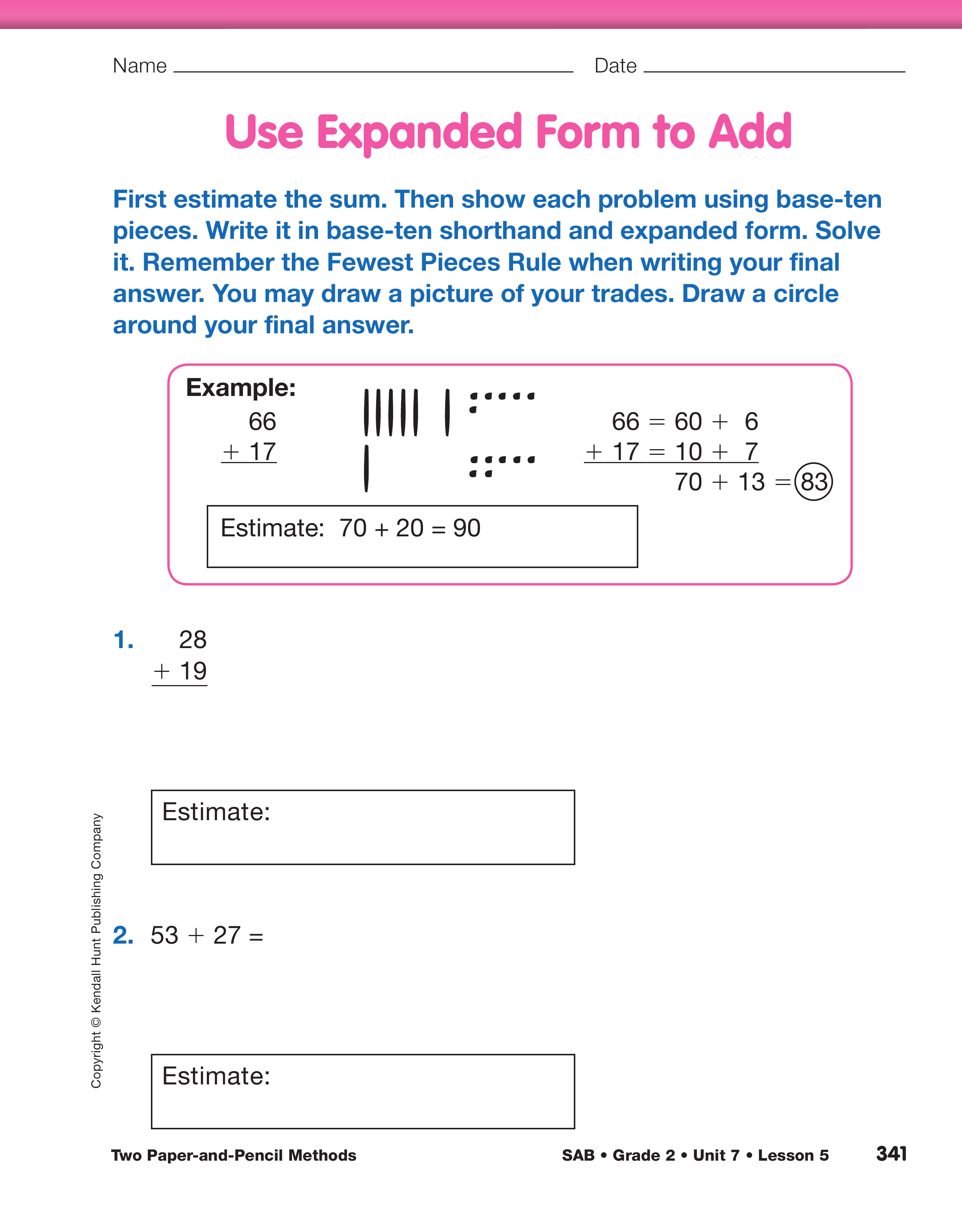
Estimate the Sum. Display the problem:
Ask students to look at the 200 Chart in the Student Activity Book Reference section.
- Look at your 200 Chart. What friendly numbers can you use to estimate this sum? (For 48, the closest ten is 50 and for 26 the closest ten is 30. I estimate a sum of 80.)
- Can anyone use a different strategy to estimate the sum? Explain. (Possible response: I think about money. 48 is close to 2 quarters and 26 is close to 1 quarter. 3 quarters is 75.)
Connect Base-Ten Pieces to Expanded Form. Distribute base-ten pieces to students and have them model the two numbers in the addition problem using skinnies and bits.
- How did you model 48? (I used 4 skinnies and 8 bits.)
- How can you write that as a number sentence? (40 + 8 = 48)
- How did you model 26? (2 skinnies and 6 bits)
- What is that as a number sentence? (20 + 6 = 26)
As students give the number sentences, add them to the problem as shown below:
| 48 = 40 + 8 |
| + 26 = 20 + 6 |
- Is this still the same problem? (Yes)
- Is 40 + 8 the same number as 48? (Yes)
- How is writing it this way like your base-ten
pieces? (It is like splitting the number into skinnies and bits.)
- Does writing the problem this way make it easier
to solve? How? (Yes, you can add tens and add ones.)
If helpful, cover up the left side of the equal sign so that just the expanded form is visible.
- Who can solve the problem when it is written this way? How do you solve it? (Possible response: I think you add the value of all the skinnies and then you add the value of all the bits.)
Give students time to finish the problem with their partners using the base-ten pieces. Ask if their answer is close to their estimate.
Help students make connections between the base-ten pieces and the written numbers using prompts such as:
- How many skinnies do you have in all? (6)
- How much do the 6 skinnies represent? (6 tens, or 60)
- How many bits do you have in all? (14)
- What do they represent? (14 ones)
Add the answer line as follows:
| 48 = 40 + 8 |
| + 26 = 20 + 6 |
| 60 + 14 |
- Is this the final answer to the problem? Have we finished it? What do we still have to do? (No, it isn't finished. We have to add 60 + 14.)
Add to the problem:
| 48 = 40 + 8 |
|
| + 26 = 20 + 6 |
|
| 60 + 14 |
= 74 |
- How many skinnies, or tens, are there now? (7)
- How many bits, or ones? (4)
- What is the answer? (74)
- Is that close to our estimate? Is this answer reasonable?
Tell students this is a way to solve problems using paper and pencil. We will call it the expanded-form method. Some problems are too difficult for mental math and it´s not possible to always carry tools like base-ten pieces.
- Why do you think this paper-and-pencil method is called the expanded-form method? (Possible response: Expand means to get bigger. When we decompose the numbers in the problems into tens and ones, we are making the problem look bigger on the paper.)
Direct students´ attention to the Use Expanded Form to Add pages in the Student Activity Book.
Work through the example problem together. Display the Addition Strategies Chart from Lesson 2.
Tell students that they can refer to these strategies when solving Question 6.
Have students work with partners to complete the problems. Remind them to use their estimation strategies
to determine whether their answers are reasonable. As students work, use discussion prompts similar to those used to discuss 48 + 26 to
them make connections among their work with the pieces, shorthand, and written numbers.
When students finish, discuss the problems and have students demonstrate their solutions. For Question 6,
discuss the various mental math strategies and tools (200 Charts, number lines) that students used. List the strategies on the board. As you list a strategy,
identify it with the name of the student who described it or another unique title. See Figure 1.
Discuss Efficiency. Compare these strategies with those on the Addition Strategies Chart. Ask students which method or strategy, including the expanded
form method, they think is most efficient for them. Develop with students a definition for efficient and a description of an efficient method. See Content
Note. Make sure it includes the following characteristics:
- reaches the correct answer
- fits the problem
- can be done in fewer steps
- can be done quickly
- is easy to understand

Efficient Methods and Strategies. In discussing the relative efficiency of a strategy, reinforce with students that
what may be most efficient for one student may not be most efficient for another. Sometimes a strategy is clearly less
efficient, such as counting all (from one) when adding two 2-digit numbers. On the other hand, in the problem 28 + 19,
different mental math strategies may prove most efficient for different students. One student may mentally
add 1 to the 19 to make 20, count up two tens from 28, then subtract 1 (28, 38, 48, 47). Another student may
choose friendly numbers 30 and 20, make a note that they are +2 and +1 larger than the actual number, add the
friendly numbers, and then subtract 3
(30 + 20 = 50; 50 – 3 = 47). Another student may not be ready for strictly mental
strategies and may choose to use the 200 Chart or the number line to perform essentially the same steps. The important
thing here is that students have frequent opportunities to explain their reasoning orally and that they listen to each
others´ explanations, make sense of them, and have opportunities to try those strategies also.
Also, an efficient method or strategy must fit the problem. To solve the problem 34 + 78, a paper-and-pencil method
will probably be most efficient for students in Grade 2. Students should observe, however, that it would not be the
most efficient strategy for solving 24 + 75, even though at first glance the problems may appear to be similar. A mental math strategy that uses friendly numbers or money
will be more efficient and is more likely to produce an accurate answer.