Estimate Sums. Remind students of the strategies
they used to estimate sums for addition problems in
Lesson 1 of this unit:
- Adding Tens
- Counting On by Tens
- Using Friendly Numbers
- Using Coins
Display the following addition problems and ask students
to estimate the sum for each problem:
| 43 |
38 |
155 |
117 |
| +16 |
+21 |
+134 |
+52 |
Explain to students that estimating the answer is
important because it will help them determine if
their actual sums are reasonable. Have students estimate
the sums first by identifying in which interval
(between which two tens) the answer will be.
For the problem 43 + 16, ask:
-
Will the total be more than 40? How do you know?
(Possible response: It has to be more than 40
because you start with 43 and you are adding 16.)
-
Between which two tens do you think the total will
be? Why do you think so? (Possible response: I
think it will be between 50 and 60 because if you
put the tens together, you get 50 and there are not
more than 10 ones, so the answer will probably
be between 50 and 60.)
-
What is your estimate for the problem [43 + 16]?
(Possible responses: 50, 60)
-
What strategy did you use to find your estimate?
(Possible response: I used friendly numbers. 43 is
close to 40 and 16 is close to 20; 40 + 20 = 60.
My estimate is 60.)
-
Did anyone use a different strategy? (Possible
response: I added tens. I added 40 + 10 and that
equals 50. My estimate is 50.)
-
Do you think the actual sum is closer to [50] or
[60]? (Possible response: I think it’s closer to 60
because if you add the tens, you get 50, but you
have to add the ones, too. 3 + 6 is close to
another 10, so I think the estimate is closer to
60.)
Include addition problems with sums to 500 that do
not involve trading, such as 155 + 134. Help students
understand how estimating 155 + 134 is similar
to estimating 55 + 34. For example, to use
friendly numbers for 55 + 34, add 60 + 30 = 90.
To use friendly numbers for 155 + 134, add
160 + 130 = 290.
Add Tens and Ones. Have students model the previous
problems using base-ten pieces. Remind students
how to use base-ten shorthand to draw a
representation of their pieces: a box ( 
) to represent
a flat, a vertical line ( | ) to indicate a skinny, and
a dot ( • ) to indicate a bit. Ask questions that make
connections between the base-ten pieces and the
numbers in the number sentences.
For example, for the problem 43 + 16, ask:
-
How can we use skinnies and bits to model the
number 43 using the Fewest Pieces Rule? (4 skinnies
and 3 bits)
-
How can we model that number using base-ten
shorthand? (|||| ••• )
-
How can we use skinnies and bits to model the
number 16? (1 skinny and 6 bits)
-
How can we model that number using base-ten
shorthand? (|••••••)
-
How can we write 43 to show we have broken it
into 4 tens and 3 ones? (40 + 3)
-
How can we write 16 to show that we have broken
it into 1 ten and 6 ones? (10 + 6)
Students use the Fewest Pieces Rule to make numbers using
the fewest number of base-ten pieces. For example, the
number 43 can be represented with 43 bits, 3 skinnies, and
13 bits, 2 skinnies and 23 bits, or 1 skinny and 33 bits. After
making all trades that are possible, 43 is represented by 4
skinnies and 3 bits. Help students understand that 4 in the
number 43 represents 4 tens or 4 skinnies and the 3
represents 3 ones or 3 bits.
Write the problem in expanded form:
The expanded form shows a number expanded into an
addition statement. Forty-three in expanded form is 40 + 3.
One hundred twenty-five in expanded form is 100 + 20 + 5.
- When I write the problem in expanded form, how is
it like modeling with base-ten pieces? (Possible
response: For 43, 4 skinnies is the same as 40
and 3 bits is the same as 3. For 16, 1 skinny is the
same as 10 and 6 bits is the same as 6.)
Have students solve the problem. Ask students to
share their strategies using base-ten pieces and number
sentences. See Figure 1 for possible strategies to
solve 43 + 16.
If students name only the digits in the tens place by
saying “4 + 1 equals 5,” ask them to tell you the
value of each digit.
- What is the value of the 4 in 43? (4 tens or 40)
Have students solve additional problems with sums
to 500, including adding two numbers with hundreds
that do not involve trading of base-ten pieces. For
example, for the
problem 155 + 134, students will
need flats, skinnies, and bits to find the sum.
Demonstrate how to use base-ten shorthand to represent
flats ( ) and ask:
-
How can you use flats, skinnies and bits to model
the number 155 using the Fewest Pieces Rule?
(1 flat, 5 skinnies, and 5 bits)
-
How can you model that number using base-ten
shorthand? (1 box, 5 lines, and 5 dots) [Write the
base-ten shorthand next to the number.]
-
How can you use flats, skinnies and bits to model
the number 134? (1 flat, 3 skinnies, and 4 bits)
-
How can you model that number using base-ten
shorthand? (1 box, 3 lines, and 4 dots) [Write the
base-ten shorthand next to the number.]
-
How can you write 155 to show we have broken it
into 1 hundred, 5 tens and 5 ones? (100 + 50 + 5)
-
How can you write 134 to show that we have broken
it into 1 hundred, 3 tens and 4 ones?
(100 + 30 + 4)
Write the problem in expanded form:
| 155 = 100 + 50 + 5 |
| 134 = 100 + 30 + 4 |
-
When I write the problem in expanded form, how is
it like modeling with base-ten pieces? (Possible
response: For 155, 1 flat is the same as 100, 5
skinnies is the same as 50, and 5 bits is the same
as 5. For 134, 1 flat is the same as 100, 3 skinnies
is the same as 30, and 4 bits is the same as 4.)
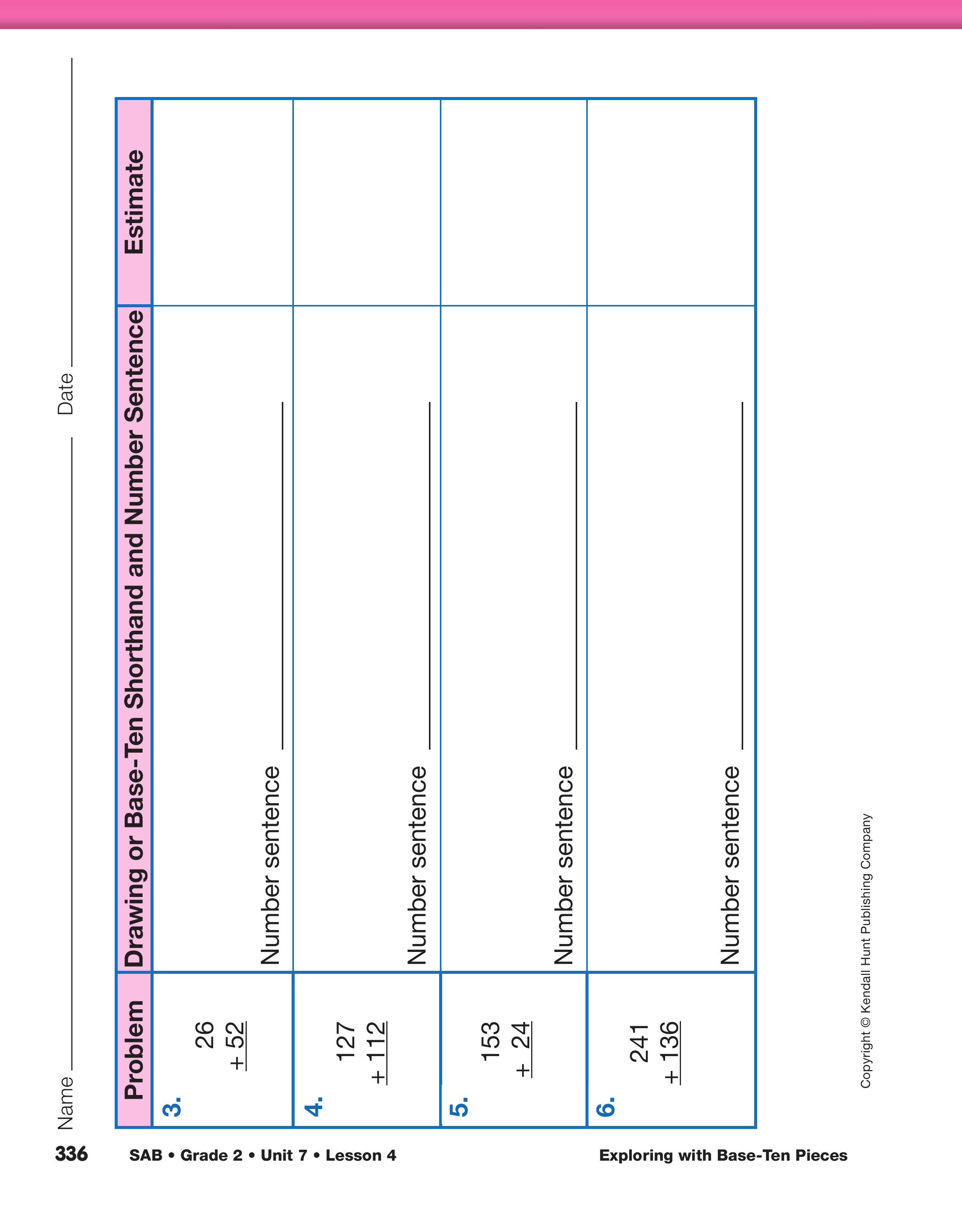
Practice Adding with Base-Ten Pieces. Use the
display of the Add with Base-Ten Pieces page from the Student Activity Book to
model how to use base-ten shorthand to record students’
solutions and estimate to check for reasonableness.
Help students make connections between
the base-ten pieces, the base-ten shorthand, and the
number sentence.
-
Look at this different example: 17 + 12. Show with
base-ten pieces how you can use skinnies and bits
to represent 17. (1 skinny and 7 bits)
-
Show with base-ten pieces how you can use skinnies
and bits to represent 12.
(1 skinny and
2 bits)
-
How can you show the problem using base-ten
shorthand? (Draw 1 line and 7 dots to represent
17 and 1 line and 2 dots to represent 12.)
-
Find the sum of 17 and 12 and write it under the
problem. (29)
-
How can you check to see if our solution is reasonable?
(Possible response: Use friendly numbers:
17 is close to 20 and 12 is close to 10. Add
20 and 10 and our estimate is 30. Our answer is
29 and that’s close to our estimate of 30.)
Have student pairs work together to solve the problems
on the Add with Base-Ten Pieces page in the
Student Activity Book using base-ten shorthand to
record their solutions and then estimate to check
their answers for reasonableness. Remind students to
write the sum for each problem.
After students solve each problem using base-ten
pieces, ask them to use other strategies to check their
solutions. For example, to solve 17 + 12, move on
the 200 Chart or skip count by tens and ones. Help
students develop the habit of checking to see if their
answers are reasonable by estimating or by using a
different strategy.
