Introduce the Story Leonardo the Traveler. Begin the lesson by asking students to read the story Leonardo the Traveler in the Student Guide. Students can read it individually, with a partner, or in a small group before reading and discussing it together as a class.
The story refers to various locations in Europe, North Africa, and the Middle East. Point out these places on a map or globe before using the prompts to help guide a class discussion about the story Leonardo the Traveler.

Leonardo the Traveler is about the great Italian mathematician Fibonacci and his role in introducing Hindu- Arabic numeration and associated algorithms to Europe. This story is based on fact. The hero, Leonardo, is known today as Fibonacci. Born in Pisa about 1170, he died about 1250 and is considered the greatest mathematician of the Middle Ages. Fibonacci is most famous for a certain number sequence (1, 1, 2, 3, 5, 8, 13, …) he investigated and that today bears his name.
The basic outline of the story is historically accurate: Leonardo's father really was a merchant, and Leonardo did live in Bugia and traveled in North Africa and the Middle East. On these travels he met Arab mathematicians who used different and more efficient strategies and representations than the European mathematicans at that time. In particular, Leonardo learned about Hindu-Arabic numeration and wrote about the new system in his Liber Abaci, Book of the Abacus, published in 1202. Finally, Leonardo did sign his name Bigollo, which can mean Leonardo the Blockhead or Leonardo the Traveler, although the exact reason is unclear. The details of the story of Leonardo's discussions with his parents and the Arabs, however, are fictionalized.
Read Aloud and Discuss Leonardo the Traveler. Discuss the story using the prompts:
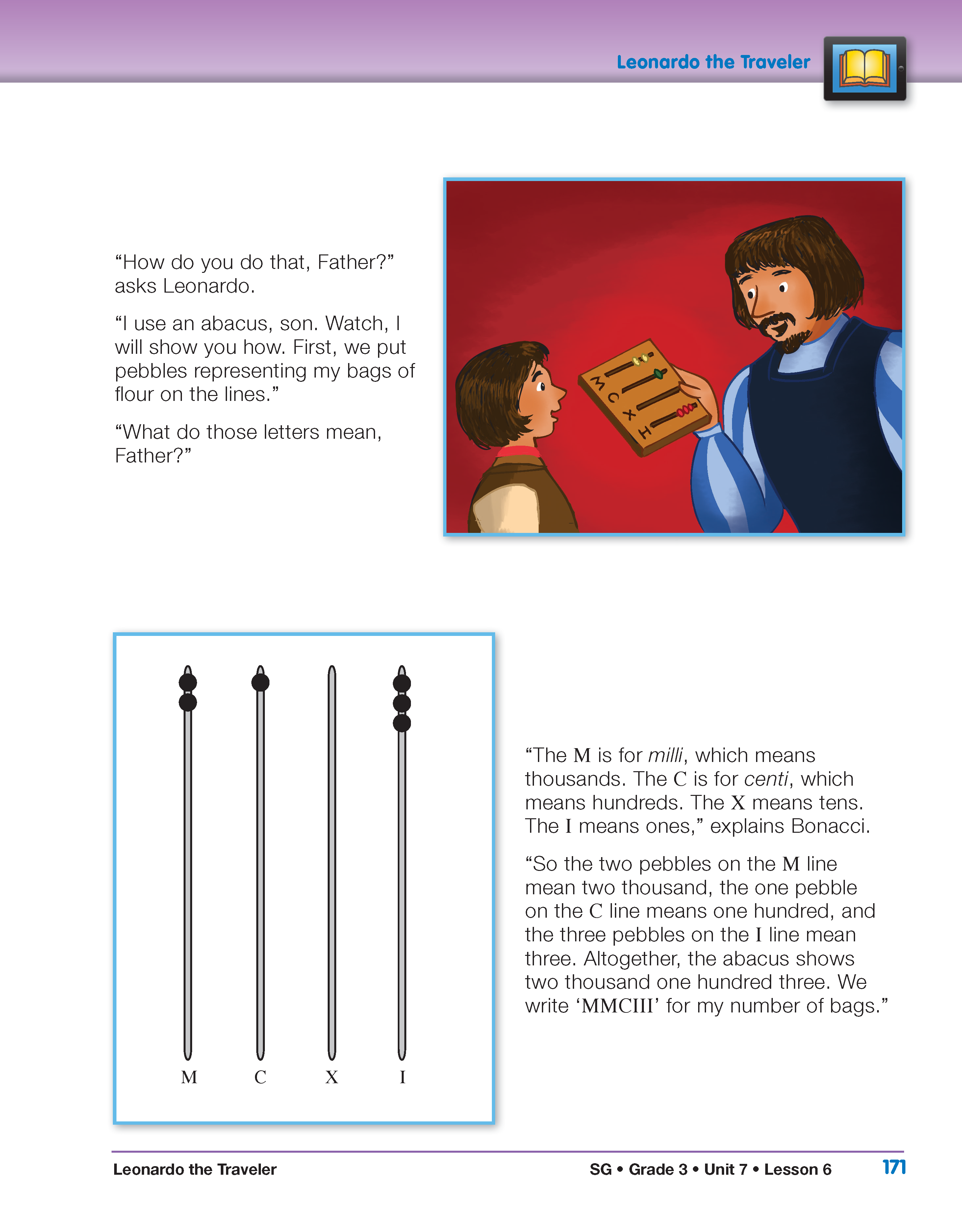
Page 170
- How would you solve this problem for Leonardo and his father? (Possible response: I could write a number sentence, 2103 + 1338 =
 and use expanded form, all-partials, or the compact method. Other responses may include using a number line or base-ten pieces.)
and use expanded form, all-partials, or the compact method. Other responses may include using a number line or base-ten pieces.)
If an abacus is available, use it to show students what the story is referencing. If using a modern abacus, point out that it has wires in place of grooves incised on the board's surface.
Page 171
Bonacci's abacus can also be called a counting board. The pebbles are not fixed on wires as on modern abacuses, but are removable and placed in grooves incised on the board's surface. We get our word counter from the custom of embedding such counting boards into tabletops in stores. The word calculate comes from the Latin calculi, pebbles, from the use of pebbles on such counting boards.
- What English words come from the Latin word parts milli- and centi-? (Milli- means thousand: millennium, millimeter; centi- means one-hundred: century, centimeter.)
- What number sentence would you write to represent the value of the number shown on the abacus?
(2000 + 100 + 3 = 2103)
- How is showing 2103 on an abacus like showing 2103 with base-ten pieces? How is it different? (You don't show anything for the tens for both because there are none. Both the base-ten pieces and the abacus show the value of the number just in different ways. The abacus uses the number of pebbles in a specific column. The base-ten pieces use the sizes of the blocks to tell you how much it is worth.)
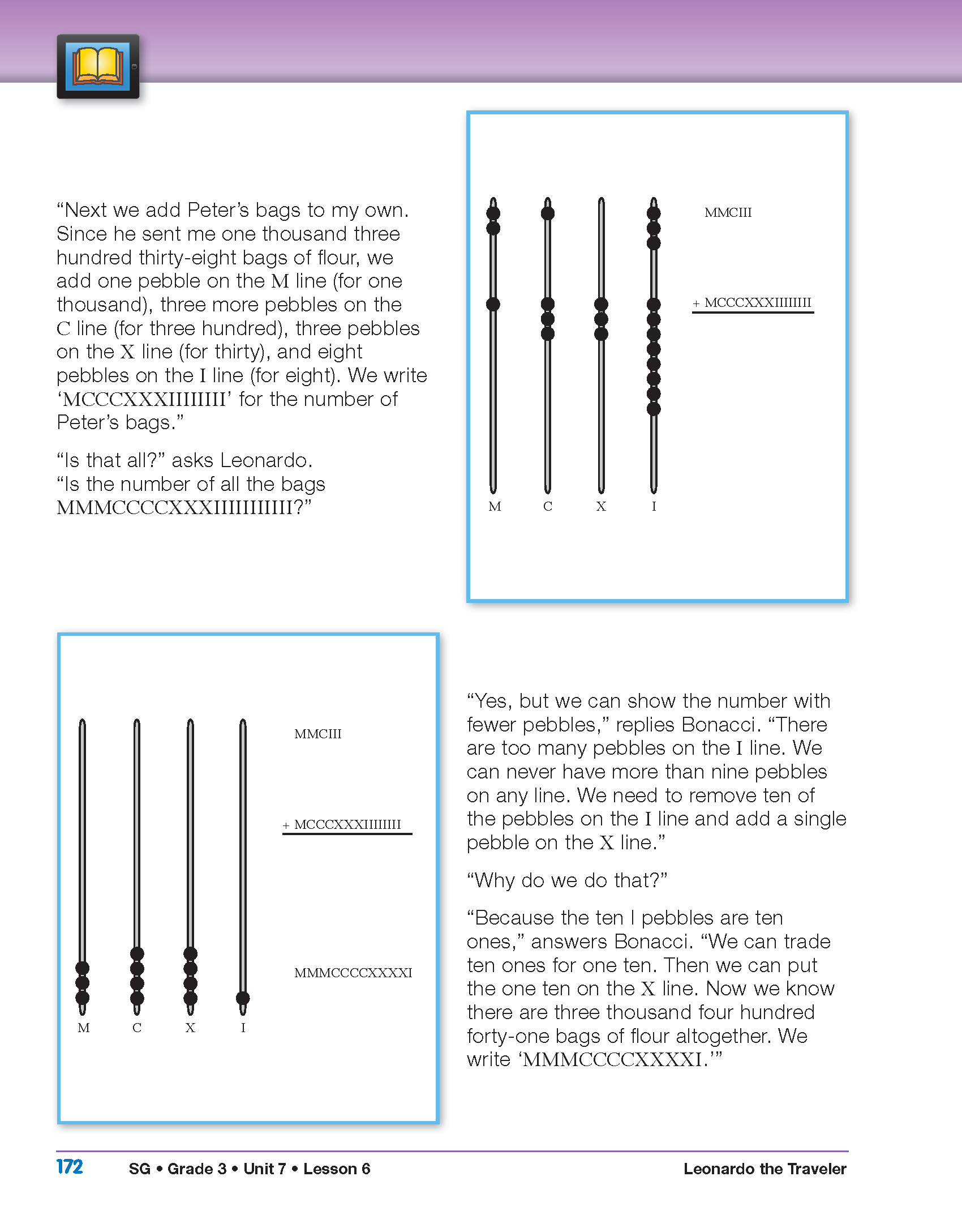
Page 172
The version of Roman numerals used in the story is simplified. No use is made of two features normally seen in Roman numerals: positional subtraction (e.g., IX = X − I) and letters that are not powers of ten (V, L, etc.). This simplified version is, however, quite functional and has some historical basis.
After reading the first paragraph ask:
- What quantity does the second set of pebbles on the abacus represent? (one thousand three hundred thirty-eight)
- How is the number of Peter's bags written in Roman Numerals? (MCCCXXXIIIIIIII)
- How would we write that number? (1338)
- How do Roman numerals compare to our way of writing numbers? (Roman numerals use letters to represent the values: M = thousands, C = hundreds, X = tens, and I = ones. They just keep writing the letters a certain number of times to tell how many, like CCC means 3 hundred. Then they add up all the values in their heads to say the number out loud. We just use numbers 0 to 9 and write them in a certain place to tell the value of each amount, like 123 is one hundred twenty-three because 1 is in the hundreds place, 2 is in the tens place and 3 is in the ones place.)
Regrouping and multiple partitioning of numbers are major themes in this story, so there are many opportunities for discussion.
Continue discussing the remainder of the page using the prompts which follow.
- Look at the changes Bonacci made on the abacus on the bottom of this page. How is this like the Fewest Pieces Rule? (It's the same. He said that there can never be more than nine pebbles on any line on the abacus just like there can't be more than nine bits in any column in base-ten. When you have ten in one column you trade them for the next larger piece like Bonacci traded ten ones pebbles for one ten pebble.)
- Show how to solve Bonacci's problem with baseten pieces or numbers. (Possible response: I would use numbers and write 2103 on the top row and 1338 on the bottom row lining up the ones, tens, hundreds, and thousands carefully, then add, remembering to make trades if any column adds up to more than 9.)
Page 174
- How can the same mark, 3, sometimes mean three hundred and other times three? (The 3 means 3 hundreds when it is the hundreds place or when there are 3 pebbles in the hundreds column on the abacus. It just means 3 ones when it is in the ones place.)
- Do you think Hindu-Arabic numbers, our numbers, are easier than Roman numerals? (Responses will vary.)
- Why does Leonardo's father say, “Do not be a blockhead”? (Because his father didn't understand the new numbers.)
- Where is Pisa? In what modern country is Pisa? What famous building is in Pisa? (Italy. The Leaning Tower of Pisa.)
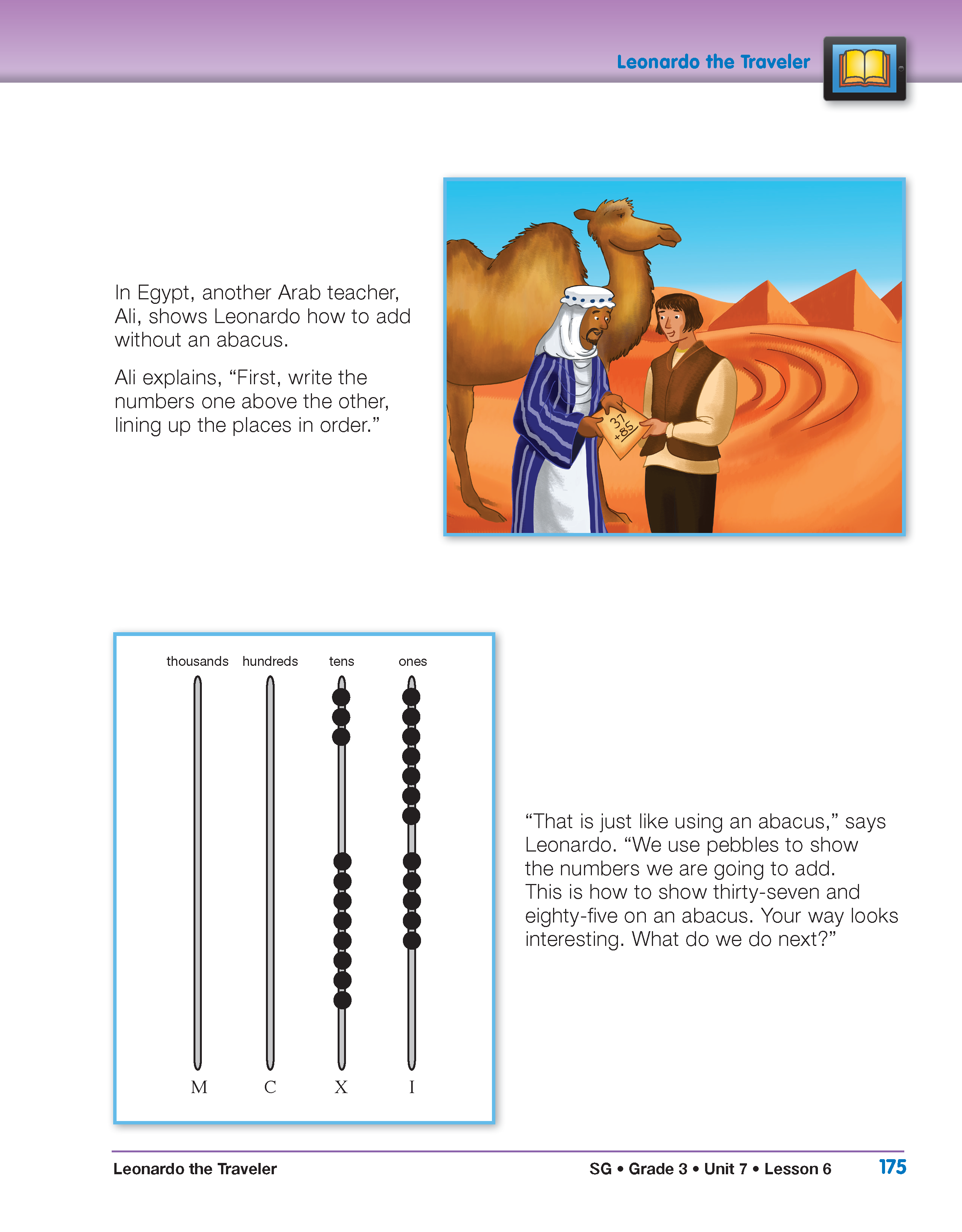
Page 175
- What is the number on the top part of the abacus? (37)
- What is the number on the bottom? (85)
- How would we use expanded form or the compact method to solve 37 + 85? Which method do you think Ali will show Leonardo? (Let some children demonstrate each of the methods on chart paper, at the board or other display, or dictate the steps to you as you record their responses on a class display. Responses may vary, but most will say that Ali will show Leonardo the compact method.)
Page 176
- What method did Ali show Leonardo? (Ali used the compact method.)
- Look at the abacus. How are the pebbles in the tens column like the numbers in the compact method? (The problem has a 1, 3, and 8 in the tens column and the abacus has that many pebbles.)
- Leonardo talks about trading 10 ones-pebbles for one tens-pebble. Where is the one tens-pebble on the abacus? (at the top of the tens column)
- How many pebbles are in the tens column on the abacus altogether? (12)
- How much is 12 tens? How do you know? (120. Possible response: 12 dimes is the same as 120 pennies.)
Page 177
- Why did Leonardo make another trade? (He traded 10 tens-pebbles for one hundreds-pebble.)
- What would that trade look like with base-ten pieces? (It is like trading ten skinnies for a flat.)
Page 178
- Do you agree that “Subtraction is a little harder, but you will learn it if you try”? (Answers will vary.)
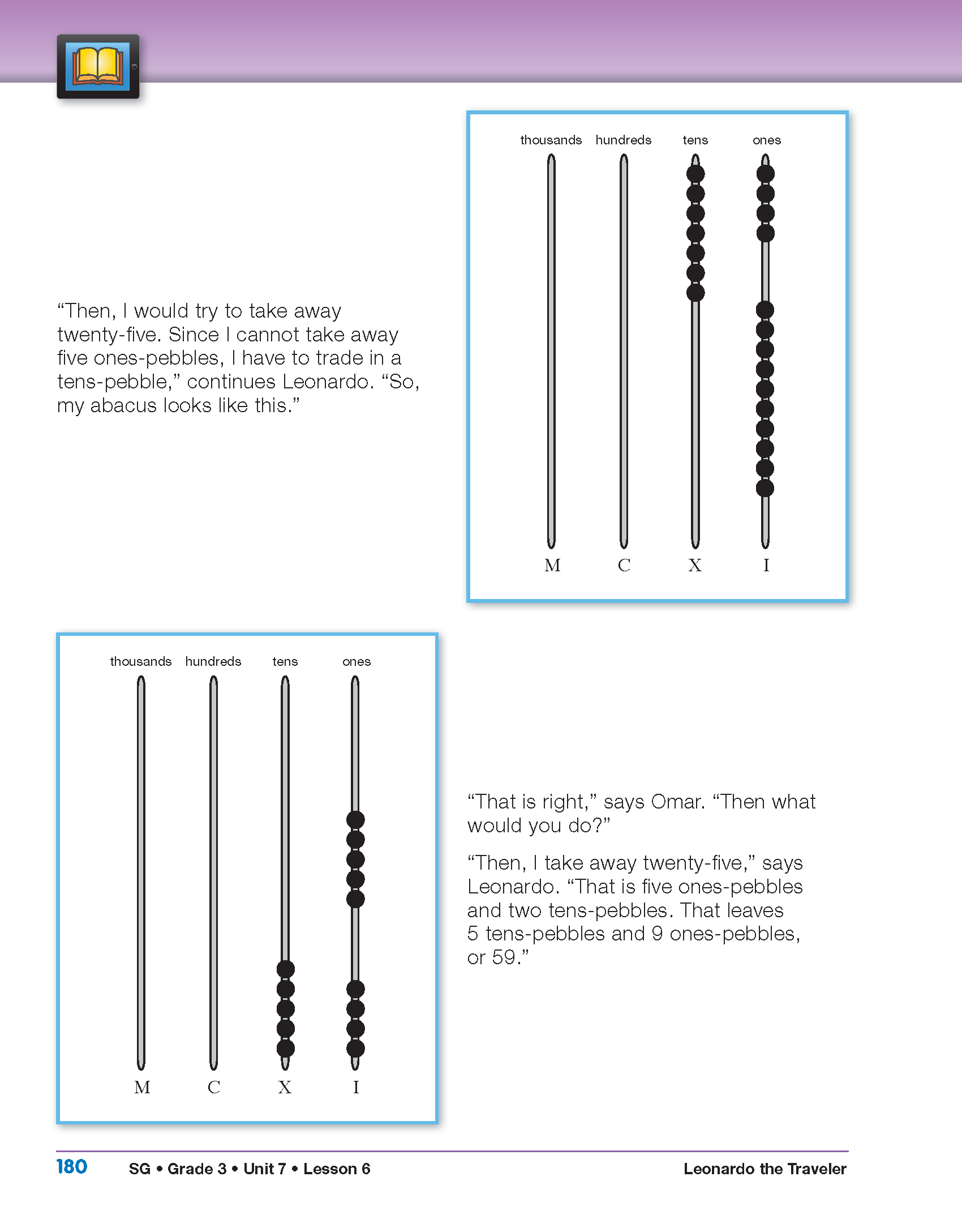
Page 180
- How many tens and how many ones does the abacus show? (7 tens and 14 ones)
- Does the abacus still show a number that is equal to 84? (yes)
- Can you write a number sentence for what the abacus shows? (70 + 14 = 84)
- How would you use the compact method to solve 84 – 25? (Possible answer: I would write the problem like Omar did and regroup one ten for ten ones. To show the regrouping I would write a little 7 above the 8 in the tens place and 14 above the 4 in the ones place.)
Page 181
- What does the 7 above the crossed-out 8 stand for? (7 tens or 70)
- What about that little 14 above the 4? (14 ones)
- Can you write a number sentence to show what the top number is? (70 + 14 = 84)
- Compare the steps on the abacus and the steps using the Hindu-Arabic numerals. How are they alike? Different? (The steps are the same except the abacus uses pebbles that you trade and move from the tens to the ones place like using baseten pieces and trading a skinny for ten ones. With numbers you think about the base-ten pieces in your head and write little numbers in the correct column to show the trades.)
Page 182
Read the last two pages of the story then use the prompts to help students understand the historical and multicultural roots of the Hindu-Arabic number system and to connect mathematics and social studies.
- Find Syria and Greece on the map on page 178.
- What do you think Leonardo said to his father to explain the Hindu-Arabic system? (I think Leonardo would explain that numbers are lined up in columns and each column has a different value. The same number could be used in different places because the column tells its value. He could tell how to use the digits 0–9 to show values by writing them in the correct column on paper like the compact method instead of putting the actual pebbles on the abacus or writing all the different letters over and over like when using Roman Numerals.)
- What strategy would you use to add 37 and 68? (Responses will vary.)
Page 183
- Do you think Leonardo was a blockhead? (Answers will vary but many will say that Leonardo learned quickly and the Hindu-Arabic system was easier to use than an abacus or Roman Numerals, so he was really smart!)