Welcome to First Grade
REPRESENTATIONS and TOOLS

A significant indicator of conceptual understanding is being able to represent mathematical … read more
"A significant indicator of conceptual understanding is being able to represent mathematical situations in different ways and knowing how different representations can be useful for different purposes" (National Research Council, 2001).
In Unit 1, students use tallies, counters, and number lines to represent quantities. These tools help students develop number sense by giving them concrete and visual representations of the size and order of numbers. Students need to have these tools readily available as they work, so they can choose the representations that make sense to them and make connections among representations.
The Number Line
In this unit, students locate numbers on the number line and move on the number line. … read more
The Number Line
In this unit, students locate numbers on the number line and move on the number line. Students will start by making single hops and will describe moving from one number to another. This representation places demands on students' one-to-one correspondence and number sense and can be a challenge for some students in early first grade. This demand will help develop these skills and is a representation used and developed throughout Math Trailblazers to support students' number sense and mental math skills. At this stage in development, be sure all the numbers on the number line are marked and moves are made one at a time. See Figure 1. In later grades students will use open number lines and group hops to represent larger numbers.
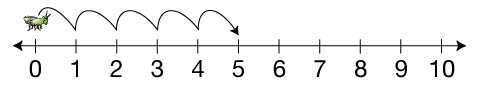
Figure 1: Number line with a math hopper
Counters
Many objects can be used as counters: connecting cubes, connecting links, beans, etc. In this … read more
Counters
Many objects can be used as counters: connecting cubes, connecting links, beans, etc. In this unit, connecting cubes are used as a visual representation of a number, to build a number line, and to practice counting on. They have many advantages over other kinds of counters because connecting cubes can be put together to form trains, trains with patterns, and arrays. See Figure 2.

Figure 2: Connecting cubes organized to show 8
Tallies
Tallies are used in this unit to keep track of objects and observations students are counting. … read more
Tallies
Tallies are used in this unit to keep track of objects and observations students are counting. Students group the tallies by five, making it easier to visualize and count.
DEVELOPING MATH FACTS

Initial work with math facts in first grade involves working with strategies … read more
Initial work with math facts in first grade involves working with strategies in the context of counting and problem solving. The first strategy students explore is counting on. As children learn to count, they usually start at one and count all the objects. The shift from needing to count from one to counting on from a larger number is an important step in children's development. Some children will need extended time and multiple opportunities before counting on is natural for them. For example, to add five and two, students need to hold five in their head as a set and then count on two more until they reach seven. This ability comes more easily to some children. Some may need to spend more time on the counting-all strategy before moving to the more efficient counting-on method for addition facts and problem solving. Our approach is based on educational research and is supported by the National Council of Teachers of Mathematics' Principles and Standards for School Mathematics (2000). It is characterized by the following:
Emphasis on problem solving. Students will learn the basic facts if they are encouraged to use a problem-solving approach. Students can invent their own strategies, learn from their peers, or learn from the teacher through class discussions. Students will discover the need to learn the facts as they encounter them in labs, activities, and games.
De-emphasis of rote work. Students learn their math facts, but we de-emphasize rote memorization, and in first grade we do not administer timed tests. These can produce an undesirable result—students perceive that doing math is memorizing facts and rules which "you either get or you don't." Instead, students should have confidence they can find answers by using strategies they understand.
Throughout first grade the focus remains on using strategies that are meaningful to students. Beginning in Unit 11, a systematic organization of the math facts is introduced via the Daily Practice and Problems. Facts are grouped together to help students think of them in a more efficient manner. However, students all still solve the problems using whatever strategies they wish.
By the end of second grade, students in Math Trailblazers are expected to demonstrate fluency with the addition and subtraction facts. The first-grade curriculum enables them to build to this fluency through experience with and understanding of the addition and subtraction concepts.
Facts will not act as gatekeepers. Students are not prevented from learning more complex mathematics based on their fluency with the math facts.
Positive Attitude, Group Work, and Communication
Fostering a positive attitude toward mathematics is an important goal. … read more
Positive Attitude, Group Work, and Communication
Fostering a positive attitude toward mathematics is an important goal. This curriculum should lead students to conclude:
- Problems can almost always be solved in more than one way.
- Using various methods on the same problem should yield the same solution (or at least a close one).
- Solution methods should be explained, discussed, compared, and contrasted.
- Some problems are hard and may require more than a few minutes to solve.
- It is normal and acceptable to make mistakes.
- Working together is part of mathematics.
- Mathematics makes sense.
- Mathematics relates to life outside of school.
- Mathematics is fun.
This unit helps establish a classroom atmosphere that promotes collaborative work, engages students in discussion, and emphasizes the use of manipulatives. This atmosphere enables students to tackle challenging problems and generate many solution strategies. In the early units, we offer general suggestions for organizing student groups to promote collaborative learning.
As students work in groups to solve problems, they will talk and write about their methods and solutions—including their struggles and successes. Early explanations may be brief and poorly stated, but they will become more complete and sophisticated as students continue to talk and write about mathematics.
Resources
- Baroody, A.J. “Why Children Have Difficulties Mastering the Basic Number Combinations and How to Help Them.” Teaching Children Mathematics, 13 (1), pp. 22–31, August 2006.
- Kagan, Spencer. Cooperative Learning. Kagan Publishing, San Clemente, CA, 2008.
- National Council of Teachers of Mathematics, Principles and Standards for School Mathematics. NCTM, Reston, VA, 2000.
- National Research Council. “Chapter 4: The Strands of Mathematical Proficiency.” In Adding It Up: Helping Children Learn Mathematics, J. Kilpatrick, J. Swafford, and B. Findell, eds. National Academy Press, Washington, DC, 2001.
- Van de Walle, J.A., K.S. Karp, and J.M. Bay–Williams. Elementary and Middle School Mathematics: Teaching Developmentally, Eighth Edition. Pearson Education, Inc., Upper Saddle River, NJ, 2013.












