Lesson 2

Using Mental Math to Divide

Est. Class Sessions: 1

Developing the Lesson

Part 2: Dividing Using Mental Math

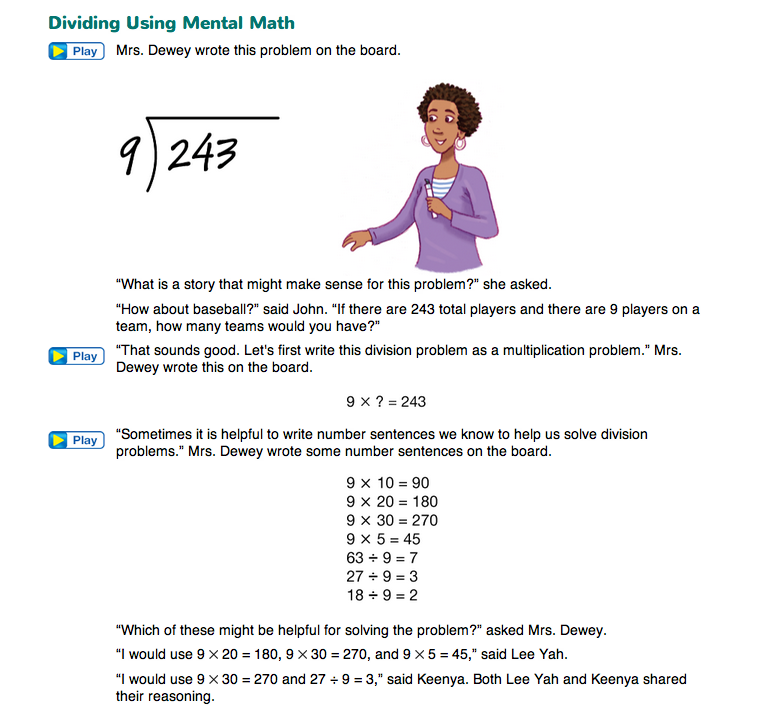
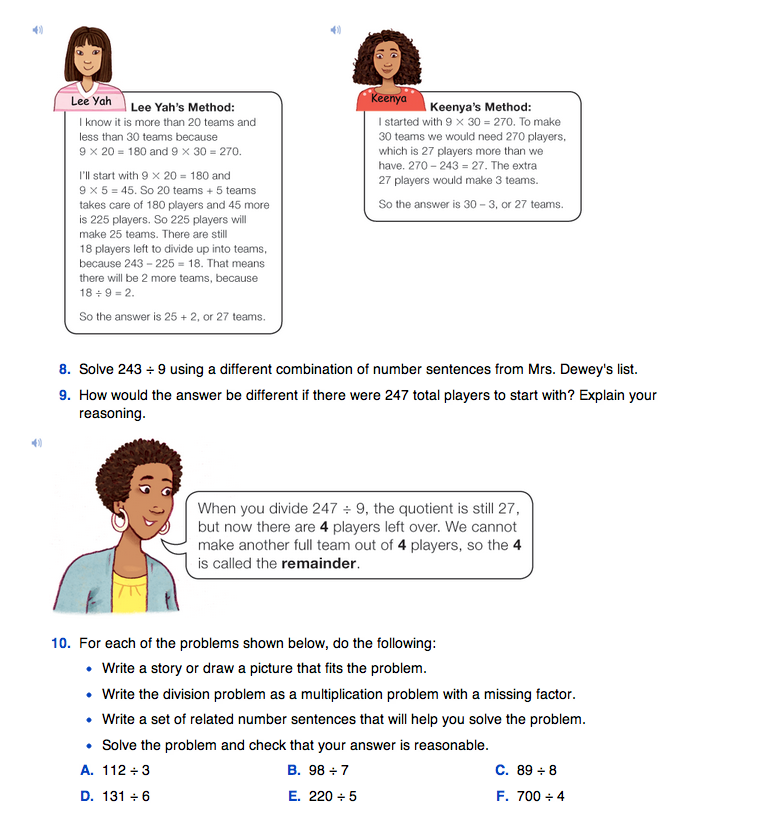
Begin this part of the lesson by reading and discussing the vignette in the Dividing Using Mental Math section in the Student Guide. Two sample solutions using a cluster of related number sentences are discussed for the problem 243 ÷ 9. Ask students to read through Lee Yah's and Keenya's strategies with a partner. Then ask them to explain one of the girl's strategies to their partners in their own words.

Write the following on the board.

392 ÷ 8

Have students work in pairs to write a story or situation that matches the division problem on the board. Choose one of the stories to use as the context for the problem.

The following is an example:

X
  • A large bookcase has 392 books in it, and there are 8 shelves in the bookcase. How many books are on each shelf if each holds the same number of books?

We will refer to this example throughout the discussion. The story you use may be different.

Ask:

X
  • How can this division problem be written as a multiplication problem? (8 × ? = 392)
  • What are some number sentences that can help you solve this problem mentally?

Write the number sentences on the board as students say them. If these number sentences do not come up, add them to the list:

  8 × 4 = 32
  8 × 5 = 40
  8 × 9 = 72
  8 × 40 = 320
  8 × 50 = 400
24 ÷ 8 = 3
48 ÷ 8 = 6
56 ÷ 8 = 7

Ask students to solve the problem with a partner using the number sentences on the list. Have each pair of students record their strategies. Again, have two or three pairs of students share their strategies with the whole class. The sample dialog shows a possible discussion with students as they explain their mental math strategies.

After students have practiced using related number sentences to solve the division problem posed, and the opportunity to discuss their mental math strategies, direct their attention back to the Student Guide. Have students work on Questions 8–9 which revisit the problem Mrs. Dewey posed of dividing players into teams.

X

Teacher: Who would like to share a mental math strategy for solving 392 ÷ 8? Which number sentence did you start with?

Irma: I started with 8 × 40 = 320. That means there are 40 books on each shelf to start out with. That's 320 books, and then I still have 72 books to divide, since 392 − 320 = 72. I had to subtract on paper. Is that okay?

Teacher: Sure, it's fine to make a few notes. Where did you go from there?

Irma: Well, 8 × 9 is 72. That means I can put 9 more books on each shelf and that takes care of all of the books. So the answer is the 40 on each shelf I started with, plus nine more. That's 49.

Teacher: Okay, that's a good strategy. Can someone share a different strategy to check if Irma's answer is reasonable?

John: Well, I don't think it's right, because I got a different answer.

Teacher: What answer did you get, John?

John: I got 42.

Teacher: Which number sentence did you start with?

John: I started with 8 × 50 = 400. Then I thought that 400 was too high by eight, since 400 − 392 = 8. So 8 less than 50 leaves 42.

Teacher: So you started with 8 × 50 = 400. So how many books are you putting on each shelf to begin with?

John: Um … 50.

Teacher: And how many books would that be in the whole bookcase?

John: Well, 400, but that's still eight too high.

Teacher: Okay, so how many books would you take off of each shelf to get to 392 total books in the bookcase?

John: One off each shelf. One less than 50 books would be—oh, I see. It would be 49 books on each shelf.

Teacher: So John and Irma both got the same answer using different ways to get there. Did anyone use a different way from John's or Irma's?…

In Question 8, students solve the problem using a different set of related facts. Question 9 relates to students' understanding of the remainder within the context of the problem. Dividing 247 total players into teams of 9 each results in the same quotient of 27 teams, but there is now a remainder of 4 players. Students could decide that 4 teams will have 10 players while the rest have 9 players.

Use the remainder that occurs in Question 9 to formally introduce the term remainder. Write the word on the board, explaining that when the leftover amount is less than the divisor, we cannot divide any further and still get a whole number answer. Use the word remainder in place of leftover throughout the rest of this unit so students become used to the term.

Student Guide: 532 533
Answers
X
SG_Mini
+
X
SG_Mini
+
Student Activity Book:
Master:
Sample rules for dividing with multiples of 10
X
+