Explore Multiplication by Multidigit Numbers
Est. Class Sessions: 2–3Developing the Lesson
Part 3. Two-Digit Multiplication Using Expanded Form
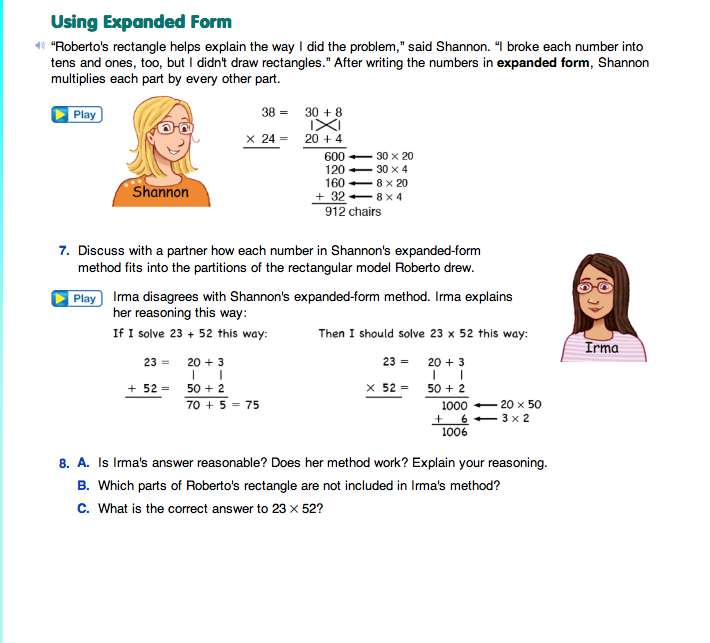
Direct Students to the using Expanded Form section of the Explore Multiplication by Multidigit Numbers pages in the Student Guide. In the opening vignette, Shannon makes a connection between Roberto's rectangle model and using expanded form to multiply. Ask students to read and study Shannon's expanded form strategy and then answer Question 7 with a partner.
In Question 8, students explore the differences between the expanded method for addition and the expanded method for multiplication. The rectangle model illustrates why every term is multiplied by every other term in multiplication (i.e., the distributive property of multiplication over addition).
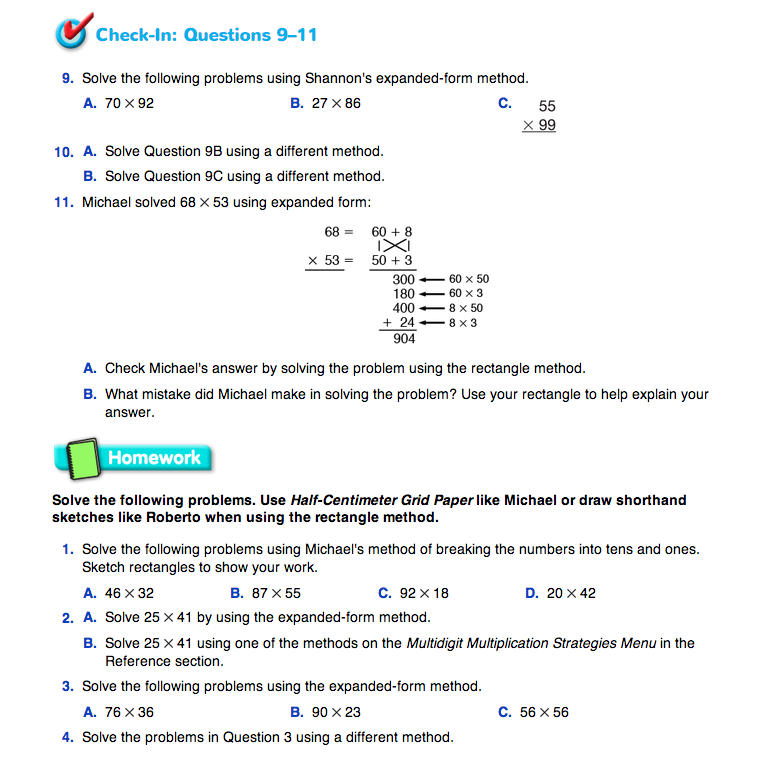
Assign Check-In: Questions 9–11. Students should complete these questions independently in order to check their understanding of multiplication strategies. Before the students begin their work, refer them to the Math Practices page in the Student Guide Reference section. For these problem solving questions, focus on Math Practices Expectations 2 and 4.
Ask students to discuss with their partner how they would apply the Math Practices to each of the problems.
Question 9 provides practice using the expanded form method. Students complete the lesson by solving Questions 10–11 in the Student Guide using multiple multiplication methods.