Conduct a Subtraction Strategy Session.
Begin the lesson by telling students they are going to have
a “strategy session” similar to the one for developing
addition strategies for larger numbers. Students will
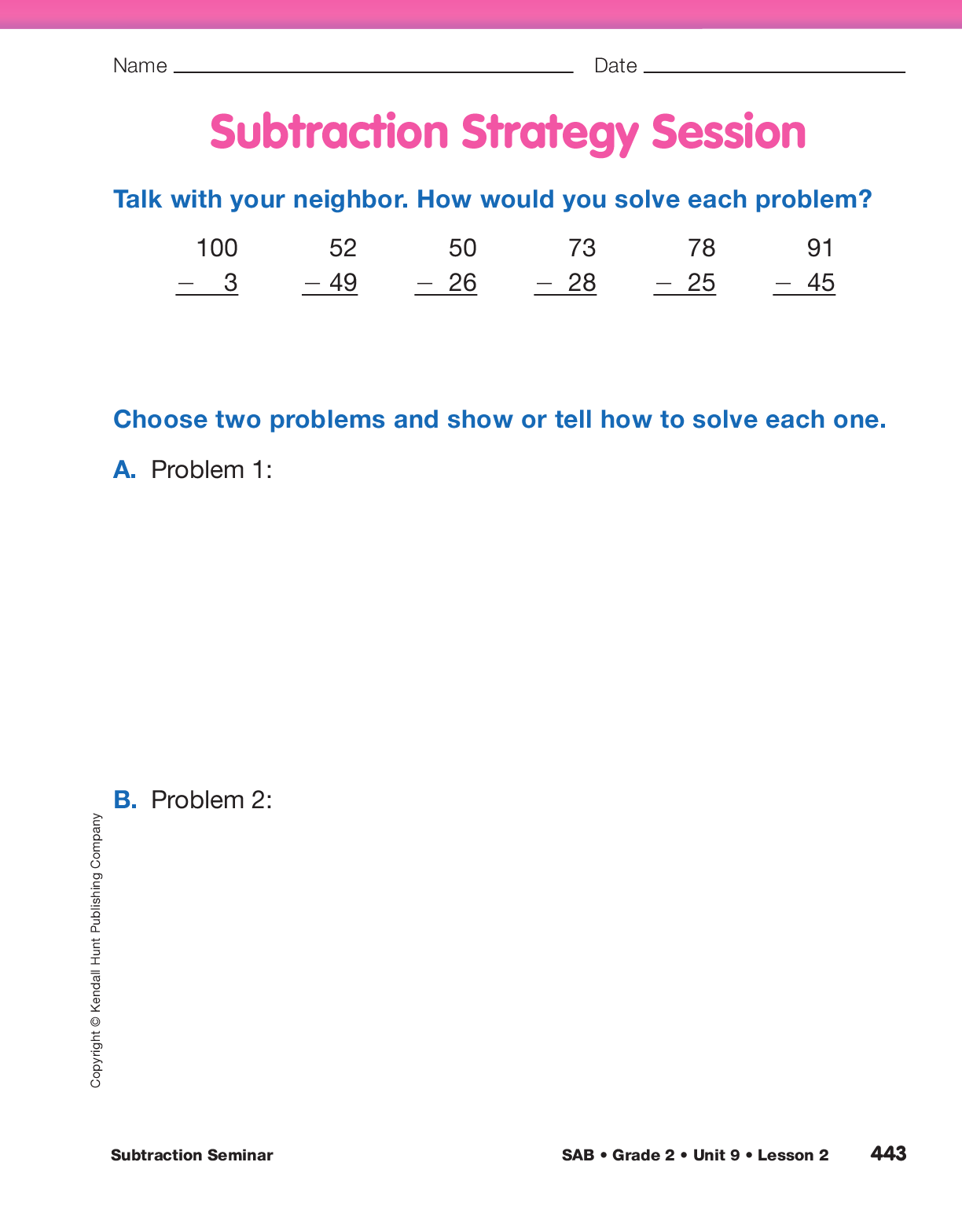
begin by looking at the problems on the Subtraction
Strategy Session page in the Student Activity Book
and discussing ways to solve each of the problems.
Introduce the problems and say:
- Discuss with your partner different strategies you
can use to solve each of the subtraction problems
on the page.
- Think of strategies that are efficient for each problem.
Depending on the problem, try to think of
strategies that are more efficient than counting up
or counting back.
- If you use tools such as the number line, 200
Chart, base-ten pieces, or connecting cubes,
explain how you use those tools to solve the problem.
Once student pairs have discussed strategies for
solving each problem, have them select two of the
subtraction problems and record their solution strategies
on the page. Explain that for each problem, they
should show or tell how they solved it and not just
name the tool they used. If students finish early,
encourage them to think of more than one way to
solve each problem.
Select one problem at a time and ask student volunteers
to demonstrate how they solved the problem.
Have the prepared
Number Line Display, the 200 Chart, base-ten pieces, and connecting cubes available
for students to use as they describe their subtraction
strategies.
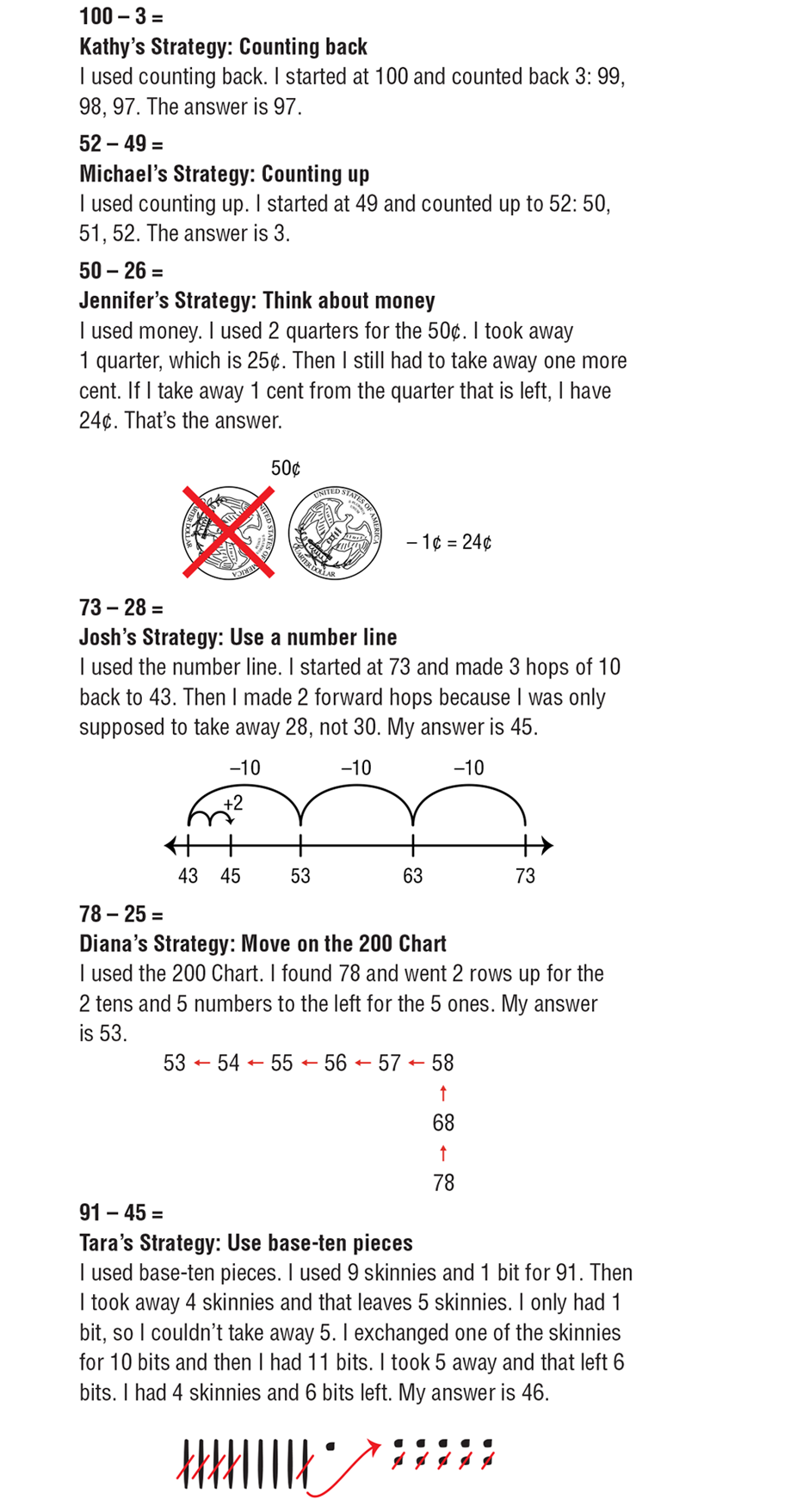
- Who can explain a strategy for solving the problem
100 − 3? (Possible response: I started at 100
and counted back 3: 99, 98, 97. The answer is 97.)
- Did someone solve it another way? (Possible
response: I wrote 1 hundred: 100. I had to
exchange the hundred for 10 tens, so I could take
away 3 ones. I took 3 away from one of the tens
and that left 7. I had 9 tens and 7 ones and that’s equal to 97.)
Develop Subtraction Strategies Chart. Once a student
has explained a strategy, identify it with that
student’s name and record the strategy on the
Subtraction Strategies Chart. See Materials
Preparation. As you continue discussing strategies
for other problems, ask students to solve the problem
using one of the named strategies on the chart. See
Figure 1 for a sample list of strategies.
Leave the Subtraction Strategies Chart posted for the rest of
the unit.
After students demonstrate their strategies and
record them on the chart, ask:
- Which strategies do you think are the easiest to
use? Why?
- Which strategies are the hardest to use? Why?
- Could you use different strategies for different
problems?
- Is there a strategy you don’t understand?
- Which strategies are the most efficient? Does it
depend on the problem?
Help students to understand that a strategy that is
efficient for one problem can be inefficient for
another. For example, using counting back by ones
is an efficient strategy for 100 − 3, but it is not an
efficient strategy for 52 − 49.

Math Trailblazers offers a range of strategies for students to
use in order to develop flexible thinking and strong number
sense. For some students a range of strategies to choose
from can be overwhelming. Students may not be able to
select s strategy that is efficient for a particular problem and
may give up instead. It is not necessary that every student be
equally fluent with every strategy. It may be helpful to narrow
the range to two or three strategies. Help students select
strategies that connect to their strengths and encourage
them to choose from those strategies when solving
problems.
Some students may simply be reluctant to abandon known
strategies, such as using counters to count all or count up.
However, these strategies are cumbersome and laborious for
two-digit addition and subtraction and are more likely to lead
to mistakes. At this point, students should be moving on to
more efficient strategies. Help students by selecting a
specific strategy that is understandable to them and ask them
to practice solving problems using that strategy. Once they
become comfortable with that strategy, they may respond to
encouragement to try another.