Lesson 2

Subtraction Seminar

Est. Class Sessions: 2–3

Developing the Lesson

Part 2: Emphasize Different Subtraction Strategies

Think about Subtraction. Display the Thinking about Subtraction Master and the Subtraction Strategies Chart. Tell students to imagine that they have a dollar or 100 cents to spend and they can choose one item to purchase. Ask a student volunteer to select an item and have students work in pairs to solve the problem using one of the strategies on the chart or any other strategy that makes sense to them.

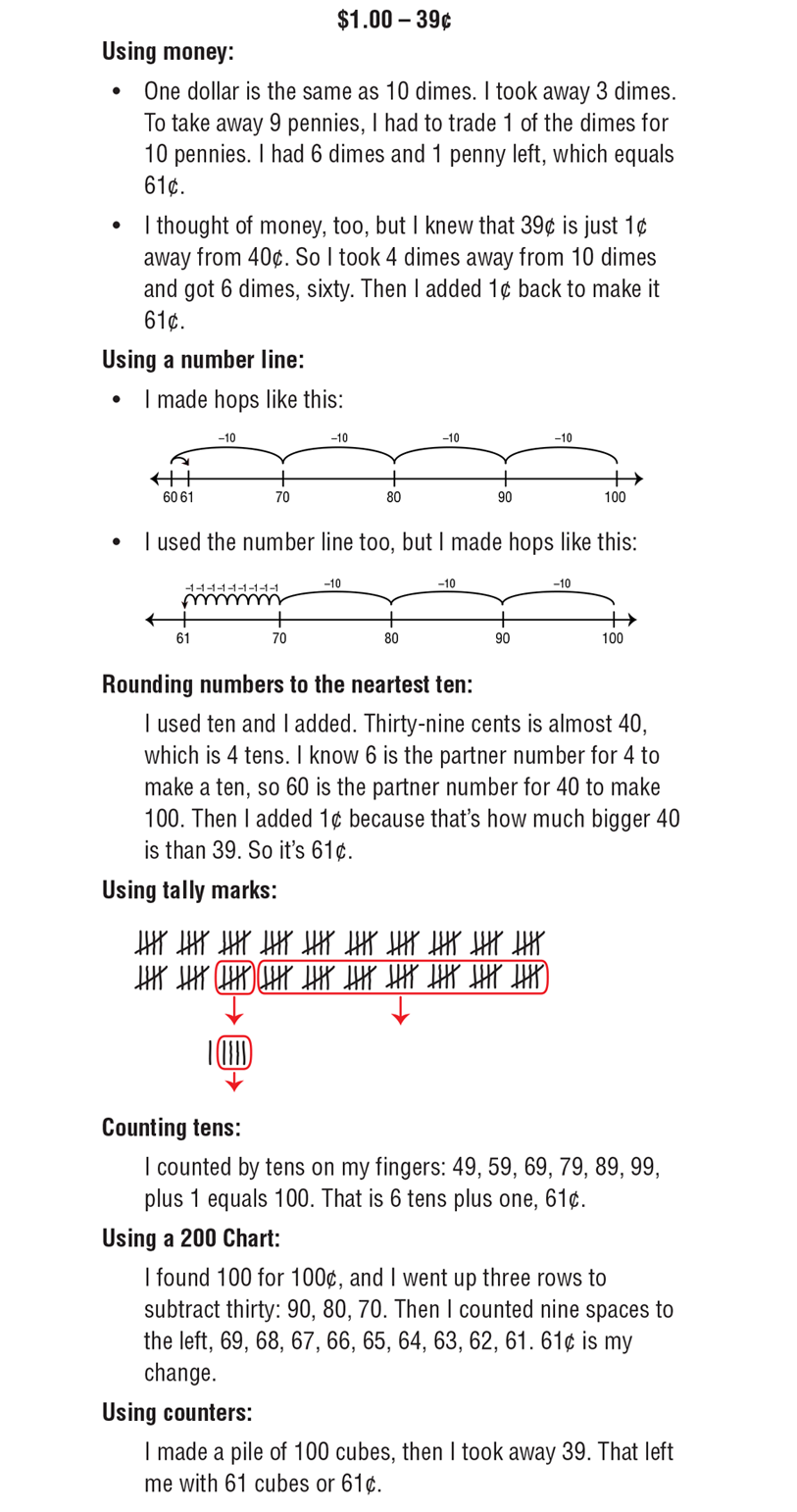
Use the speech balloons on the Master to record the thinking of individual students. Add any new strategies to the Subtraction Strategies Chart. Discuss whether each student’s answer is reasonable using estimation strategies. For example, to estimate the difference between $1.00 and 79¢, students might use the friendly numbers 100 − 80 to estimate a difference of 20. See Figure 2 for some solution strategies that might be suggested for buying a 39¢ item.

Have a student volunteer select another item to purchase on the Thinking about Subtraction Master and repeat the process. Encourage students to try a strategy from the chart that they have not used before. Add any new strategies to the chart.

Compare the strategies by asking questions similar to the following:

X
  • How is counting by tens like using the number line? (Possible response: We go up by tens in both strategies. When you start at 39 and count up to 99 that’s 6 tens and then add 1 more to get to 100. On the number line you can do the same thing.)
  • How are they different? (Possible response: I do one in my head and the other I do on the number line.)
  • How could you use coins to solve this problem? (Possible response: Think of 10 dimes and take away 4 dimes. That’s 6 dimes or 60¢. Add a penny because you took away 40¢ instead of 39¢. The answer is 61¢.)
  • Try solving the problem using a strategy you haven’t used before.

Try a Variety of Strategies. Encourage students to use the variety of strategies on the Subtraction Strategies Chart to solve these problems:

16 75 40 51
− 8 − 39 − 23 − 17

Instruct students to check each of their solutions by using a second strategy or by estimating. Use the Sample Dialog to guide your discussion of using efficient strategies and checking subtraction problems for reasonable answers.

X
Use this Sample Dialog to discuss using efficient strategies and checking for reasonable answers.

Teacher: What strategy did you use to solve 16 − 8?

Peter: I subtracted the ones first and then, subtracted the tens. For 16 − 8, I subtracted 6 − 8 = 2 and 1 − 0 = 1. My answer is 12.

Kayla: I got a different answer. For 16 − 8, I used doubles. I know that 8 + 8 = 16, so 16 − 8 = 8.

Peter: Oh, that’s right! I know my doubles. How did I get the wrong answer?

Teacher: Does 6 − 8 = 2?

Peter: No, I guess I can’t say the bottom number minus the top number because the top number is smaller.

Teacher: That’s right! That’s why we’re checking our answers to make sure they are reasonable. How did you solve 75 − 39?

Christina: For 75 − 39, I counted up from 39 to 75.

Teacher: Does anyone have a strategy that is more efficient?

Grace: I don’t think that counting up is an efficient strategy because it takes too long to count up to 75 if you start at 39. I think you should only use counting up by ones if the numbers are close.

Teacher: Good thinking! How did you solve the problem?

Grace: I counted back by tens. I started at 75 and counted back 4 tens: 65, 55, 45, 35 and then added one because I took away 40 instead of 39. My answer is 36. Counting back by tens is faster and more efficient than counting up by ones.

Teacher: What was your answer for 51 − 17?

Maria: I got 46 for an answer. I subtracted 1 − 7 = 6 in the ones column and 5 − 1 = 4 in the tens column.

Teacher: Did you check your solution by estimating or using another strategy?

Maria: I used friendly numbers. I said 51 is close to 50 and 17 is close to 20. 50 − 20 = 30. The answer should be close to 30.

Teacher: Is 46 close to 30?

Maria: No, but I don’t know what I did wrong.

Joseph: I got 34. If you look in the ones column, you can’t say 1 − 7 = 6. I used the number line. I started at 51 and went back 2 tens: 41, 31 and then I added 3 ones: 32, 33, 34 because I took away 20 instead of 17. My answer is 34.

Rosa: I got 34 for an answer, also. I started at 51 and took away 10 and I was at 41. Then, I went back another 7: 40, 39, 38, 37, 36, 35, 34. It’s also like using the 200 Chart because you can start at 51 and go up one line to 41 and go to the left 7 ones and your answer is 34.

Teacher: It’s important to use efficient strategies that are fast and accurate and to check your answers for reasonableness to make sure your answers make sense.

Adventure Book:
Student Activity Book:
Master:
Student explanations for subtracting $1.00 − 39¢
X
+