Use a class discussion to summarize the ideas of the
lesson. Ask student groups to write their number
sentences on the board and show the class the two
partitions that the number sentence represents. Use
prompts similar to the following that encourage students
to relate addition sentences with multiples of
ten
(20 + 80 = 100) to basic addition facts for ten
(2 + 8 = 10).
- Show us one of the two parts. How many groups
of ten are in your first part? (Responses will vary.
Possible response: 2 groups of ten)
- Show us the other part. How many groups of ten
are in your second part? (Responses will vary.
Possible response: 8 groups of ten)
- How many groups of ten were in the whole long
chain? (10 groups of ten)
- Do you still have all of the links? How can you be
sure? (Responses will vary. Possible response:
2 tens + 8 tens = 10 tens)
- How many links are in the first part? (Responses
will vary. Possible response: 20 links)
- Who would like to check this answer?
- How many links are in the second part?
(Responses will vary. Possible response:
80 links)
- Let's skip count by tens to check.
- What number sentence did you write that fits the
way you broke the chain? Why? (Responses will
vary. Possible response: 20 + 80 = 100; the first
part has 20 links and the other part has 80 links.)
Ask other groups to share their number sentences
and chains with the class and record the different
number sentences on the board.
If you notice that a
particular number sentence is missing, hold up the
partition, such as 3 groups of ten, and ask:
- What if one part had 7 groups of ten in it? How
many links would there be in that part? (70 links)
- I have the other part here in my hands. [Hold the
chain so that students cannot count the links.]
Talk with your partner. Decide how many links are
in this part of the chain.
- How did you decide? (Possible response: We
broke our 100-link chain after 7 tens. We counted
by tens with the second part. We counted to
30 links. So the number sentence is
70 + 30 = 100.)
- Is there another way to find the answer? (Possible
response: We started at 70 and counted 80, 90,
100. That's three more tens, so 30. Or, we knew
that
7 + 3 = 10, so 70 + 30 = 100.)
- What number sentence fits the way this chain is
broken? (70 + 30 = 100)
While students share their solutions, a discussion
may arise as to whether two number sentences such
as 20 + 80 = 100 and
80 + 20 = 100 are the same
or different solutions. Ask individuals for their
opinions. Have these students explain why they
consider the sentences different or the same. Remind
students of the turn-around rule. Then come to a
class agreement.
To conclude, ask students questions such as:
- What is the same about these number sentences? (They add up to 100. They have two parts.)
- What is the same about all of our partitions?
(They have groups of ten in them. The two parts
add up to 100.)
- If I have 1 group of ten and 9 groups of ten, how
many links do I have in each part? (10 links and
90 links)
- What number sentences describe this partition?
(1 group of ten + 9 groups of ten = 10 groups of
ten; 10 links + 90 links = 100 links)
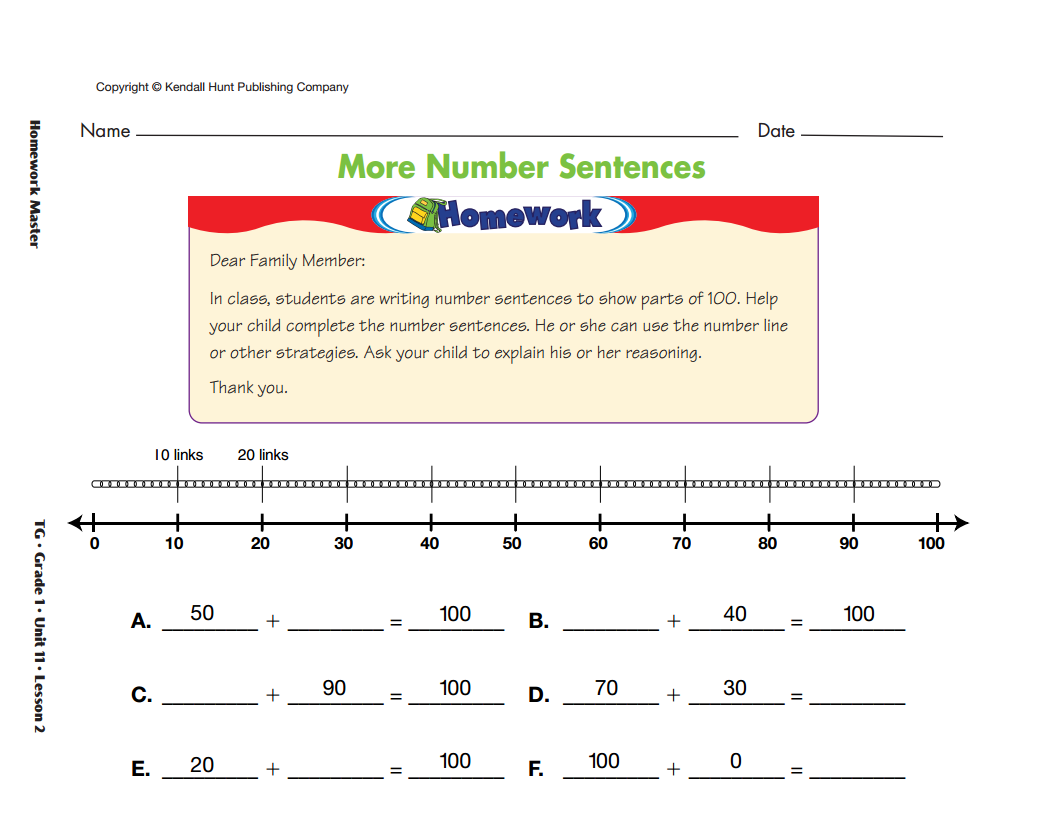
Assign the More Number Sentences Homework
Master. Students complete number sentences with
missing addends. Introduce the page using the first
number sentence as an example.
- What do you notice about the sums written in the blanks? (They are all 100.)
- Look at the first problem: 50 plus some number
equals 100. How can you solve it? (Possible
response: I think about links. There are 50 links
so I start there and count up by tens: 60, 70, 80,
90, 100. I counted 5 more tens, so it's 50.)
- How can you use the number line on the page to
complete the number sentence? Show us on the
class number line. (I start at 50 and hop 5 times:
60, 70, 80, 90, 100. 50 + 50 = 100.)
- Is there another way to solve this problem?
(Possible response: I thought about 5 + 5 = 10.
5 tens plus 5 tens is 10 tens and that is the same
as 50 plus 50 equals 100.)