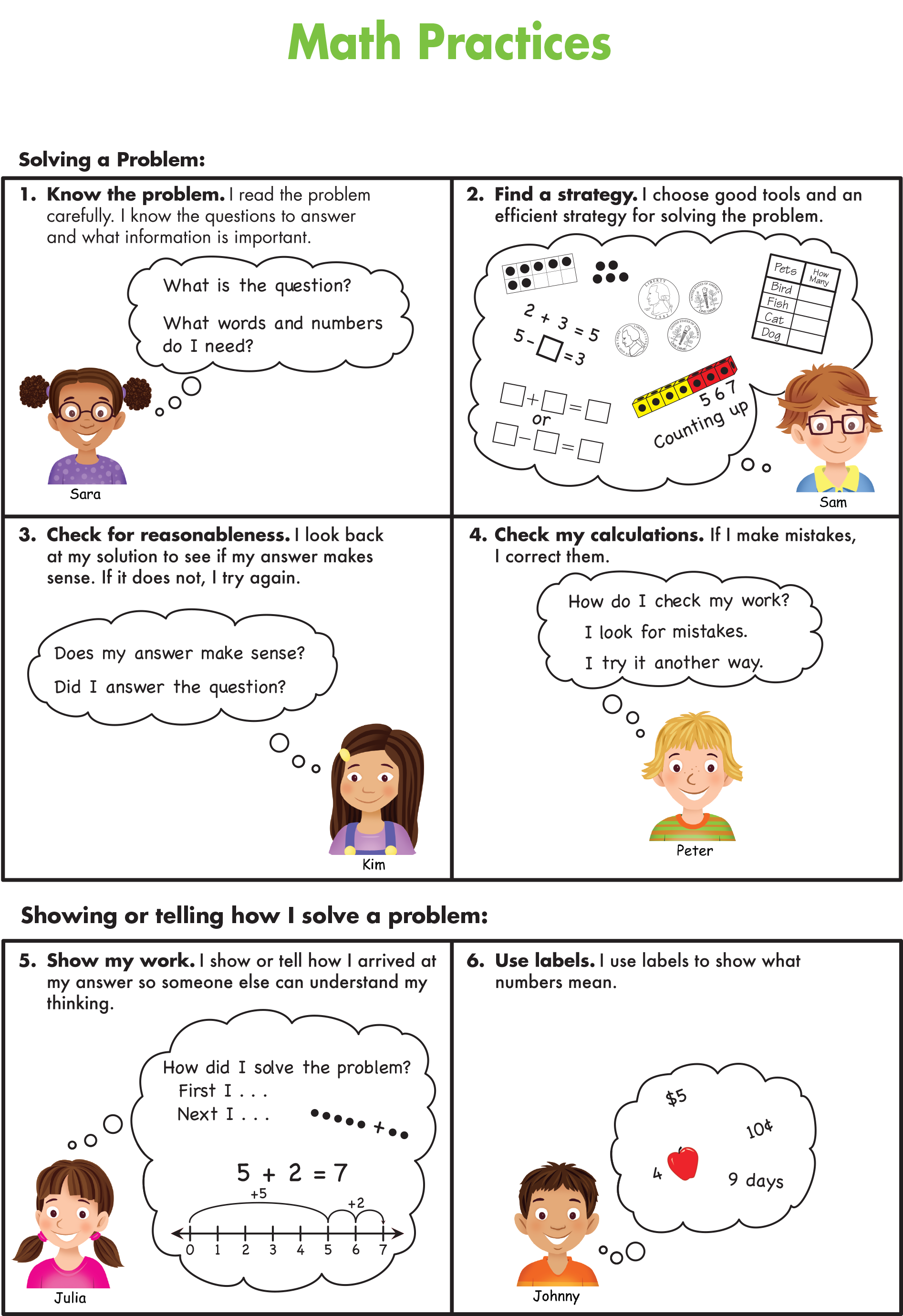
Use Math Practices to Solve Problems. Remind
students that in the last lesson they counted bags of
marbles by tens and twenties and solved addition
and subtraction problems. In this lesson, they will
solve different problem types using the Math
Practices. See Content Note.
It's important that students encounter a range of different
kinds of problems. The work of Thomas Carpenter and his
colleagues with the Cognitively Guided Instruction Project
(CGI) provides insights that can help teachers with this
process (Carpenter, et al., 1999). They devised a
classification scheme for problems most adults would
solve by addition, subtraction, multiplication, or division.
CGI classifies the addition and subtraction situations into
11 types of problems.
Display the chart with the three problems you prepared
prior to the lesson. See Materials Preparation.
Display and direct students' attention to the Math
Practices page in the Student Activity Book. Have
number lines, connecting cubes, and the 100 Chart
in the Student Activity Book Reference section readily
available.
Read Question 1 on the chart aloud and then ask:
- For Math Practice 1, Know the problem, you have
to know what the question is asking us to do and
what information is important to solve the problem.
What do you know? (Possible response: We
know Maria had 40 marbles. We don't know how
many she found, but we know she had 60 marbles
at the end. We have to find how many marbles
she found.)
- For Math Practice 2, Find a strategy, you have to
find a strategy. What strategy and what tools
should you choose? (Possible response: I think
we need to add the number she started with and
add more until we get to 60. I would use
connecting cubes.)
- For Math Practice 5, Show my work, you have to
show your work. How would you do this?
(Possible response: I start with 40 connecting
cubes. I add another train of 10 to make 50, and
then another train of 10 and I get 60. The two
trains of ten cubes means that she found
20 marbles.)
- What is a number sentence for this problem?
(Possible response: I knew it was 40 + = 60.
I added 20, so the number sentence is
40 + 20 = 60.)
- For Math Practice 3, Check for reasonableness,
you have to check for reasonableness or make
sure your answer makes sense. Does this solution
make sense? How do you know? (Yes, because if
you add 40 + 20, you will get 60. I know
4 + 2 = 6, so 4 tens plus 2 tens will be 6 tens or
60.)
Read Question 2 on the chart. In this question, the
start is unknown but students know the change and
the result. Follow the same procedure using the
Math Practices page, focusing students' attention on
Math Practice 1, Know the problem; Math Practice 2, Find a strategy;
and Math Practice 5, Show your work.
Question 3 on the chart is a multi-step problem. Read Question 3 and ask:
- What do you need to find out [MPE1]? (how many
marbles are left)
- What is the important information in the problem?
(Possible response: Tony had 60 marbles. First,
he sold 40 marbles and then he sold 10 more.)
- What strategy can you use to solve the problem
[MPE2]? How do you know? (Possible response:
subtraction; If Tony is selling marbles, he will
have less at the end.)
- What is a number sentence for the problem?
(Possible response: 60 − 40 − 10 = 10, or
60 − 40 = 20 and then 20 − 10 = 10)
- How would you show your work [MPE5]? (Possible
response: I would use the 100 Chart. Start at 60
and go back 4 rows to 20 and then go back one
more row to 10.)
- Does the answer make sense [MPE3]? (Possible
response: Yes, I know I have to have less in the
end because Tony sold marbles twice. First he
sold 40 and then he sold 10. If I think of it as
6 tens − 4 tens − 1 ten, it's like 6 − 4 − 1 and that
equals 1, so the answer is
1 ten or 10.)
- Solve this problem another way.
If students do not suggest it, share the following
solution, displaying the number sentences. First add
the two amounts Tony sold: 40 + 10 = 50. Then subtract
60 − 50 = 10. This may be confusing to students
because they have to add before they subtract. See
Content Note. Demonstrate the problem by starting
with 6 trains of 10 cubes. Put 4 trains together with
1 train and then take them away. To verify the solution,
start again with 6 trains of ten cubes, take away
4 trains of ten cubes and then 1 train of ten cubes.
- How many did I take away altogether?
(40 + 10 = 50)
- Why did I add 40 + 10? (That was the number of marbles that Tony sold.)
- Does this solution make sense? How can you
check it? (Possible response: I could solve it
another way. Start at 60 on the class number line.
Hop back 40 to 20, and then hop back 10 to 10.
The solution makes sense.)
Fostering a positive attitude toward mathematics is an
important goal and this curriculum should lead students to
conclude:
- Problems can almost always be solved in more than one way.
- Using various methods on the same problem should yield the same solution (or at least a close one).
- Solution methods should be explained, discussed, compared, and contrasted.
- Some problems are hard and may require more than a few minutes to solve.
- It is both normal and acceptable to make mistakes.
- Mathematics makes sense.
Solve Addition and Subtraction Problems. Next,
introduce the problems on the Adding and
Subtracting Bags of Marbles pages in the Student
Activity Book and have students work in pairs to
complete Questions 1–6. Have connecting cubes,
100 Charts, and number lines readily available.
Remind students to keep the display of the Math
Practices page in mind as they solve their problems.
Emphasize that for MPE3, students should check for
reasonableness, and if their answer does not make
sense, they should try again. Monitor students as
they work and look for the use of a variety of strategies.
The following questions can be used both while
students work as well as upon completion to discuss
solution strategies:
- What information was important in this problem?
- Was there any extra information?
- Did you add or subtract to find this answer?
- Show or tell how you solved the problem in Question [number].
- Did someone solve this problem another way?
- How can you check to make sure this answer is reasonable?
- Did you use any tools to solve Question [number]?
- Explain what the numbers in your number sentence mean.
See the Sample Dialog for a sample discussion of Question 5.

|
Use this sample dialog to discuss Question 5 on the Adding and Subtracting Bags of Marbles page.
Teacher: Tony had 70 red marbles in seven bags of 10. He
sold 40 marbles. Then he found 20 more marbles in a
box. How many marbles does he have now? What
information in the problem is important and what is the
question we have to answer?
Julia: I see the numbers 70, 7, 10, 40, and 20. I think those are
the important numbers I need to solve the problem. We
have to find out how many marbles Tony has at the end of
the problem.
Teacher: Does everyone agree?
Faith: I don't think we need to use all those numbers. There
were 70 marbles in 7 bags of 10. The numbers 7 and 10
just describe how the marbles are placed in bags: 7 bags
of 10—that's the same as 70. We don't have to use the 7
and 10 to solve the problem.
Teacher: Great answer, Faith! It's important to figure out
which numbers you need to solve the problem. Now we
know which numbers are important and the question we
have to answer. Who can tell me how you solved the
problem?
|
Brandon: Tony had 70 marbles and sold 40. Then he found 20
more. My number sentence is 70 − 40 − 20 = 10.
Teacher: Does someone have a different solution? Is the
answer reasonable?
Sarah: That doesn't seem reasonable. If Tony started with 70
and he sold 40, he would have 30 left. Then he found 20
more marbles. He should have more than 30, not less! I
think he should have 50 marbles at the end. My number
sentence is 70 − 40 + 20 = 50.
Teacher: Sarah, show or tell us how you solved the problem.
Sarah: I used the 100 Chart. I found 70 and went back 40 and I
was at 30. Then I had to add 20, so I moved from 30 to 40
to 50. My answer is 50.
Teacher: Great job, Sarah! When we have a problem with
more than one step, we have to solve one step at a time.
First Sarah found 70 − 40 = 30 and then she said
30 + 20 = 50.
|
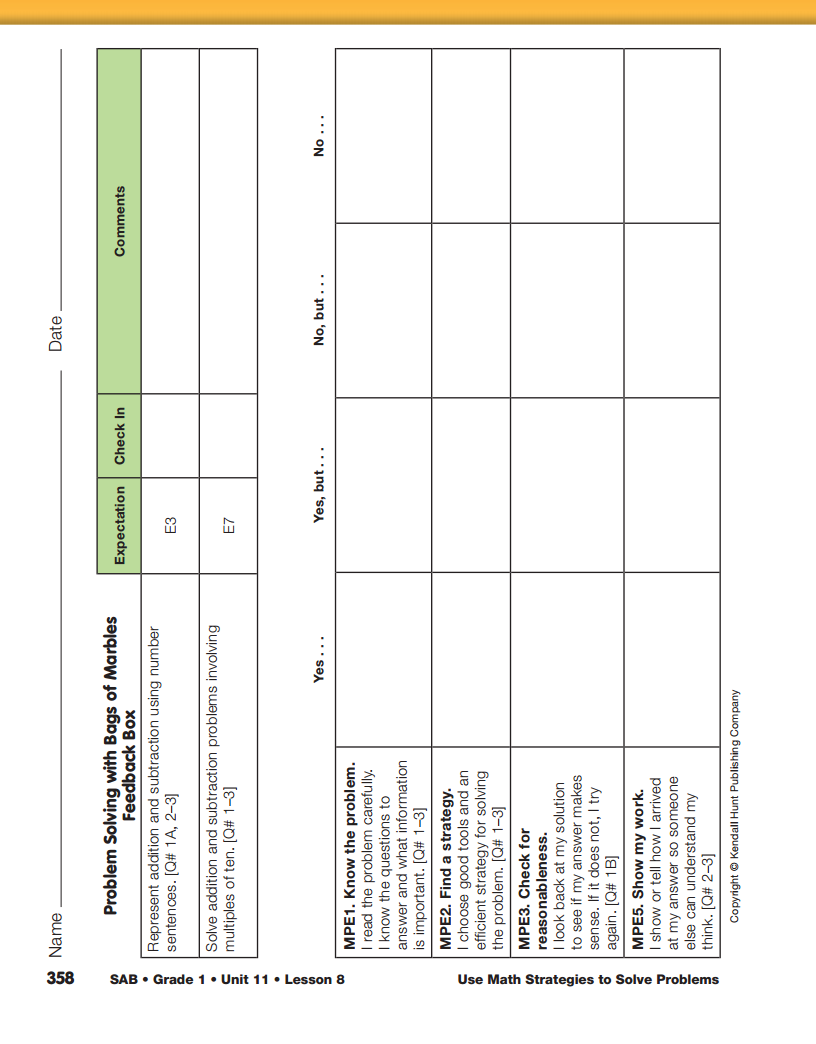
After students have had time to discuss solution
strategies, assign the Problem Solving with Bags of
Marbles page in the Student Activity Book to assess
students' abilities to solve problems using efficient
math practices.
Use the Problem Solving with Bags of Marbles with the
Feedback Box in the Student Activity Book to assess
students' abilities to represent addition and subtraction
situations using number sentences [E3]; solve addition and
subtraction problems involving multiples of ten [E7]; know
what is important to solve a problem [MPE1]; find addition
and subtraction strategies to solve problems [MPE2]; check
for reasonableness [MPE3]; and show how to solve
problems [MPE5].