For Question 3, students estimate the distance between two objects by measuring the distance on the map and using the scale. For example, on the data table in Figure 2, object A is the wastebasket and object B is Mr. Moreno's desk. Measuring on our map in Figure 3, the distance between object A and object B is 4.7 cm. Direct students to measure the distance between object A and object B on their map using a centimeter ruler.
- How can you use the measured distance to estimate the actual distance? (Possible response: Use the scale on the map. The scale on my map is 1 cm = 100 cm, so I should multiply the measured distance by 100 to find the actual distance.)
- What is the measured distance between object A and object B on your map?
- What is the estimated distance between object A and object B?
- Show how you know? (Possible response: I multiplied 4.7 × 100 on the calculator and got 470 cm.)
- How can you check if your estimate is correct? (Possible response: Measure the actual distance.)
Ask two students to use metersticks to measure the actual distance between objects A and B to check the calculations.
Error or Mistake. Students need to compare their predicted distance from the map to the actual distance in the classroom to see if they were close in their predictions. They need to find the difference between the two measurements. This is called error.
In mathematics, we make a distinction between “error” and “mistake.” In the dictionary these words may be used interchangeably, but in this context, “error” has a different meaning. In our case, “error” means the difference between an estimated result and the actual measured result.
In Question 4, students can either choose two objects or you can assign different pairs of objects to student groups. After students have had a chance to estimate the distance between the two objects and check their estimate by measuring the actual distance, ask students to share their work with another group of students.
While students are working circulate and ask:
- How did you estimate the distance?
- Show or tell how you estimated the distance.
- What was the actual distance between the objects?
- Was your prediction close?
How Close is Close Enough. How can we help students with this concept? Using 10% as a benchmark for closeness is appropriate for this age level. Students can find 10% of a number by dropping the last digit (e.g. 10% of 183 is 18 so an estimate of 165–201 is considered close). If students are off 12 cm from a prediction of 15 cm, we would say that is not close.
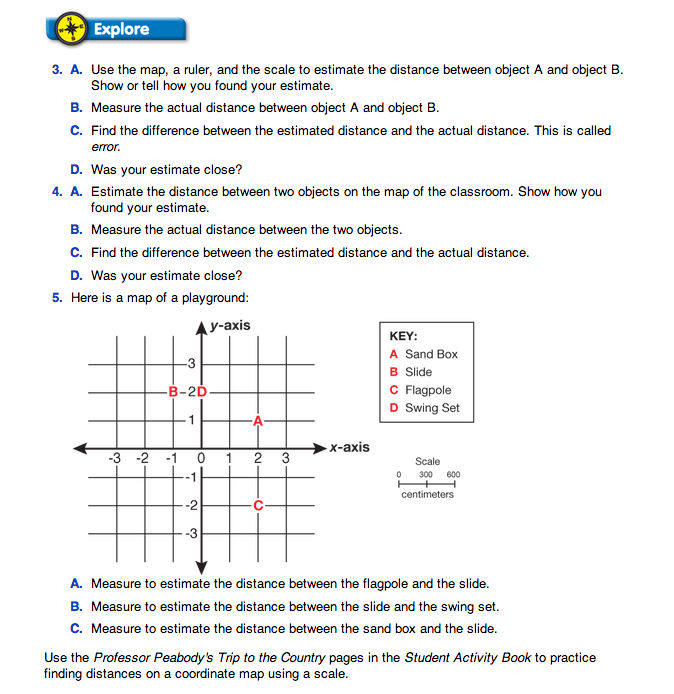
As students complete Question 4, ask students to complete Question 5. In Question 5 students use a scaled coordinate map to estimate the distances between objects on a playground.
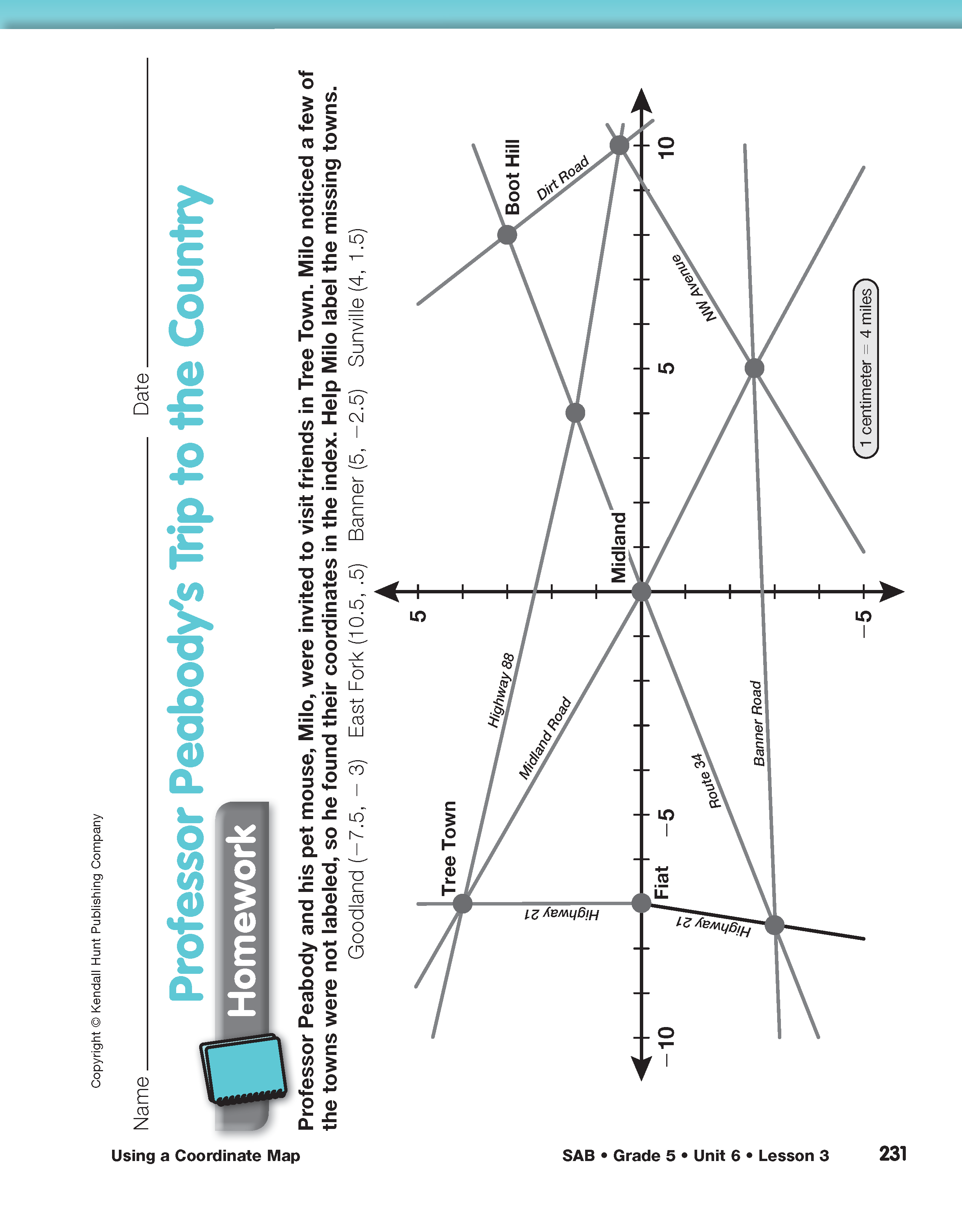
For additional practice in using a scale map to find distance, assign the Professor Peabody's Trip to the Country pages in the Student Activity Book for homework.