Location and Shapes
“Given children's affinity toward, knowledge of, and ability to gain geometric knowledge, it is important that this domain of mathematics not be neglected. Instruction in geometry needs to complement the study of number and operation in grades pre-K to 8” (National Research Council, 2001). Math Trailblazers echoes this expectation by emphasizing the importance of geometry in the mathematics curriculum. This unit represents the focal point of geometry in Fifth Grade. Students explore the nature of two-dimensional geometric elements including lines, angles, and polygons. Students also discover relationships within and among these elements as they advance their understanding through stages, from basic intuition to analysis and informal deduction.
van Hiele Levels of Geometric Development

Much of the approach to geometry found in the Math Trailblazers curriculum is grounded… read more
Much of the approach to geometry found in the Math Trailblazers curriculum is grounded in the insights of Dutch educators Pierre van Hiele and Dina van Hiele-Geldof. Ongoing research in mathematics education continues to confirm the five levels of geometric development first described by the van Hieles in the 1950s (Burger and Shaughnessy, 1986). The five levels are:
Level 0: Visualization. Students judge geometric objects by their appearance, but not by attributes. For example, a student can identify a rectangle because it “looks like a rectangle,” but not because it has opposite sides equal and four right angles.
Level 1: Analysis. Students begin to describe the properties of objects. A figure is no longer judged because it “looks like one,” but rather because it has certain properties. For example, an equilateral triangle has three equal sides, three equal angles, and line symmetry.
Level 2: Informal Deduction. Students logically order the properties of figures and are able to deduce that one property precedes or follows from another property. They see relationships among figures. For example, a square has all the properties of a rectangle; therefore, a square is a rectangle. Students may also be able to define a square based on its properties.
Level 3: Deduction. Students write formal proofs based in an axiomatic system. A rigorous high school geometry course is taught at level 3.
Level 4: Rigor. Students can work with different axiomatic systems. This level corresponds to college work in geometry (Crowley, 1987; van Hiele, 1999).
The van Hieles found that each level, while not age specific, builds on the previous level. Students proceed from level to level sequentially and no level can be omitted. Advancement depends on content and method of instruction (Crowley, 1987; van Hiele, 1999). Moreover, a student's experiences with lower-level reasoning at the elementary school level are critical to success with geometry in later schooling. Students who are at level 0 or 1 when entering high school geometry have a poor chance of success. Students who begin high school geometry at level 2 have at least a 50% chance at succeeding (Senk, 1989). Unfortunately, many upper elementary students are still at level 0. This is not surprising, as researchers have found that most geometry questions asked in standard elementary math textbooks were answerable with level 0 understanding (Fuys, Geddes, and Tischler, 1988).
Analyzing Shapes
In previous grades, students focused on developing the concepts and vocabulary needed to describe… read more
Analyzing Shapes
In previous grades, students focused on developing the concepts and vocabulary needed to describe two-dimensional and three-dimensional shapes (level 1). In this unit, students will use those descriptions and shape properties to sort and classify shapes (level 2). For example, in Grade 4 Math Trailblazers students learned how to identify an acute, obtuse, and right angle and find those angles in polygons. They also analyzed the sum of the interior angles of a triangle. In this unit, students will classify triangles using these angle descriptions. Students then use deduction to decide if a right triangle can also be classified as an equilateral triangle. Students also analyze the ordered pairs of shapes plotted on a coordinate grid to decide if the angle is a right angle. In Lesson 6, students use a more deductive approach (level 2) to classify polygons. Classification builds on the idea of inclusion. For example, since a square is a quadrilateral with four right angles, a square is a rectangle.
A WORD on VOCABULARY

This unit contains a great deal of mathematical vocabulary. Encourage students to use mathematical… read more
This unit contains a great deal of mathematical vocabulary. Encourage students to use mathematical words that precisely and accurately express their thoughts. However, keep in mind that students remember definitions with greater meaning when they learn them through discourse. Direct memorization is generally far less productive than using words in context, since words learned by rote are quickly forgotten. A better strategy is to encourage students to discuss their work using their language and then model geometers' terminology to extend the discussion.
Etymology, or the study of words, teaches us that words often do not have an exact, single meaning. Word meanings evolve over time, and words may be defined differently among different groups or individuals. This is even true in mathematics. For example, in a study of school geometry texts, the word “quadrilateral” was found to have seven different definitions (Usiskin, 2008). Although each of these definitions includes the same set of shapes, each emphasizes something different about quadrilaterals. When considering the word “trapezoid,” we even find two definitions in textbooks that include different sets of shapes. One definition includes quadrilaterals with only one pair of parallel sides; another with at least one pair of parallel sides. In the end, the class must agree to settle on a particular definition, but we should do so knowing mathematicians sometime disagree. See Figure 1.
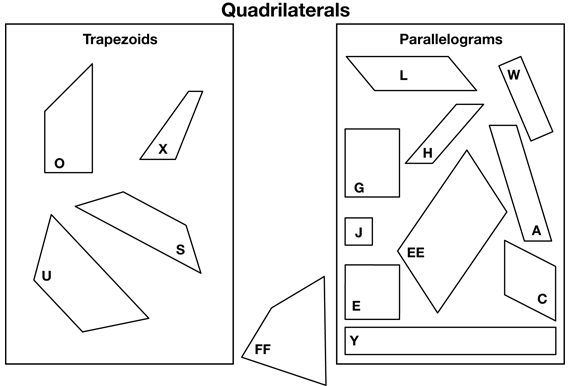
Figure 1: Quadrilaterals sorted as trapezoids and parallelograms
LOCATION and DISTANCE

In this unit, students are also introduced to negative numbers within real-world contexts, including… read more
In this unit, students are also introduced to negative numbers within real-world contexts, including measuring temperature and depth. Then, positive and negative numbers are applied to the task of making a number line and then a coordinate grid.

Figure 2: Mr. Origin
Students locate objects relative to a plastic figure called Mr. Origin. Mr. Origin is based on a figure invented by Robert Karplus, a physicist who became interested in elementary education and spent a year in Geneva studying with the Swiss psychologist Jean Piaget. In earlier grades activities on mapping and coordinates, Mr. Origin was positioned on a line or at the intersection of two lines to represent the origin. Students described the location of objects in relation to Mr. Origin using terms like front, back, left, and right. They also measured the distance between objects along those lines.
In this unit, students describe the location of objects as points on a coordinate grid with Mr. Origin at the origin. Students learn to describe these locations with ordered pairs in all four quadrants (e.g., right, left, front, back). Locating objects in this way is now known as using Cartesian coordinates (in honor of Descartes). Mathematicians generally label the horizontal (right and left) axis the x-axis and label the vertical (front and back) axis the y-axis. This terminology is used in this unit.
There are several major ideas in this lesson on coordinates. First, the notion that directions (right, left, back, front) depend upon choosing a reference point, the origin. Second, the location of an object can be specified by giving two distances with their directions (the coordinates or the ordered pair). Third, these coordinates can be plotted on graph paper to make a map. Finally, measurements on the map are usually not the same as distances in the real world. Students use the map scale to estimate the distances on a map. This is an opportunity to review and connect what students learned about ratios in Unit 5.
In Lesson 5, students use coordinates for another purpose: to draw polygons. As they do this they analyze how sets of coordinates make polygons with certain properties. For example, which set of coordinates will make a square? In these experiences, students revisit concepts, descriptions, and properties of polygons, particularly quadrilaterals and triangles.
MATH FACTS and MENTAL MATH

This unit continues the review and assessment of the multiplication and division facts to… read more
This unit continues the review and assessment of the multiplication and division facts to develop mental math strategies, gain proficiency, and to learn to apply multiplication strategies to larger numbers. Students will focus on the multiplication and division facts for the last six facts.
Resources
- Burger, W., and J.M. Shaughnessy. “Characterizing the van Hiele Levels of Development in Geometry.” Journal for Research in Mathematics Education, 17, pp. 31–48, National Council of Teachers of Mathematics, Reston, VA, 1986.
- Clements, D., and J. Sarama. Learning and Teaching Early Math: The Learning Trajectories Approach. Routledge, New York, 2009.
- Crowley, M. L. “The van Hiele Model of the Development of Geometric Thought.” Learning and Teaching Geometry, K–12. 1987 Yearbook. Mary Montgomery Lindquist, (Ed.). National Council of Teachers of Mathematics, Reston, VA, 1987.
- Fuys, D., D. Geddes, and R. Tischler. “The van Hiele Model of Thinking in Geometry Among Adolescents.” Journal for Research in Mathematics Education, Monograph Number 3. National Council of Teachers of Mathematics, Reston, VA, 1988.
- National Research Council. “Developing Mathematical Proficiency Beyond Number.” In Adding It Up: Helping Children Learn Mathematics. J. Kilpatrick, J. Swafford, and B. Findell, (Eds.). National Academy Press, Washington, DC, 2001.
- Senk, S. L. “Van Hiele Levels and Achievement in Writing Geometry Proofs.” Journal for Research in Mathematics Education, vol 20, no. 3, 309–321. National Council of Teachers of Mathematics, Reston, VA, 1989.
- Usiskin, Z. The Classification of Quadrilaterals: A Study in Definition. Information Age Publishing, Charlotte, NC, 2008.
- van Hiele, Pierre M. “Developing Geometric Thinking through Activities That Begin with Play.” Teaching Children Mathematics, vol 6, February, 310–316. National Council of Teachers of Mathematics, Reston, VA, 1999.
- Wilson, P. R., and A. Osborne. “Foundational Ideas in Teaching about Measure.” Teaching Mathematics in Grades K–8, Research-Based Methods. Thomas R. Post, (Ed.). Allyn and Bacon, Inc., Boston, 1988.











