Fraction Sums and Differences

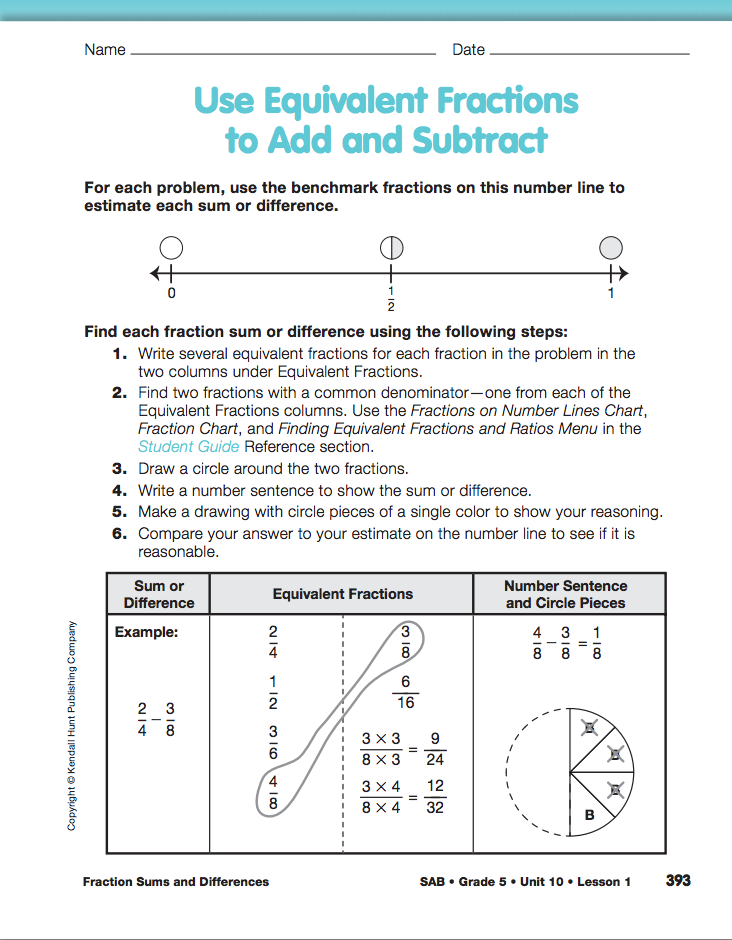
Find Common Denominators. Tell students that they will learn a numerical way to add and subtract fractions with unlike denominators. They will use their fraction circle pieces to help them understand why this method makes sense. Ask students to refer to the Use Equivalent Fractions to Add and Subtract pages in the Student Activity Book. This activity is designed to help students progress toward the more formalized method for finding common denominators that will be presented in Lesson 2. Read through the instructions and the first example together with the class.

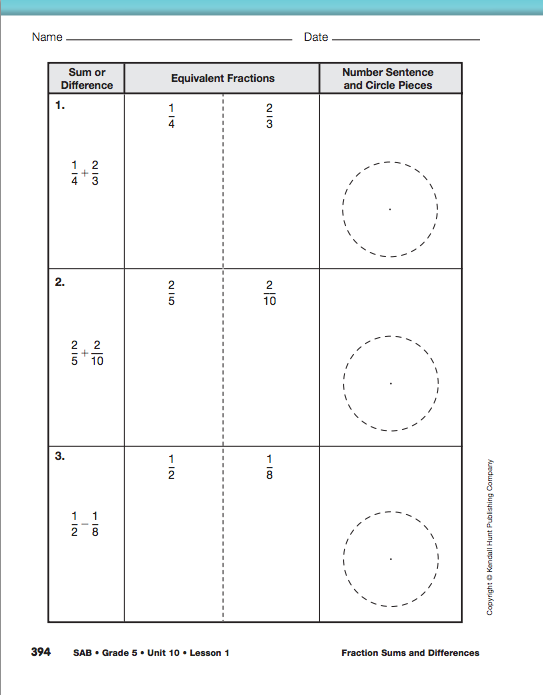
Students work with partners to solve addition and subtraction problems for fractions with unlike denominators using the common denominator method demonstrated by Jessie in the Sample Dialog. Using this method, they explore a trial-and-error approach to finding common denominators. After estimating the answer, they list equivalent fractions for both fractions in the sum or difference and inspect the sets of fractions for a common denominator. They can then easily add or subtract the numerators of fractions with like denominators.

After finding the sum or difference using a common denominator, students represent the addition or subtraction problem with circle pieces. Have students first show the original sum or difference using circle pieces. Then, using the one-color method, have them replace the pieces with a single color to model the finding of a common denominator. An example is shown in Figure 6.

Remind students to check their calculations by comparing their answers to their estimates on the benchmark fraction number line.

Circulate as students complete the addition and subtraction problems.

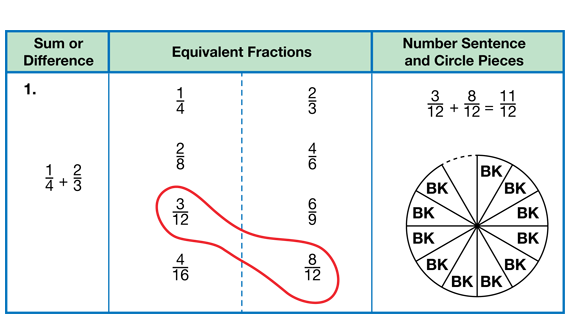
After student pairs have completed two or three of the problems, ask one pair of students to use a display of the Use Equivalent Fractions to Add and Subtract pages to show how they solved Question 1. First ask how they estimated using the benchmark fraction number line. They should write the equivalent fractions they found for each addend and circle the fractions that share a common denominator. Ask them to show how they found the sum using circle pieces of a single color using display fraction circle pieces. See Figure 7.

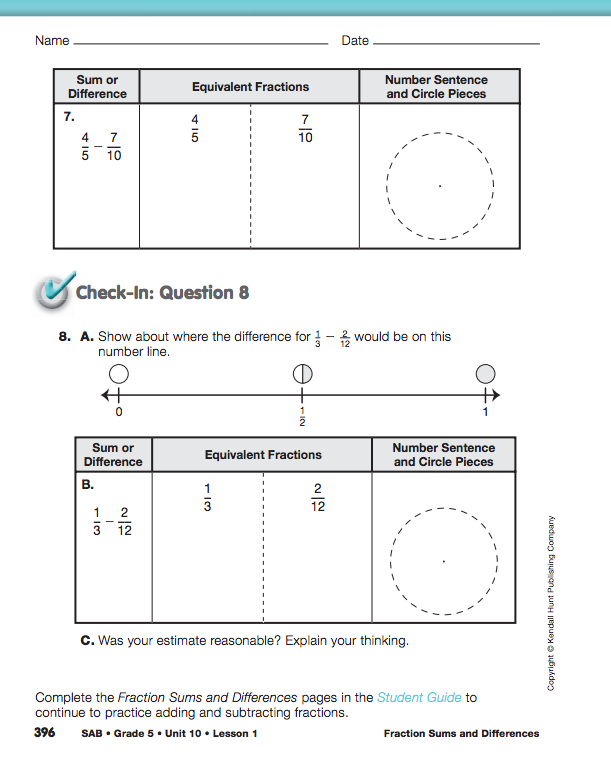
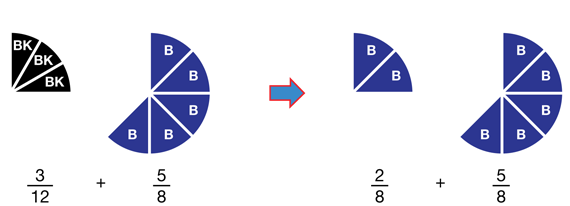
Students may find Questions 5 and 6 more challenging since there is not an obvious single color that can be used to represent both fractions in the sum. For example, in Question 5 there does not appear to be a common denominator that can be shown by a single-color fraction circle piece for 3/12 and 5/8. However, if students first recognize that 3/12 is not in simplest form, they can rewrite it as 1/4. Then they will see that 8 is a common denominator for both fractions. A similar approach can be used for Question 6. Be sure to discuss student solutions to these questions.

Discuss Strategies For Finding Common Denominators. After students have completed all or most of the sums and differences in the pages, take a few minutes to discuss the strategies they used to find common denominators.

Use a few of the problems for this discussion. Ask students to share what common denominator they found. Then ask if anyone found a lower common denominator. The common denominators shown in the Answer Key represent the lowest possible common denominator for each problem.

Ask students to complete Question 8 on the Use Equivalent Fractions to Add and Subtract pages in the Student Activity Book independently.

Assign the Estimate, Add, and Subtract Fractions pages in the Student Activity Book as homework.