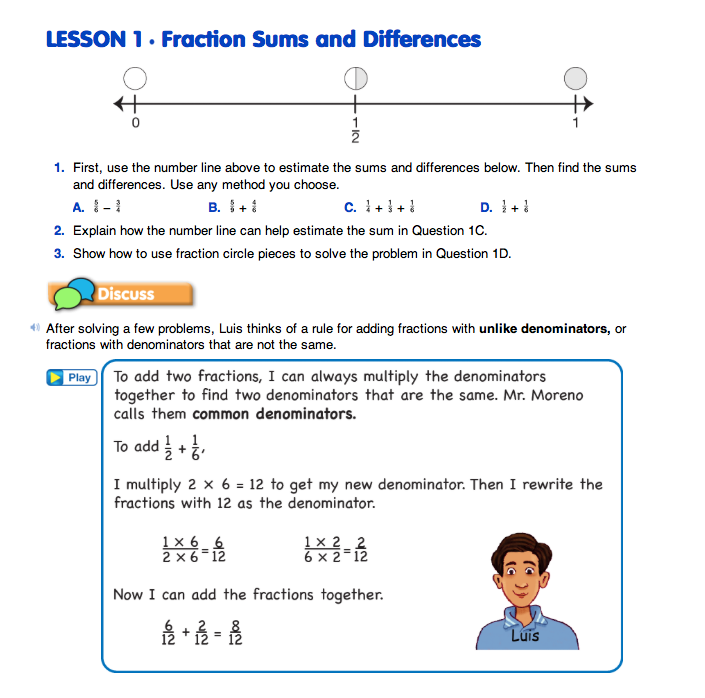
Have students work in pairs to answer Questions 1–3 on the Fraction Sums and Differences pages in the Student Guide. Questions 1–3 provide more practice with estimation and fraction sums and differences. Students can use these questions to try some of the strategies that may have been presented in the class discussion.
As a class, read through Luis's strategy of multiplying denominators together to find a common denominator.
Have students discuss his strategy with their partners using Question 4 as a prompt to guide the discussion.
Assign Check-In: Questions 5–7 for students to complete individually. Students will estimate fraction sums and differences and then use any strategy they choose to find exact fractions sums and differences.
Encourage students to refer to the Fraction Chart, Fractions on Number Lines Chart, and Finding Equivalent Fractions and Ratios Menu in the Student Guide Reference section. Provide access to fraction circle pieces.
Use Check-In: Questions 5–7 on the Fraction Sums and Differences pages in the Student Guide and the corresponding Feedback Box in the Teacher Guide to assess students' abilities to estimate sums and differences of fractions using benchmarks and mental math strategies [E9]; add and subtract fractions including those with unlike denominators using area models and paper-and-pencil methods [E8]; find a strategy [MPE2]; and check for reasonableness [MPE3].

If students are not able to find estimates using mental math or the Fractions on Number Lines Chart, have them look back at the problems on the Use Equivalent Fractions to Add and Subtract pages in the Student Activity Book. Ask them to sketch a fraction benchmarks number line and use it to estimate these sums and differences without finding exact answers.
Discuss using benchmarks of 0, 1/2, and 1 in their strategies. For example, to estimate the answer to 2/4 − 3/8, students can reason that 2/4 is equal to 1/2, and using the number lines chart they can say 3/8 is a little less than 1/2. So, 2/4 − 3/8 will be close to 0.
It is important that students can estimate using number lines, mental images of fraction circle pieces, or benchmarks before they learn formal paper-and-pencil procedures for adding and subtracting fractions.