Workshop: Add and Subtract Fractions

Est. Class Sessions: 2

Developing the Lesson

Part 2: Workshop

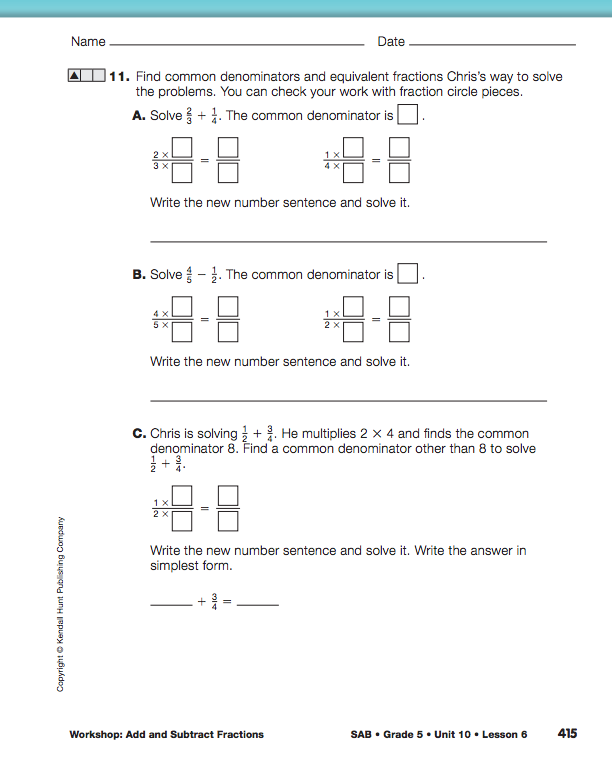
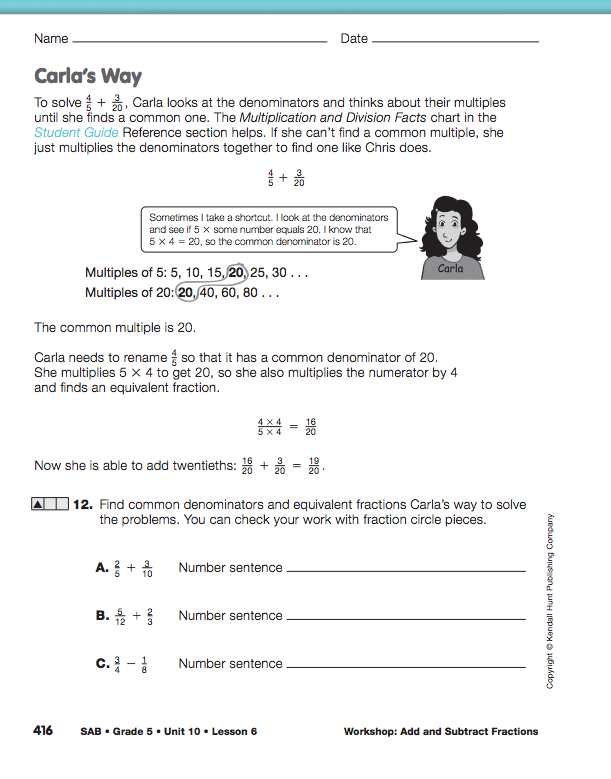
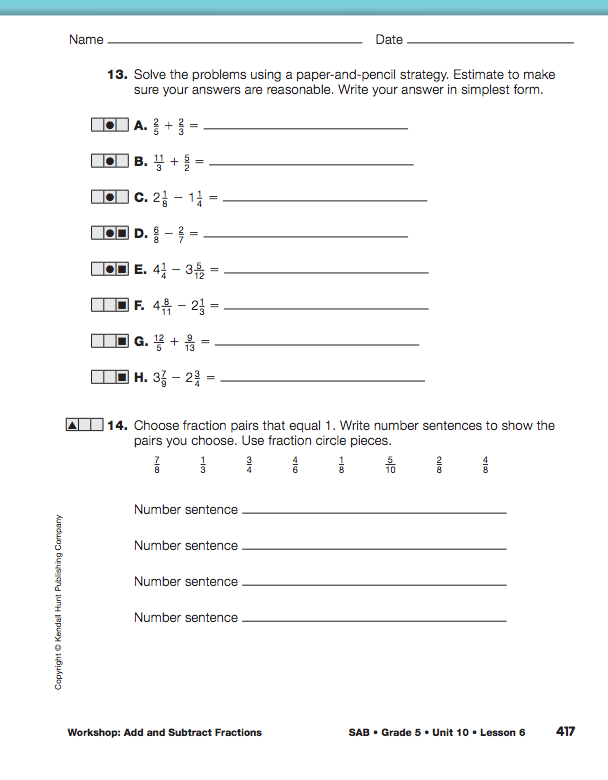
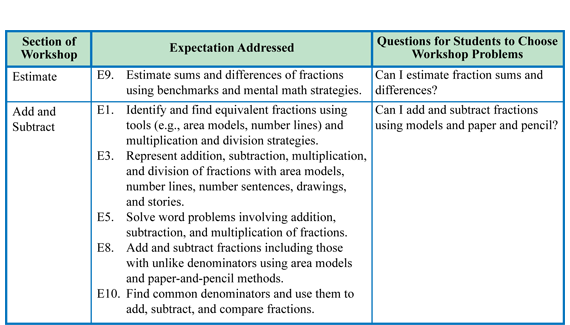
Choose Targeted Practice. Direct students to the problems and Workshop Menu on the Find Fraction Sums and Differences pages in the Student Activity Book. Minis of the Workshop pages not shown here are in the Answer Key. This Workshop addresses Expectations as shown in Figure 2.

Assign Self-Check: Questions 1–2 for students to complete individually. These questions serve two purposes. First, they clearly communicate the content of the related targeted practice to students. Second, they help students quickly self-assess their progress with Expectations to help them choose which problems to work on in the Workshop. Students may use the Fraction Chart, Fractions on Number Lines Chart, and the Multiplication and Division Facts pages in the Student Guide Reference section as they are working.

Students use Self-Check: Questions 1–2 and the Workshop Menu in the Find Fraction Sums and Differences pages in the Student Activity Book to assess their abilities to find equivalent fractions [E1]; represent addition and subtraction of fractions with area models [E3]; add and subtract fractions including those with unlike denominators using area models and paper-and-pencil methods [E8]; estimate sums and differences of fractions using benchmarks and mental math strategies [E9]; and find common denominators and use them to add and subtract fractions [E10].

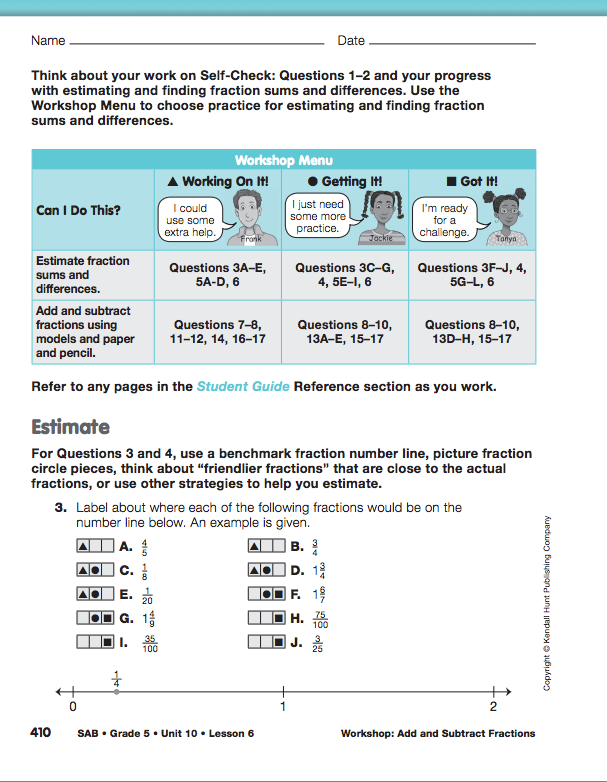
After completing the self-check questions, students self-assess by thinking about the “Can I Do This?” questions in the left-hand column of the Workshop Menu. See Figure 3. Ask students to think about their progress with these Expectations and choose from the following groups:

Students who are “working on it” and need some extra help should circle the problem set marked with a triangle (). These problems provide scaffolded support for developing the essential underlying concepts as well as some opportunities for practice.
Students who are “getting it” and just need more practice should circle the problem set marked with a circle (). These problems provide opportunities to practice with some concept reinforcement and some opportunities for extension.
Students who have “got it” and are ready for a challenge or extension should circle problems marked with a square (). These problems provide some practice and then move into opportunities for extension.

Check students' choices to see how well they match your own assessment of their progress on the related Expectations. Help students make selections that will provide the kind of practice they need.

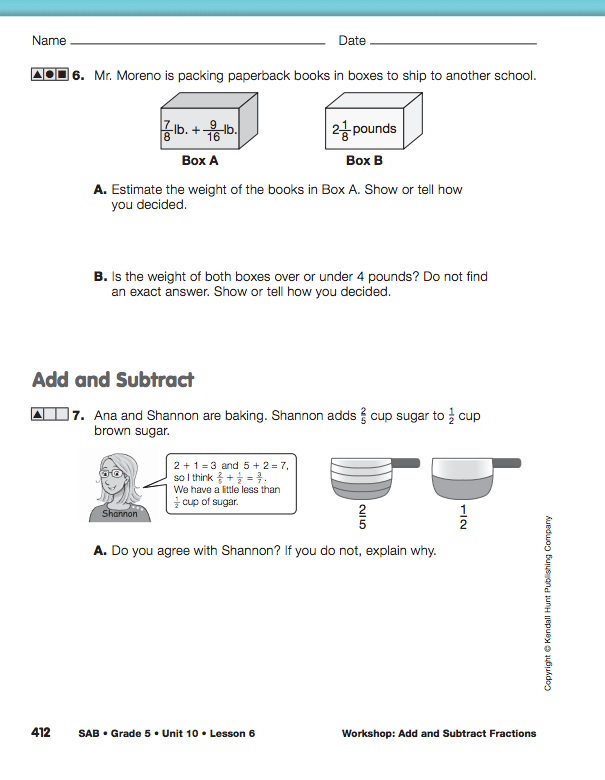
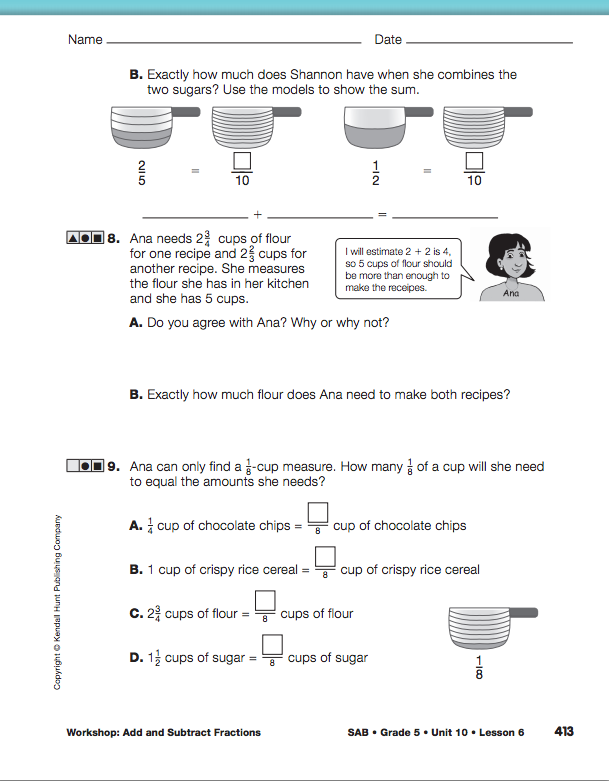
Find Fraction Sums and Differences. Once students select the questions to complete in the Workshop, have them work independently or with a partner to solve the problems they chose. Match groups of students who have chosen similar sets of problems from the menu. Encourage students to use the Fraction Chart, Fractions on Number Lines Chart, and the Multiplication and Division Facts pages in the Student Guide Reference section as they are working.

For students who need more time, unfinished problems can be completed at the end of the Workshop lesson as needed. They can also be sent home as homework. For students who finish all of their selected problems before the rest of the class, encourage them to work on more advanced problems from the menu or begin to play either the Circle Duets or Closest To game.

Address Common Errors. A common error in adding fractions is to add both the numerators and denominators. Question 7 on the Find Fraction Sums and Differences pages in the Student Activity Book addresses this misconception. Ask students who struggle with this concept to explain why Shannon's reasoning does not make sense, and to connect the operation with the models on the page.

Another difficulty lies in finding common denominators because students struggle to find multiples of the denominators. In Questions 11–12, students are shown two ways to find common denominators. In Question 11, students multiply the denominators together. Although finding the least common denominator is preferred because computation is easier, remember that any common multiple will work. With practice, students will learn that finding the smallest multiple is more efficient.

In Question 12, students practice listing multiples of the denominators to find common ones. This method requires students to have good recall of multiplication facts. For students who struggle, encourage the use of the Multiplication and Division Facts chart in the Student Guide Reference section to find multiples.

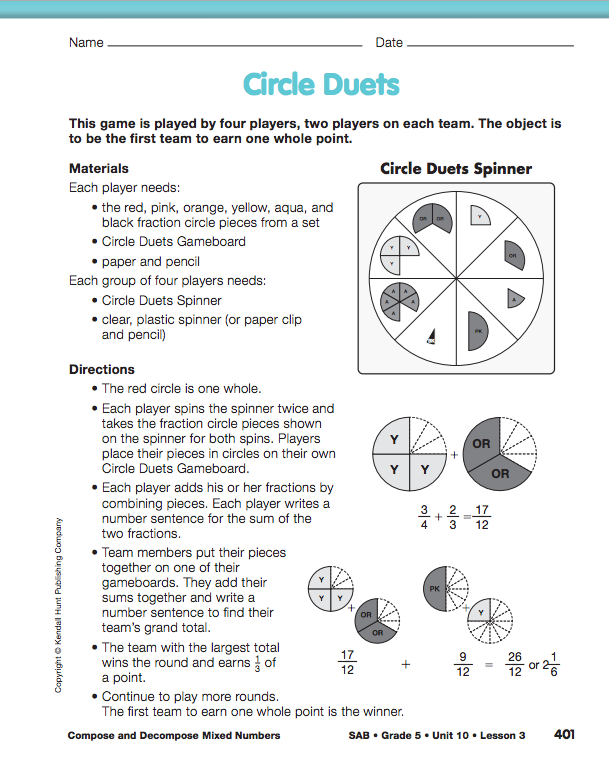
Play Circle Duets or Closest To Games. At the end of the Workshop, students choose to play one of the two games introduced in this unit. Students may play Circle Duets from Lesson 3 to practice adding fractions. Each student playing Circle Duets needs pieces of the following colors from a set of fraction circle pieces: red, pink, orange, yellow, aqua, and black, and their Circle Duet pages from the Student Activity Book. Each group needs one spinner or paper clip and pencil per game.

Students may opt to play Closest To from Lesson 5 to practice estimating and finding fraction differences. Each player needs their prepared set of 4 Number Squares cards (0, 1, 2, 3) and a copy of the Closest To Recording Sheet Master. Each group needs a prepared set of Closest To Game Cards from Lesson 5. For this game, students start with the minuend 41/4 and subtract the mixed number displayed on a game card. Briefly review the directions for the games if needed.

Closest To Game. If a student is struggling with using paper-and-pencil methods to subtract fractions, he or she can be paired to play with a partner who is more able. Both students can estimate the differences, and one student can find the exact difference. Being able to estimate fraction sums and differences is very important, especially for students who struggle with using paper-and-pencil algorithms.

Another alternative is to create a deck of Closest To Game Cards only with fractions that can be modeled with fraction circle pieces (e.g., 21/2, 13/8, 33/4, 21/3, 22/12 , 12/5, etc.) Pull out the game cards displaying other fractions (e.g., 21/7, 25/11 , 33/16 , 15/9, etc.) Encourage students to use fraction circle pieces during the game. The Closest To Game Cards Master is in Lesson 5.