Group and Count to Measure Length
Children strengthen their sense of number by solving problems involving measurements and data. Measuring by laying multiple copies of a unit end to end and then counting the units by using groups of tens and ones supports children's understanding of number lines and number relationships.
From the National Council of Teachers of Mathematics, Curriculum Focal Points for Prekindergarten through Grade 8 Mathematics: A Quest for Coherence, 2006.
LENGTH

Group to Count Efficiently. Throughout the unit, students continue to practice grouping … read more
Group to Count Efficiently
Throughout the unit, students continue to practice grouping objects by twos, fives, and tens in order to find more efficient ways to count. They exchange pennies for nickels and dimes, and count by fives or tens and then count on to find the value of coins. While measuring with nonstandard units like links, paper clips, or connecting cubes, students practice arranging the items in groups of five or ten to facilitate skip counting.
Measure with Standard and Nonstandard Units. Students explore measurement concepts using a variety of units. They estimate, … read more
Measure with Standard and Nonstandard Units
Students explore measurement concepts using a variety of units. They estimate, discuss, predict, and share their observations and thinking. Students have a rich informal background with measurement. Their experiences have probably included informal work with comparisons that has helped them build measurement language, such as long, tall, short, longer, shorter, longest, and shortest.
Measuring with nonstandard units, such as links, and standard units, namely inches, gives students concrete models to use for comparison.
The Rolling Along with Links lab provides students with authentic reasons to measure and real reasons to compare quantities. They collect data in order to compare measurements, determining which car is the "better roller." See Figure 1.
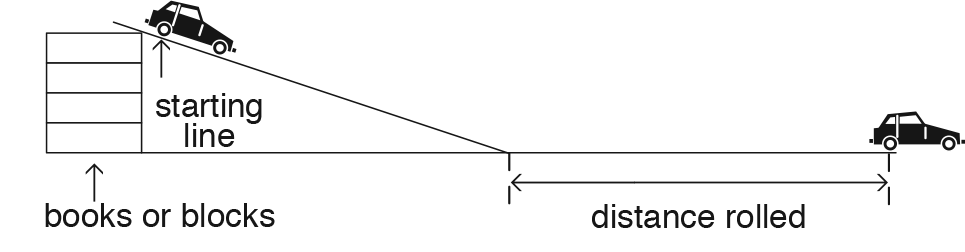
Figure 1: Measuring distance rolled in links
The type of car and the distance each travels are the two experimental variables that students study. This lab contains two basic ingredients of real-world experiments: (1) reliable results depend on doing everything the same way each time we complete a trial—in this case, roll the cars, and (2) the results may not be the same each time (but they should be close). Experimental variables that stay the same are called controlled or fixed variables. Students understand controlled variables as keeping everything "fair."
Students will find that, despite keeping certain variables controlled, results vary. The cars will not roll the same distance each time. Scientists conduct more than one trial for each observation, i.e., they perform the experiment several times to account for these discrepancies. They then use the average value to make predictions. Like scientists, students will conduct several trials, then use an average. One type of average is a median, or middle value. To find the median, students can roll a car down the ramp three times, mark the distance of each roll, and measure only the middle distance or they can measure all three distances and use the value in the middle.
TIME

Measure Time. Time is measured in units. Decades, centuries, and millennia are … read more
Measure Time
Time is measured in units. Decades, centuries, and millennia are examples of very large units of time. We use a clock to measure smaller units of time—seconds, minutes, and hours. Calendars are used to measure time in the middle ranges of days, weeks, months, and years.
There are two attributes of time that are measured: the duration of an event and the time of occurrence. The time of occurrence can be defined in general terms, for example: "I did this today," "I left this morning," or "my birthday was last week." It can also be defined more specifically: "I arrived at three o'clock" or "We will leave at 2:15." The second attribute, the duration of an event, is the measurement of the length of an event from its start to its finish.
In first grade, the study of time begins by helping students understand the attributes of time by first comparing events that have different durations. Students learn about seconds, minutes, and hours and develop some concept of how long these units are using familiar activities in their lives.
Clock Reading. "The common instrument for measuring time is the clock. However … read more
Clock Reading
"The common instrument for measuring time is the clock. However, learning to tell time has little to do with time measurement and more to do with the skills of learning to read a dialtype instrument" (John Van de Walle, p. 397, 2013).
Reading an analog clock is very complex. It not only involves reading two or more scales (hours, minutes, and possibly seconds), it also requires reading those scales in a circular, as opposed to a linear, pattern. Digital clocks are easier to read, but without a conceptual understanding of how hours and minutes relate to one another, the time display holds little meaning.
To help students understand and read an analog clock, we begin by telling time using a one-handed clock. This allows students to develop approximate language relative to the hours on a clock. See Figure 2.

Figure 2: Telling the approximate time using the hour hand
Once students understand the hours on the analog clock, they are introduced to the minute hand and the relationship between the movements of the minute and hour hands. Students first learn to tell time to the nearest hour and half hour on an analog clock. They then make connections between the time displayed on a digital clock and the analog clock.
MATH FACTS and MENTAL MATH

Addition Facts. Students work on developing number sense and mental math strategies for the addition … read more
Addition Facts
Students work on developing number sense and mental math strategies for the addition facts with sums to ten in Group C. See Mathematics In this Unit in Unit 6 for more about addition facts development in Grade 1.
Group C sums to 10: 1 + 9, 2 + 7, 2 + 8, 3 + 6, 3 + 7, 4 + 6, 5 + 5
These facts involve the make-ten and use-ten addition strategies. For example: Since 8 + 2 = 10, 2 + 7 is one less, or 9.
Resources
- Curriculum Focal Points for Prekindergarten through Grade 8 Mathematics: A Quest for Coherence. The National Council of Teachers of Mathematics, Reston, VA, 2006.
- Principles and Standards for School Mathematics. The National Council of Teachers of Mathematics, Reston, VA, 2000.
- Van de Walle, J.A., K.S. Karp, and J.M. Bay-Willams. Elementary and Middle School Mathematics: Teaching Developmentally, Eighth Edition. Pearson Education, Inc., Upper Saddle River, NJ, 2013.












