Lesson 1

From the Fish Hatchery

Est. Class Sessions: 2

Developing the Lesson

Part 1: Two-Digit Multiplication with Rectangles

Share Estimation Strategies. Have students read the short vignette at the beginning of the From the Fish Hatchery pages in the Student Guide. Questions 1A–D involve estimation of the total number of eggs received by Mrs. Dewey's class, 12 × 27. List students' estimates on the board and have them explain their strategies for estimation.

X

Discuss the context and vocabulary in the vignette. A fish hatchery is a facility that raises fish. It provides an optimum environment for fish eggs to develop and hatch by maintaining proper water temperature and oxygen levels, and providing adequate food supplies and safety from predators. In order to survive, the fish eggs need water that does not have too much or too little acid in it.

Note whether students choose convenient numbers that they can easily multiply mentally. See the Mathematics in this Unit section in the Teacher Guide for a discussion of possible difficulties students may have with estimation.

In Question 1C, a student rounds the 12 in the problem to 10 and multiplies, knowing that it is easier to multiply by 10 than by 12. He then states that the answer has to be more than 270 eggs, the product of 10 × 27. Since he rounded 12 down to 10, Luis knows his estimate is lower than the exact answer. His estimate may not be as close as estimates resulting from other strategies, but sometimes estimating a minimum or maximum can be very useful.

Question 1D provides an estimate by rounding one number up and one number down, to make the problem 10 × 30 = 300 eggs.

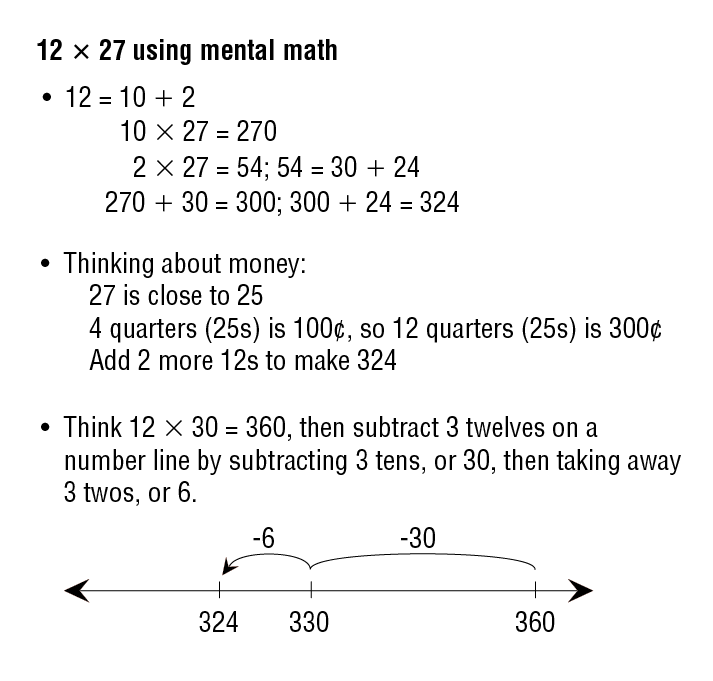
Use Mental Math. Ask students to work in pairs to answer Questions 2 and 3. Students must find an exact answer using mental math strategies. Remind students that they should do as much work as possible in their heads. They should not write the problem and only make notes such as the answer to an intermediate step. Challenge them to solve both problems using more than one strategy. See Figures 1 and 2 for several ways to solve each problem without using a paper-and-pencil method.

X

For Questions 2 and 3, some students may want to do the problem using a paper-and-pencil method. Acknowledge their enthusiasm and tell them that they will be able to demonstrate that method later in the lesson or in the next lesson. Remind them, however, that for now, the question is asking for methods other than paper and pencil.

As students describe their strategies, involve the whole class by asking questions such as:

X
  • Have you ever used [student's name]'s strategy to solve a problem?
  • Tell [student's name]'s strategy in your own words.
  • Use [student's name]'s strategy for solving 12 × 27 to solve 13 × 26.
  • Whose strategy do you like best for 12 × 27, [student's name]'s or [student's name]'s? Why?
  • Which makes more sense to you?
  • Which is easiest for you to do in your head or with just a few quick notes? Which is hardest for you?
  • What steps did you write down? What did you see in your head?
  • Show a way to use subtraction to solve 12 × 27.
    (See the third example in Figure 1.)

Discuss Question 2B:

X
  • Are your exact answers close to your estimates?
  • If not, do you need to check your exact answers?

Continue reading the page in the Student Guide about the fish egg containers. The hatchery sent 25 eggs in each of 13 containers. Questions 4 and 5 ask students about a discrepancy that Mrs. Dewey noticed: 13 × 25 does not equal 12 × 27. Let students hypothesize about why they think the fish hatchery might have sent 325 fish eggs instead of the expected 324 (12 for each student).

Student Guide: 488
Answers
X
SG_Mini
+
Student Activity Book:
Master:
Possible strategies for solving 12 × 27 without using a paper-and-pencil method
X
+
Possible strategies for solving 22 × 15 without using a paper-and-pencil method
X
+