A Different Sieve

Est. Class Sessions: 2

Developing the Lesson

Completing the Chart. This activity is similar to the activity in Lesson 2. However, in this activity students sift for prime numbers using a Six-Column 100 Chart instead of a traditional 100 Chart. After completing the chart, they find several different patterns and write about the patterns they see.

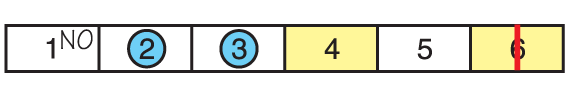
Read the directions to A Different Sieve in the Student Guide. Make sure students understand that they will circle the prime numbers and mark out multiples of the first four prime numbers 2, 3, 5, and 7 on a Six-Column 100 Chart. They should use a different colored crayon to identify the multiples of each of these numbers. If a number is a multiple of more than one of these numbers, it should be marked with more than one color. For example, 6 is a multiple of both 2 and 3. If you shade all of the multiples of 2 yellow and draw a green, vertical line through all of the multiples of 3, then the box containing the number 6 should be marked with both yellow and green. See Figure 1.

While students should be able to complete this chart independently, you may have them work with a partner. Once a student has completed the sifting process for the multiples of the first four prime numbers, he or she is ready to look for and write about the patterns that are contained in the completed chart. See Figure 2.

Use these or similar questions to get students started or to help students find patterns in the sieve.

Ask:

Where are the even numbers on the Six-Column 100 Chart? (in columns two, four, and six)
Where are the prime numbers? (in the first and fifth columns, except for 2 and 3)
Where are the multiples of five? (The multiples of 5 run down diagonally beginning at the right.)
Are the prime numbers mostly even or odd? (The prime numbers are odd except for 2.)
How are the multiples of 2 and the multiples of 3 marked on your chart? (with a color for two and vertical line down the middle for 3)
Make a list of the numbers that are multiples of both 2 and 3. (They are most of the numbers in column six: 6, 12, 18, 24, 36, 48, 54, 66, 72, 78, 84, 90, 96.)
Which numbers are multiples of more than one of the four numbers we checked? (6, 10, 12, 14, 15, 18, 20, 21, 24, 28, 30, 35, 36, 40, 42, 45, 48, 50, 54, 56, 60, 63, 66, 70, 72, 78, 80, 84, 90, 96, 98, 100) How can you tell? (More than one marking is in the square.)

Setting Problem-Solving Expectations. Give students a few minutes to identify and describe a pattern they see in the chart. Circulate and choose one or two students to share the pattern they found. Use these descriptions to review Math Practices Expectation 5. Work with the class to develop problem-specific expectations using a display of the Math Practices Notes Master. See Figure 3. As students share patterns they found, check to see that they clearly describe the pattern and how it is represented on the Six-Column 100 Chart.

Ask:

In writing about the number patterns you found, how do you describe the patterns so someone else can understand your thinking? (Possible student responses: I describe the patterns using words like prime, factor, composite, and multiple. In my description, I show or tell where the patterns are on the Six-Column 100 Chart. I use words like row, column, right, left, diagonal, first, or third.)

When expectations have been established, ask students to continue working to identify and describe as many patterns as they can in the Six-Column 100 Chart. Some of the patterns that students might write about include:

All of the prime numbers, except for the numbers 2 and 3, are in the first and fifth columns.
The prime numbers are all odd except for the number 2.
All of the multiples of 2 are in columns two, four, and six.
All of the multiples of 2 are even numbers.
The multiples of 3 are in the third and sixth columns.
Every other multiple of 3 is also a multiple of 2.
The multiples of 5 form diagonals that begin at the right and go down.
The multiples of 7 form diagonals that begin at the left and go down.
Every other multiple of 5 is also a multiple of 2. These multiples end in 0.
Every other multiple of 7 is also a multiple of 2.
To find numbers with common factors, you can look for numbers that have the same colors. For example, 27 and 12 have 3 as a common factor because they both have the color for three in their box.

Written feedback provides you with a permanent record of a student's progress toward effective communication. Your suggestions for clarification or expansion of written work will help establish what a student can do independently and what a student can do with additional input or structure. If you give a student verbal feedback, you may want to make a note in your record so you can differentiate between what the student accomplished independently and what the student accomplished with additional input from you.

While students are writing, circulate, observe and provide feedback.

Ask: