Lesson 8

Check It Out

Est. Class Sessions: 2

Developing the Lesson

Part 2: Solving Problems Two Ways to Check for Reasonableness

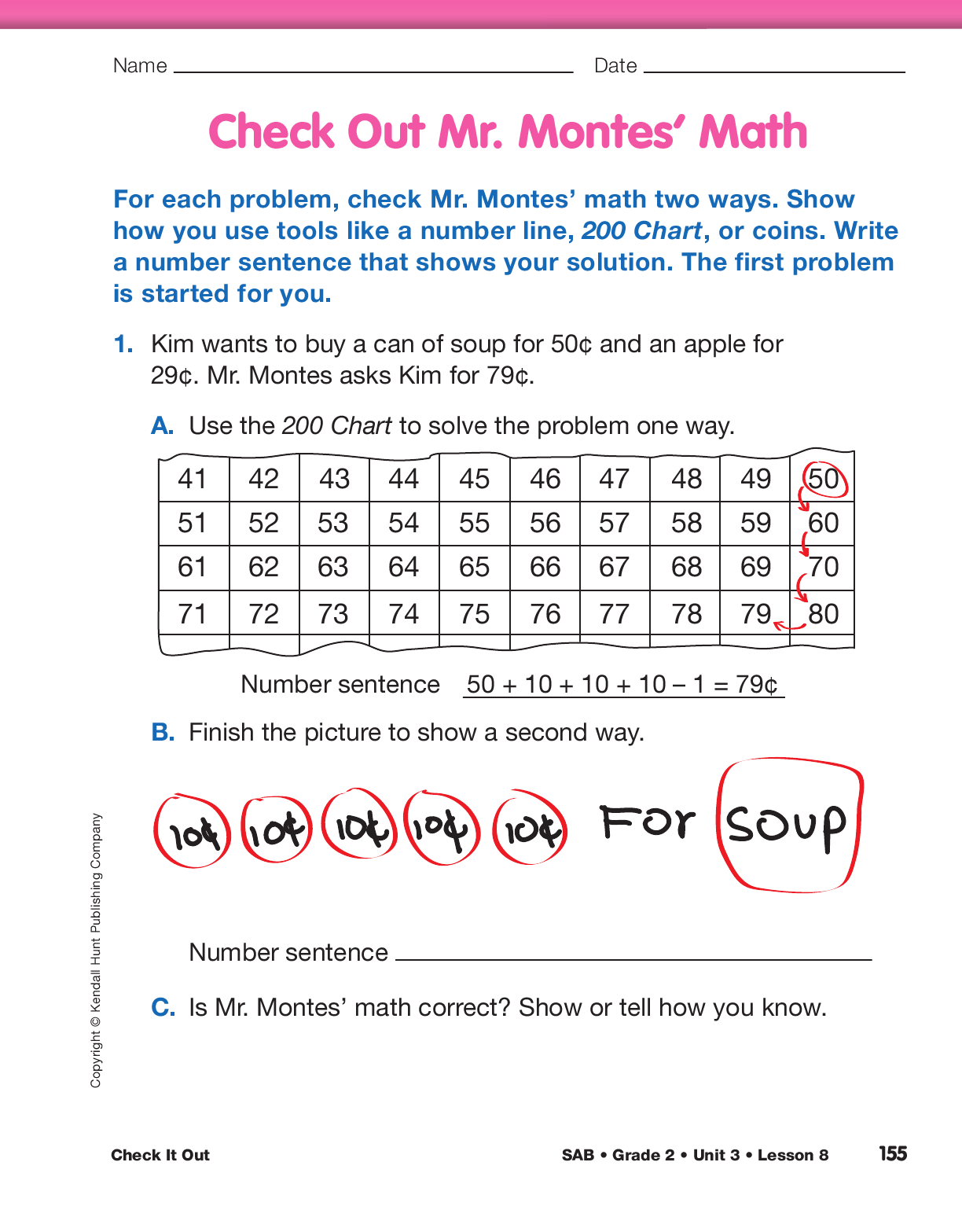
Check Out Mr. Montes’ Math. Tell students that they will now solve some other problems that students found in Mr. Montes’ grocery store. Students should have number lines, 200 Charts, connecting cubes, and coins available to solve these problems. Students should work with partners to solve the problems.

Start by reading Kim’s problem in Question 1 of the Check Out Mr. Montes’ Math pages in the Student Activity Book.

Kim solved the problem two ways. Ask students to look at how Kim used the 200 Chart to solve 50 + 29.

Ask:

X
  • Where did Kim start? (50, the cost of the can of soup)
  • What did Kim do next? (She skip counted by tens to add 30 to 50.)
  • Why did she add 30? (30¢ is just one more than 29¢. Adding 30 is just one too many.)
  • Why did Kim go back one on the 200 Chart? (She added one too many when she added 30. By going back one she is adding 29.)
  • How does Kim’s number sentence show how she moved on the 200 Chart to solve this problem? (Possible response: 50 is where she started. Each +10 shows how she skip counted by ten on the 200 Chart and the −1 shows how Kim moved back one because she had added one too many.)
  • What label does Kim use so we know what the 79 means? (cents)

Kim also started to solve the problem a second way. Ask students to finish Kim’s picture to solve the problem.

Ask:

X
  • What did Kim draw? (She drew 5 dimes to show 50¢ for the soup.)
  • What did you add to Kim’s picture? (two dimes and nine pennies for an apple)
  • Is Mr. Montes’ math correct? How do you know that? (Yes. Kim’s answer was 79¢ and there is 79¢ in the picture.)
  • How can you use the picture to show that Mr. Montes’ math is correct? (Skip count by ten for the dimes and then count on for the nine pennies.)
  • How does solving a problem two ways help you check the reasonableness of your answer? (If the answers don’t match, then something isn’t right. I can try to solve the problem again so that my answer makes sense.)

Have students work in pairs to solve the other problems on the Check Out Mr. Montes’ Math pages. As you circulate, ask students to show how they are using the tools and strategies. Encourage students to show their strategy in the number sentence, like Kim. See the Sample Dialog for a sample discussion of Question 3.

X

Use this sample dialog to guide your discussion of
Question 3 from the Check Out Mr. Montes’ Math page.

Teacher: Who can show how you figured out if Mr. Montes gave Nisha the correct change?

Chloe: I used the 200 Chart. I started at 90¢ and moved to the row above to 80. That’s 10 and I moved to the left by ones 7 more and I got to 73. Mr. Montes gave the right change.

Teacher: What is your number sentence?

Chloe: 90¢ − 10¢ − 7¢ = 73¢ or 90¢ − 17¢ = 73¢

Teacher: Does everyone understand why Chloe counted back 10 and then 7?

Casey: When you count back 10 and 7, that’s the same as counting back 17.

Teacher: Good answer! Did someone solve it another way?

Brandon: I used the number line. I started at 73 and made 2 jumps of 10 to 93. Then I moved back 3 ones to the left because Nisha gave Mr. Montes 90¢, not 93. My number sentence is 73 + 10 + 10 − 3 = 90. My number sentence could also be 73 + 17 = 90.

Teacher: Remember to use labels. What is the label for your numbers?

Brandon: Oh, it’s 73¢ + 10¢ + 10¢ − 3¢ = 90¢.

Teacher: We only had to solve it two ways, but did anyone else use a different strategy?

Javier: I used connecting cubes to solve the problem. I took 9 tens and I took away 7 tens and 3 ones from another ten. I had 1 ten and 7 ones and that makes 17. My number sentence is 90¢ − 73¢ = 17¢.

Kathy: My number sentence is 17¢ + 73¢ = 90¢. I started with the change 17¢ and counted on 73 to see if that equaled 90. I did it on the 200 Chart. Is that right?

Teacher: What does everyone think? We have
90¢ − 73¢ = 17¢, 90¢ − 17¢ = 73¢, 73¢ + 17¢ = 90¢,
and 17¢ + 73¢ = 90¢. The number sentences are not the same but do all of them show reasonable answers?

Grace: Oh, I see. They all belong to the same family! Every number sentence uses 90, 73, and 17! All the number sentences show that 17¢ is the correct change.

Teacher: That’s right. Are they all reasonable strategies? Does our answer make sense?

Joey: Yes. Everyone just solved it a different way.

Teacher: We can find out if our answers are reasonable by solving the problem in more than one way.

Self-Assess with Math Practices Checklist. Assign the Two Solutions page in the Student Activity Book to assess students’ abilities to solve a problem in more than one way. Have desk number lines, 200 Charts, coins, and connecting cubes readily available. Display and direct students to the Math Practices page in the Student Activity Book Reference section to review the following Expectations:

  • MPE2: Find a strategy. I choose good tools and an efficient strategy for solving the problem.
  • MPE3. Check for reasonableness. I look back at my solution to see if my answer makes sense. If it does not, I try again.
  • MPE6. Use labels. I use labels to show what
    numbers mean.

Explain to students that solving the problem two ways helps them check the reasonableness of their solutions. Emphasize that both ways should have the same answer and if not, they should go back and check their solutions.

X

Use the Two Solutions page in the Student Activity Book to assess students’ abilities to represent addition using multiple representations (e.g., stories, drawings, diagrams, counters, number sentences, number lines, 200 Chart) [E3].

Students use the Self-Check: Checklist on the Two Solutions page to self-assess their abilities to find strategies to solve problems [MPE2]; check for reasonableness [MPE3]; and use labels to show what numbers mean [MPE6].

Adventure Book:
Student Activity Book: 155 156 157
Answers
X
SG_Mini
+
X
SG_Mini
+
X
SG_Mini
+
Master: