Use Math Practices.
Display 4 red and 9 blue connecting
cubes on the display and ask:
- How many more blue cubes are there? (5 cubes)
- Show me how you figured that out? (Possible
response: I knew 4 matched up and then counted
up from 4 to 9 and found that was 5.)
Refer students to the Math Practices page you have displayed.
- Which of these Math Practices did you just use?
(Possible response: I had to know the problem; I
just showed you my work; I used labels; I found a
strategy.)
Write 4 + 9 = 13 on the display.
- John wrote 4 + 9 = 13 as an answer to this problem. What do you think about John’s response?
(Possible response: John did not interpret the problem. So, he does not know the problem.)
- What can John do to be sure he knows the problem?
(Possible response: Draw a picture, use the cubes to
act out the problem.)
- Look at John’s work again. Do you know what the
problem was about? (No, John did not include units.)
- Is John’s answer reasonable? (Possible response:
No, the problem is asking for a comparison so the
answer has to be between 4 and 9, not more than 9.)
- What could John have done to be sure his answer
was reasonable? (Possible response: He could
re-read the problem and decide if the answer
makes sense.)
- Can you tell me a problem that does match John’s
number sentence? (Possible response: There are
4 red cubes and 9 blue cubes. How many cubes are
there altogether?)
Tell students that in this lesson they are going to solve
many problems and the Math Practices page can help
them remember what to do while solving a problem.
During this lesson, students will focus on the following
Math Practices:
MPE1. Know the problem. I read the problem carefully.
I know the questions to answer and
what information is important.
MPE3. Check for reasonableness. I look back at my
solution to see if my answer makes sense.
If it does not, I try again.
MPE6. Use labels. I use labels to show what numbers
mean.
Act Out Subtraction Word Problems. Have students
act out various separating/taking away and comparison
situations. See the Problem Types Content Note. For a
separating/taking away situation, begin by calling up a
group of 11 students.
- How many students are in front of the class?
(11 students)
Then send 4 students back to their seats.
- How many did I send back to their seats?
(4 students)
- How many are still in front of the class? (7 students)
- Who can tell a subtraction story about these students?
(Possible response: There were 11 students.
4 of them returned to their desks for pencils. How
many remained standing?)
For a comparison situation, have 7 students sit in
chairs and ask 4 to stand.
- How many students are sitting in chairs? (7 students)
- How many students are standing? (4 students)
- How many more are sitting than standing? (3 more students are sitting than standing.)
Problem Types. The Cognitively Guided Instruction Project
(CGI) classified 11 types of addition and subtraction problem.
Two basic subtraction problem types will be used in this
activity:
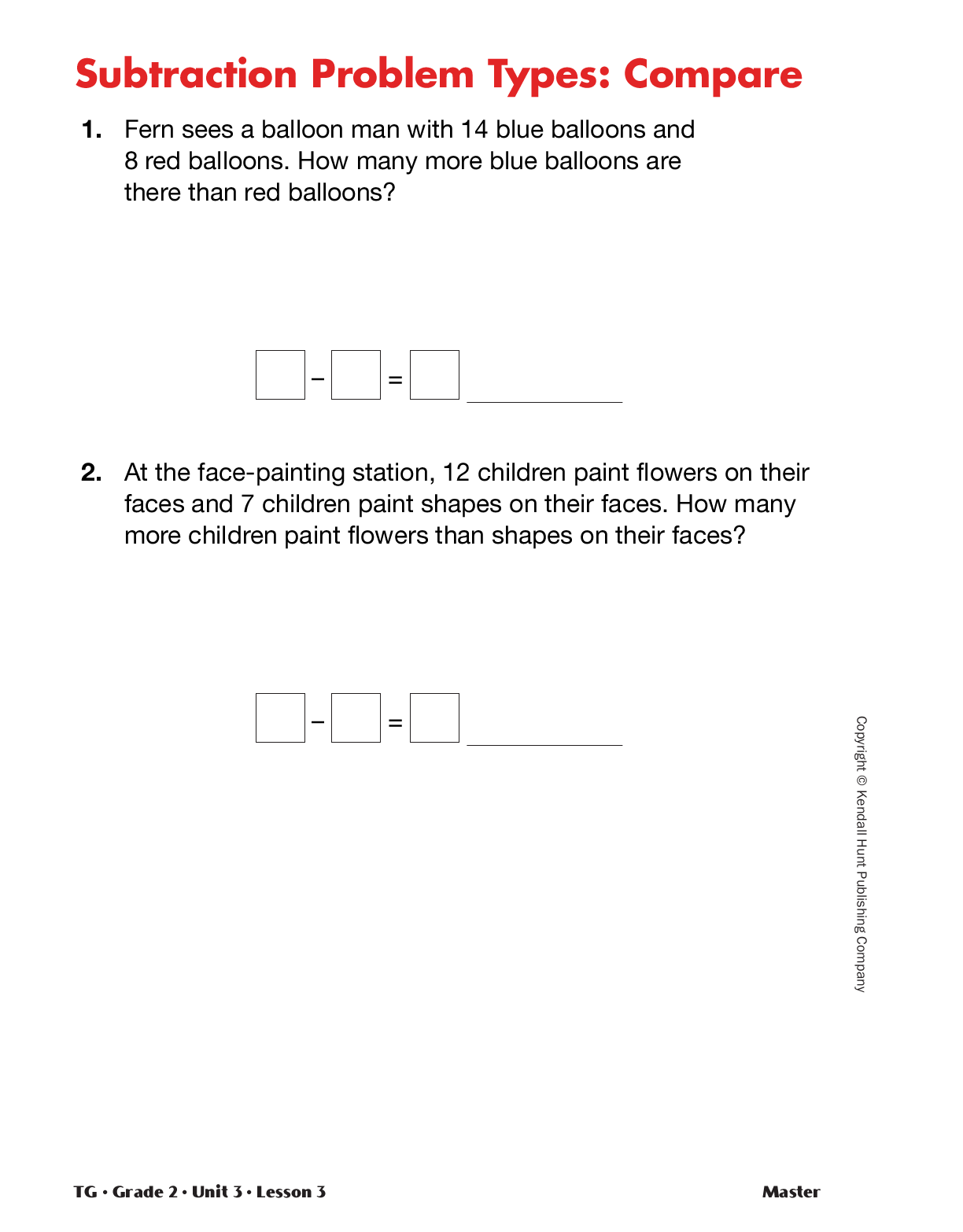
- Separate/Take Away
Frank had 18 tickets at the carnival. He used
9 tickets for
rides. How many tickets were left?
- Comparison
There were 12 turtles and 4 frogs at the petting zoo. How
many more turtles than frogs were at the petting zoo?
See
Mathematics in this Unit for more information on problem types.
Important note: Students do not need to know the names of
the different problem types but it is important that students
encounter multiple examples of subtraction situations.
Discuss Types of Subtraction Problems. Use the display
of the Subtraction Problem Types Masters to help
students become more familiar with separate/take
away and compare situations. As you read each of the
problems, encourage students to refer to the
Subtraction Strategies Menu for the Facts in the
Student Activity Book Reference section. Discuss using
the counting up, counting back, using ten, using doubles,
making ten, and thinking addition strategies.
Remind students that math tools such as the number
line, connecting cubes, and ten frames can help as
well.
As students consider each problem, ask the following
questions to emphasize the Math Practices
Expectations:
- What is the question? What words and numbers do
you need to answer the question?
Underline the words, numbers, and questions needed
to solve the problem.
- What tools or strategies can you use to solve the
problem? (See the Subtraction Strategies Content Note.)
- Who would like to act out or draw the problem?
- What should be included in your work? (Possible
response: my thinking, tools I used or thought
about, a number sentence)
- What label do you need to include in your answer?

Subtraction Strategies. Students may use a variety of
strategies to solve a subtraction problem. They should be
encouraged to try different strategies and to share their
strategies. A discussion of strategies helps students verbalize
number relationships and encourages them to think about
problems in new ways. It is important to emphasize that a
strategy that works well for one person may not be helpful to
another. It is not necessary for students to remember the
names of strategies, but they should remember how to use
those that make sense to them. The following is an
explanation of some subtraction strategies:
- Counting Up: Students may use this strategy for problems
with missing addends. For example,
6 + = 10. Start
with the lower number and count up to 10: 7, 8, 9, 10. The
answer is 4.
- Counting Back: Students may use this strategy for subtracting
smaller numbers. For example, to solve
10 − 2,
students count back 2 numbers: 9, 8. The answer is 8.
Counting back is similar to the counting-on strategy but
the numbers are getting smaller instead of larger.
- Reasoning from Related Addition and Subtraction Facts:
As students begin to understand fact families, they may
use related addition facts to solve subtraction facts. For
example, knowing 4 + 3 = 7 will help students solve
7 − 4 and 7 − 3.
After considering the questions for each problem, have
student pairs find the solution. Encourage students to
solve the problem in more than one way (counting up,
counting back, thinking addition). Remind students
that when writing a subtraction number sentence, they
should write the larger number in the problem first and
subtract the smaller number. See the Subtraction
Properties Content Note. See the Sample Dialog for a
discussion of strategies and writing number sentences.

Use this dialog to guide your discussion of strategies and
number sentences for Question 1 on the Subtraction Problem
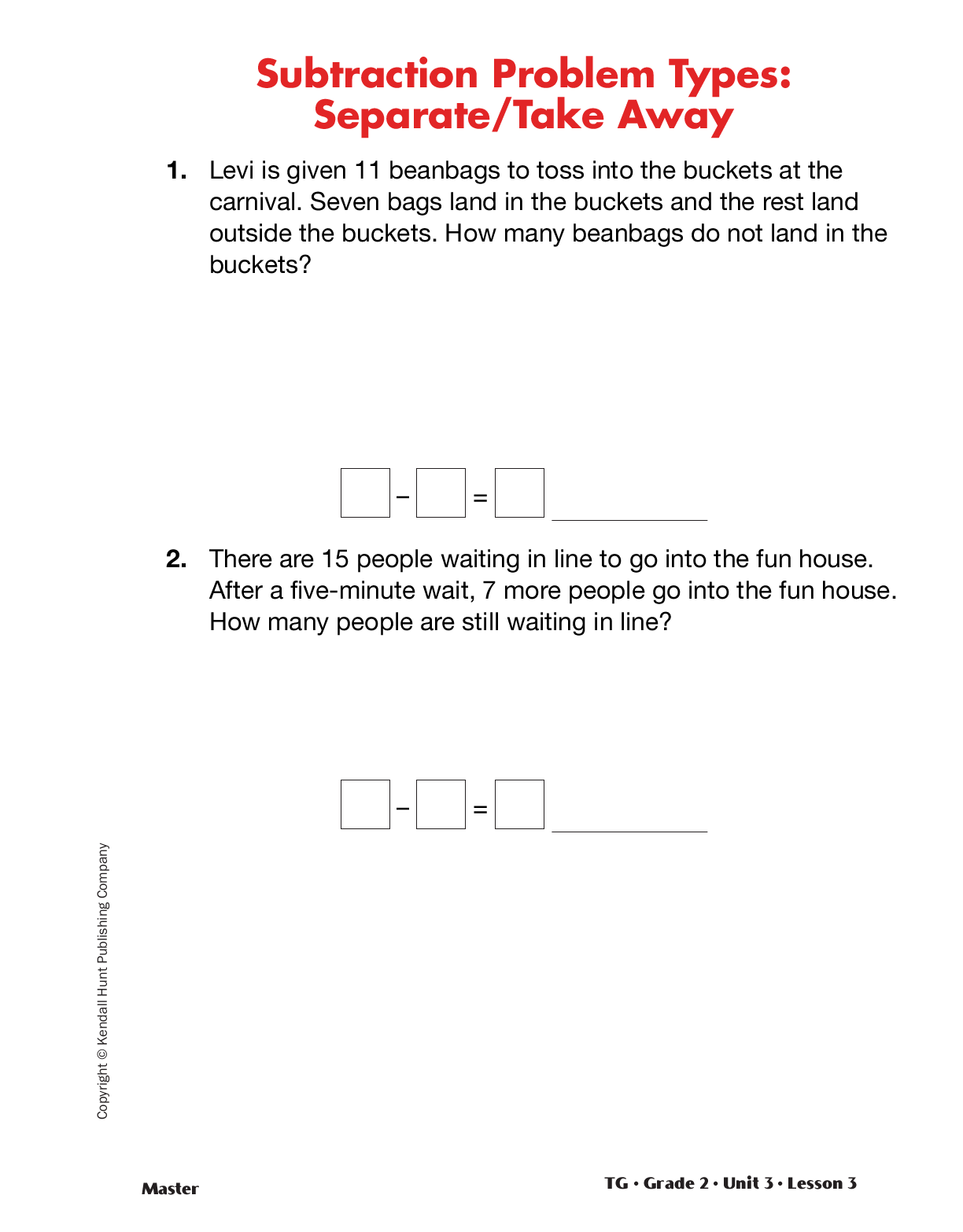
Types: Separate/Take Away Master.
Teacher: What is the question for Question 1?
Julia: How many beanbags did not land in the buckets?
Teacher: What words and numbers do you need to solve
the problem?
Sam: We know that Levi is given 11 beanbags to toss and
7 land in the buckets. The rest did not land in the
buckets.
Teacher: Who can show or explain how you solved the
problem?
Natasha: I used the number line and I counted up from 7 to
11. My answer is 4 beanbags.
Romesh: I used the number line and I counted back 7 and
my answer is 4 beanbags, too. Is that wrong?
Teacher: What do you think?
Romesh: Since I got the same answer, I think it’s just another
strategy you can use because my answer makes sense.
Teacher: I’m glad you checked to see if your answer was
reasonable. How do you know your answer makes
sense?
Romesh: Well, I know the important numbers in the problem
are 7 and 11. Levi had 11 beanbags and 7 landed in the
buckets. If I added instead of subtracting the numbers,
7 + 11, my answer would be 18. How could
18 beanbags miss the buckets if he only had 11
beanbags in the beginning?
Teacher: Good answer! What is your number sentence?
Suzanne: My number sentence is 7 − 11 = 4 beanbags.
Teacher: Are you saying you started with 7 and took away
11?
Suzanne: No, I started with 11 and took away 7.
Teacher: Then what should your number sentence be?
Suzanne: Oh, I see. It should be 11 − 7.
Teacher: Right! When you write a subtraction number
sentence, the order of the numbers is important. What
did you start with? What did you take away? If you’re
comparing two numbers, write the larger number
first.
Jason: I don’t have a subtraction number sentence. I have an
addition number sentence: 7 + 4 = 11. Since I counted
up from 7 to 11, I said 7 plus what number is equal to
11. My answer is 4 also.
Teacher: That’s good, Jason. You can write an addition
number sentence: 7 + = 11 or you can write a
subtraction number sentence: 11 − 7 = . Both times
the number that goes in the box is 4. Just remember
that the missing number in the box answers the
question.

Subtraction Properties. The commutative property in addition
says that the order in which the addends are added does not
affect the sum of the numbers. Both 3 + 2 and 2 + 3 equal 5.
However, this property does not apply to subtraction. When
subtracting, the order in which numbers are subtracted will
change the difference. It is important to emphasize this to
students so that they write subtraction number sentences
correctly.
After students have had an opportunity to solve the
problems, have student pairs demonstrate their work
and explain how they solved the problem.
- What is your number sentence? Did you write it correctly?
- Did anyone solve the problem a different way?
- Did anyone get a different answer?
- Is your answer reasonable?
Referring to the Math Practices page displayed,
remind students to check the answer for reasonableness.
If some students solve the problem by using
addition for a subtraction situation (e.g., 11 + 7
instead of 11 − 7), encourage them to look back at the
problem to see if their answer makes sense.
- Look at the question: How many beanbags do not
land in the buckets? If your number sentence is
11 + 7 = 18, does it make sense that 18 beanbags do
not land in the buckets if Levi only had
11 beanbags?