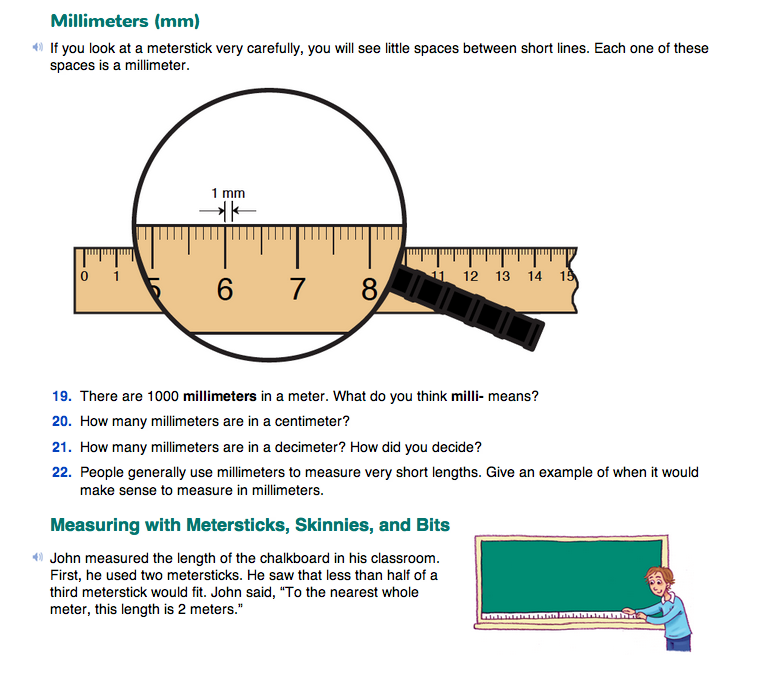
Ask students to read the vignette in the Measuring with Metersticks, Skinnies, and Bits section of the Student Guide. Discuss Mrs. Dewey's written notation to show John's measurement of 2 meters, 4 decimeters, and 1 bit: 2.41m. Reinforce that a decimeter (a skinny) is one tenth (1/10) of a meter long and that a centimeter (a bit) is one one-hundredth (1/100) of a meter long. Write Mrs. Dewey's decimal as a fraction: 241/100. Compare the decimal notation with the corresponding fraction notation.
Continue the discussion by asking:
- How would you record 1 decimeter as part of a meter? (0.1 m)
- What fraction is that? (1/10 m)
- How would you record 1 centimeter as part of a meter? (0.01 m)
- What is it as a fraction? (1/100 m)
- How would you record 1 millimeter as part of a meter? (0.001 m)
- What is it as a fraction? (1/1000 m)
Use the same vignette about John to discuss with students a variation of the Fewest Pieces Rule as applied to measurement.
- If John had used only skinnies and one bit (centimeter) to measure the chalkboard, how many pieces would he have used? (24 skinnies and 1 bit: 25 pieces)
- If he had used only bits (centimeters), how many pieces would he have used? (241 bits, so 241 pieces in all)
- Why did Mrs. Dewey say John had used the fewest pieces? (Because he used only 7 pieces: 2 metersticks, 4 skinnies, 1 bit.)
- Could he have used fewer pieces than 7 and gotten an exact measurement? Why or why not? (If he had measured to the nearest meter, he would have used only 2 pieces, but it wouldn't have been an exact measurement.)

Communicating Accuracy in Measurement. The units of measure signal the accuracy of recorded measurements. For example, to record the height of a door as 2 m tells the reader that the height is measured to the nearest meter. Technically, that means that the height can be anywhere between 1.5 meters and 2.5 meters, which is not very accurate. To record the height of a door as 223 cm communicates that the measurement is accurate to the nearest centimeter and the error in measurement could be at most 0.5 cm. That is, the height is between 222.5 cm and 223.5 cm, which is much more accurate.
In Part 1 of the lesson, students record measurements in whole numbers as discussed above. In Part 2, students use decimals to record measurements. Here the place value of the digits indicates the accuracy of the measurements. For example, if Mrs. Dewey records the length of the chalkboard as 2.4 m, she tells the reader that she measured to the nearest tenth of a meter. When she records the measurement as 2.41 m, she tells us that she measured carefully to the nearest hundredth of a meter.
Recording Measurements with Decimals. Note that 2.4 m tells the reader something different than 2.40 m. Writing 2.4 m tells us that the measurement is accurate to the nearest tenth of a meter, whereas writing 2.40 m signifies that the measurement is accurate to the nearest hundredth of a meter.
Ask similar questions about John's measurement of the height of the basketball player in the poster described in the vignette.
Have students tell a partner in their own words what the Fewest Pieces Rule means when measuring. Then call on a student volunteer to explain to the whole class and have others add to the student's explanation as appropriate. Make sure that students recognize that it means that when measuring, they use as many metersticks as possible, then as many skinnies as possible, and then as many bits as possible.
Have students complete Question 23 in pairs and discuss answers and strategies.
Reading Decimals. The symbols 4.3 are usually read as “four and three-tenths” or “four point three.” Either reading is correct, but we prefer the former at this stage because it makes the connection with fractions explicit. We want students to think of decimals as fractions, not merely as strings of symbols.
See the Writing Numbers in Words page in the Reference section.