Decimal Hoppers

In this part of the lesson, decimal hoppers are introduced to help students represent simple computations with decimals and to introduce the context of the game students will play in Part 2 of the lesson.

Read with the whole class the introductory vignette about decimal hoppers in the Decimal Hoppers pages in the Student Guide.

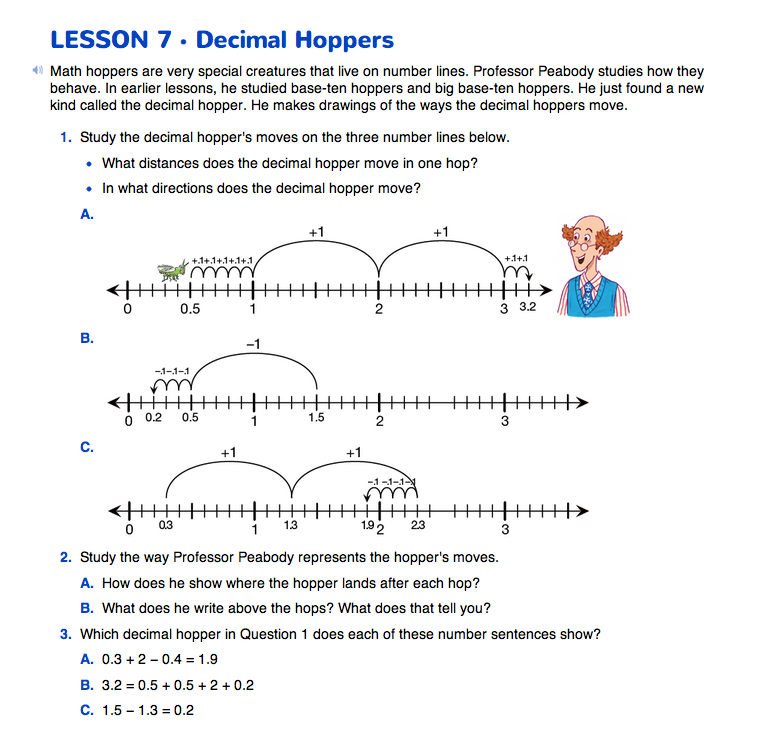
Ask students to work with a partner as they answer Questions 1–5. Question 1 asks students to describe the movements of the decimal hopper. Like the base-ten hoppers from previous units, decimal hoppers can hop only distances based on base-ten place value units (e.g., 1, 10, 100), but now the rule extends into decimal fractions as well as whole numbers. In addition to hops of distances of 1, 10, or 100, decimal hoppers can hop a distance of 0.1 or 0.01 (or 0.001, though hops will be limited to 1, 0.1, and 0.01 in this lesson). Recall that hoppers can jump both to the right and left. When the hopper jumps to the right, the distance is added to the starting position. When jumping left, the distance is subtracted.

In Question 2, students revisit the conventions for showing the hopper's moves. It is important that students can consistently and clearly represent the quantities and directions associated with the movement of the hopper along the number line.

Question 3 provides practice relating the number line representation with number sentences.

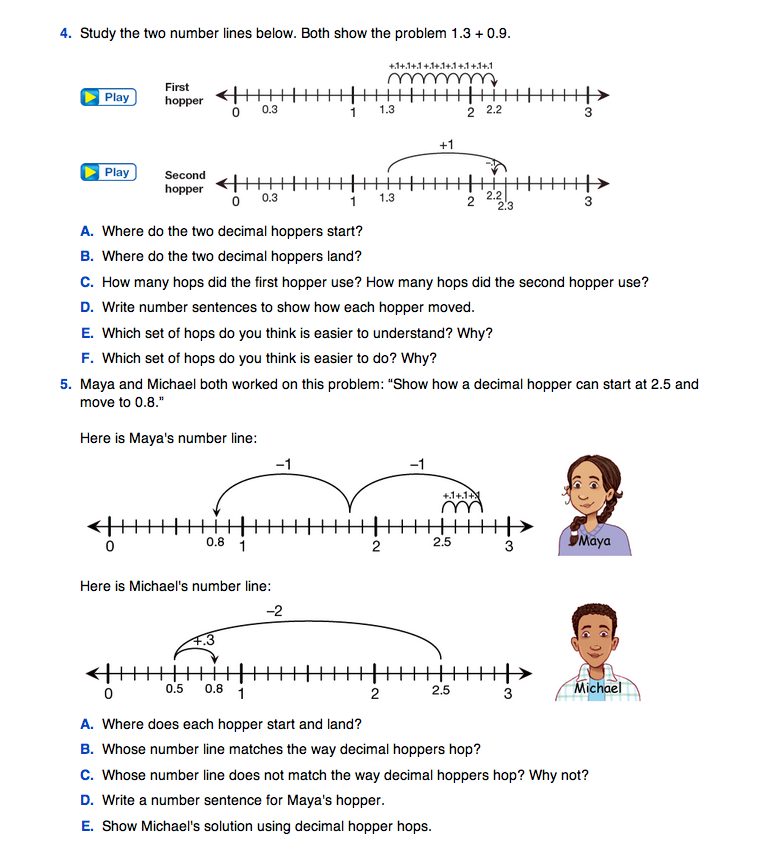
In Questions 4 and 5, students explore the number line and the 'rules' about how decimal hoppers move. It is important for students to realize that there is more than one way (in fact, an infinite number of ways) for a hopper to move from one point to another. For example, Question 4 shows two different ways a hopper can move to represent 1.3 + 0.9. While both hoppers show the same sum, they do so taking different paths. As students think flexibly about getting from one point on the number line to another, they begin to develop flexible notions about adding and subtracting decimals. They also make connections between the number line and numerical representations of decimal sums and differences.

Question 5 clarifies how a decimal hopper moves in preparation for the game in Part 2.