Fraction quantities can be represented numerically using either common fractions or decimal fractions. Students are familiar with common fractions, expressed with a numerator and denominator as with 1/2, 3/5, 7/10, 25/8, or 9/4. Decimal fractions, or decimals, express fractional quantities by including digits to the right of a decimal point, as with 0.5, 0.046, or 2.33. Decimals make use of base-ten place-value concepts to symbolize fractional quantities, which makes them particularly useful for performing computations and recording measurements.

Students make sense of decimal fractions by connecting decimal concepts to their existing understanding of common fractions and by exploring various representations of decimal fractions. For example, students learn the meaning of decimals by reading “4.3” aloud as “four and three-tenths,” writing 1.36 as 1 + 3/10 + 6/100, or seeing that 1/2 of a meter and 0.5 meter occupy the same location on a meterstick. As important, students extend their knowledge of whole number place value to decimals. In the base-ten system, each digit place represents a multiplier ten times that of the next place to the right. For example, the tens place has a value of 10 times the ones place. The hundreds place has a value of 10 × 10, or 100 times the ones place. Applying place value concepts to the right of the decimal, the tenths place has a value of 1/10 the ones place, and the hundredths place has a value of 1/100 of the ones place.

Representations

In this unit, students make connections among different representations of decimals: symbols, words, physical models, and pictures. See Figure 1. Translating among these representations—drawing a shorthand picture of a collection of decimal base-ten pieces, saying numerical decimal fractions aloud, and writing a decimal number—helps students maneuver between the many contexts in which decimals appear in the real world and helps develop conceptual understanding of decimal fractions. Research indicates that students with a foundational understanding of decimals can learn computational procedures and solve problems involving decimals more effectively (Ball, 1993; Hiebert & Wearne, 1986; Hiebert, Wearne, & Taber, 1991; Lesh, Post, & Behr, 1987; National Research Council, 2001).

The Area Model

Research indicates that the area model provides a powerful context for students' early work with fractions and decimals (Behr & Post, 1992; Cramer, Post, and delMas, 2002). In particular, the use of fraction circle pieces and rectangles helps students establish ideas about equal parts, relative sizes of fractions, equivalent fractions, unit wholes, and operations on fractions. Children build on their everyday experiences (cutting toast, sharing pizza, etc.) when they use such models. Many real-life measurement situations (cooking, sewing, carpentry) involve fractions that are similar to fractions modeled with rectangles or circles. In this unit, area models are adapted specifically for decimal fractions. Fraction circle pieces representing fifths and tenths are used to build number sense of decimals to the tenths place. Tenths Helper charts and Hundredths Charts allow students to model decimals using rectangles.

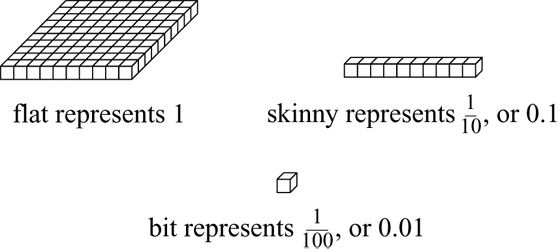
Students also use base-ten pieces to model decimals to the hundredths place, but with an important change. When modeling whole numbers with base-ten pieces, the unit whole is represented as a bit. When modeling decimals, however, the unit whole is represented by a flat. Consequently, a skinny now represents 1/10 (0.1) and a bit 1/100 (0.01). See Figure 2. Students have completed many activities in which the size of the whole changes, including lengths on a number line and sizes of pizza slices, so this change is a natural extension of their understanding that the size of the whole changes in varied contexts. Using base-ten pieces in this way provides a connection point to existing understandings about place value with whole numbers. (Strictly speaking, base-ten pieces are a volume model for decimals and not an area model, but they can be interpreted as an area model when drawn on paper or viewed from above.)

The Number Line

When a decimal fraction is represented on a number line, it is understood to occupy a unique location along a continuous scale. Students' familiarity with ruler measurement, number lines, and base-ten hoppers makes this a useful model for understanding decimals. In this unit, students represent decimals on number lines by measuring lengths to tenths of a meter and hundredths of a meter in a lab context. Students also locate decimal fractions on scaled number lines and move “decimal hoppers” between number line locations. These activities establish number sense about how moving 1 unit along a number line compares with moving 0.1 units or 0.01 units.

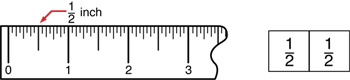
Students may have misconceptions about fractions on number lines because the focus shifts from an amount of area to a position on a number line. For example, in trying to locate 1/2 inch on a ruler, a child might become confused by thinking about 1/2 of the whole ruler. Furthermore, on a ruler or number line, the position is marked at the endpoint of the length. This is a departure from the area model, where labels are typically shown in the center to represent the entire quantity of area. See Figure 3. These differences may present some difficulty at first, but will deepen understanding as students grow accustomed to the features of each model.

Money

Money is a useful model for representing and operating on decimals for two reasons. First, money is commonly shown with decimals to the hundredths. Second, children typically have good intuition for situations involving money. Certain conventions in our way of writing money, however, make its use problematic for beginning instruction in decimals. For example, we usually write amounts of money using exactly two decimal places (e.g., $4.50, not $4.5). Pure decimal numbers do not have this constraint; 4.50 is as correct as 4.5. One can also think of an amount like $4.58 in terms of two distinct units, dollars and cents. In this interpretation, the number before the decimal point tells how many dollars, and the number after the decimal point tells how many cents, thus making it harder for students to see $.58 as a decimal fraction of a dollar and not simply as “58 cents.” It may be useful, therefore, to encourage students to interchange the terms “cents” and “hundredths of a dollar” to create a more general representation of decimals when modeling with money.