Building with Triangles

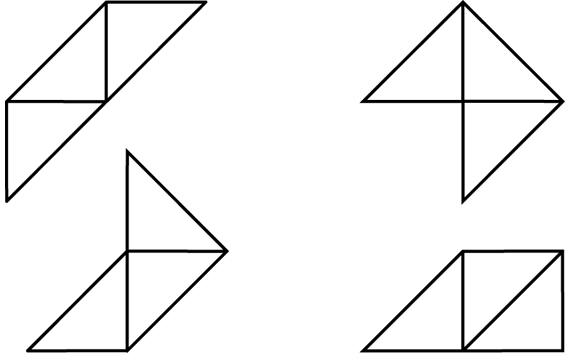
Question 8 in the Student Guide directs students to build all the shapes that can be made putting three triangles together edge to edge just as they did with two triangles. Ask students to repeat the basic steps in Question 6 using three triangles in their data collection. Figure 7 shows the four shapes that can be made with three triangles.

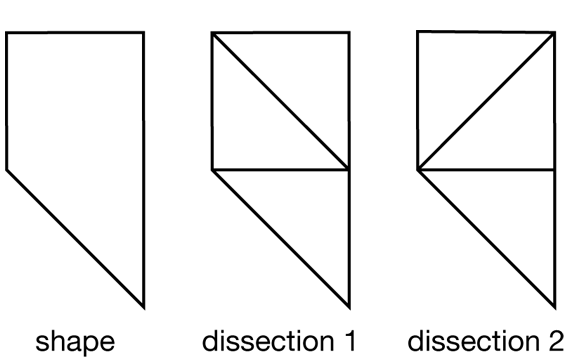
The three-triangle case is not much different from the two-triangle case. One new wrinkle is that one of the shapes can be dissected in two different ways. Figure 8 shows the shape and both dissections. For simplicity, we want to consider these shapes to be the same, just as we consider flips and turns to be the same. Your students may not raise this point, and we recommend not bringing it up if it is not mentioned. The important thing to stress is the outline of the shapes; the interior triangles do not matter.

Question 9 in the Student Guide asks students to share a pattern they noticed in their table. Students should notice a relationship between number of sides and number of vertices (e.g., 4 sides, 4 vertices).