Median or Mean? For many data sets, the median and the mean will be very close to one another. In these cases, the choice of the mean or median may depend on the ease of the calculations. The median is often easier to find, and for this reason, students have used it successfully to average data collected in labs in grades 1–3 and can continue to do so in grade 4. We introduce the mean because, although it is harder to compute, it is the most commonly used average and it often represents the data better than the median. Statisticians call both the median and mean measures of central tendency because they both give information on the values in the middle of the data set.
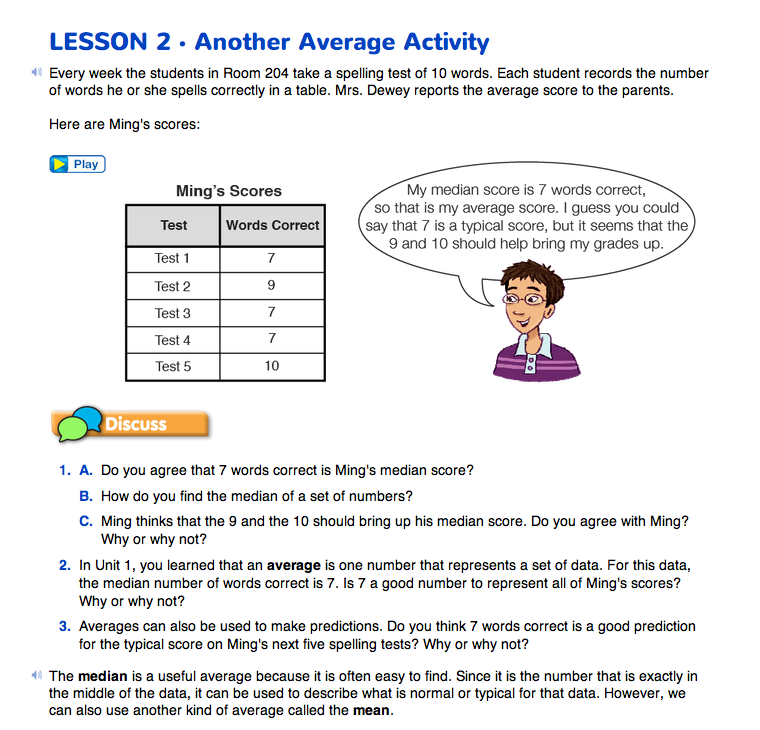
When calculating the mean, every value is involved in the computation. To find the mean of Ming's scores, add the scores and divide by the number of scores: (7 + 9 + 7 + 7 + 10) ÷ 5 = 8. The scores of 9 and 10 pulled his average up. When finding the median, individual values are not taken into account. Ming's median score is 7 because it is the middle value when the scores are ranked in order from smallest to largest. The 9 and the 10 are not taken into account.
Students do not need to master the subtleties of the differences between means and medians at this time. Our intent is to give students experience using both, so that they can use one or the other when they collect data for labs and activities.
Finding Medians and Means. Begin by asking students to read the opening vignette on the Another Average Activity pages in the Student Guide. Use Questions 1–3 to discuss Ming's spelling test scores, review medians, and develop a context for learning to use the mean to find an average.
After reading about mean in the paragraph following the questions, have students build towers of cubes to represent Ming's five spelling tests. See Figures 2 and 3. The number of cubes in each tower corresponds to the number of correct words on each test.
- What does each stack represent? (One test score)
- What does each cube represent? (One word spelled correctly)
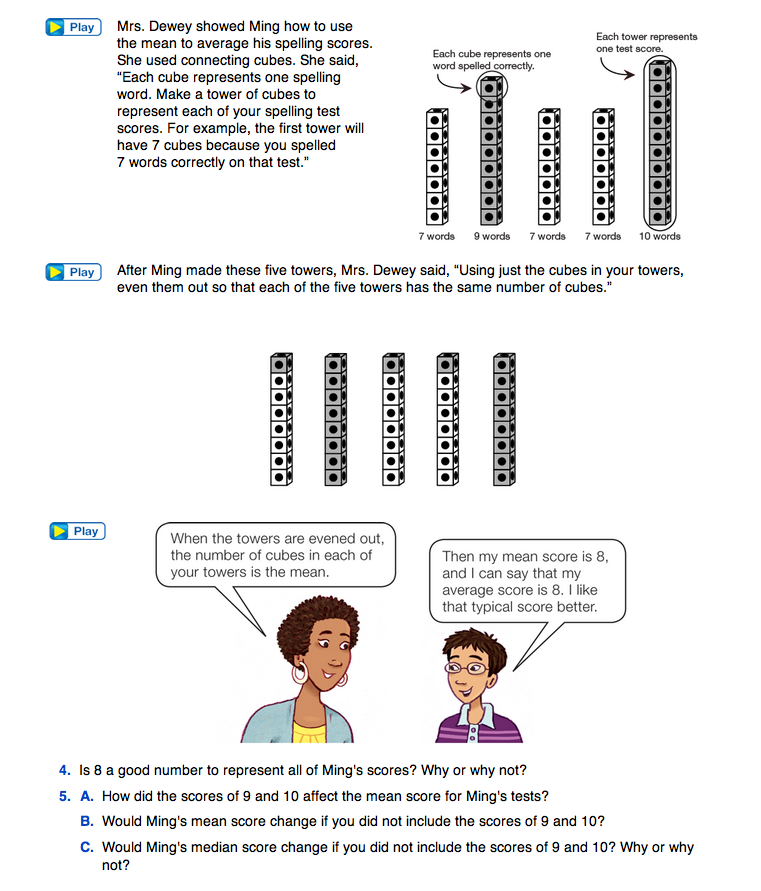
If possible, ask students to use one color for each tower. Building each tower with a single color of cubes helps students see how averaging is an “evening out” of Ming's test scores. For example, the first test can be shown with 7 red cubes, the second with 9 blue cubes, the third with 7 green cubes, the fourth with 7 yellow cubes, and the fifth with 10 brown cubes. This will show clearly how cubes are moved from the taller to the shorter towers to even out the number of cubes in each tower.
Then have students “even out” the five towers, moving cubes from the higher towers to the shorter ones. The height of the “evened out” towers is the mean score.
- What happens when we “even” the stacks out? (The cubes from the higher stacks fill in the shorter stacks, to make 8 cubes in each stack.)
- Do we still have the same total number of cubes? (Yes)
- Do we still have the same number of words spelled correctly? (Yes)
- How many total words did Ming spell correctly on his five tests? (40 words)
- If he had spelled the same number of words correctly on each test, how many would that be on each test? (8 words)
- How many cubes would be in each of the 5 stacks? (8 cubes each)
Continue reading the vignette and use Questions 4–5 to discuss how well the mean represents the scores.
Question 4 asks if 8 is a good number to represent all of Ming's spelling scores. Although Ming never spelled 8 words correctly on any of the tests, 8 does fairly represent all of the scores. Ming spelled a total of 40 words correctly on all five tests, so if he spelled the same number of words correctly on all the tests, each of his scores would have been 8. Evening out the towers illustrates this point without using division to find the mean.
Question 5 asks how the scores of 9 and 10 affected the mean score. Taking the cubes from the towers with 9 and 10 cubes and placing them on the towers with 7 cubes increases the number of cubes in those towers to 8 cubes. This shows how the higher scores increase Ming's mean score. However, Question 5C shows that higher (and lower) scores often do not affect the median in the same way.

Building Towers or Calculating Averages? The method of using manipulatives to find the mean helps students understand the meaning of the mean and how it is calculated. By redistributing cubes to even out towers, students make sense of the mean as the number that results from “evening out” all of the data values without changing the total quantity represented in the data. It is important that students establish this intuitive understanding before numerically computing the mean, even if numerical computations can be done more quickly. Students will have an opportunity to calculate means using numerical methods in Lesson 3.
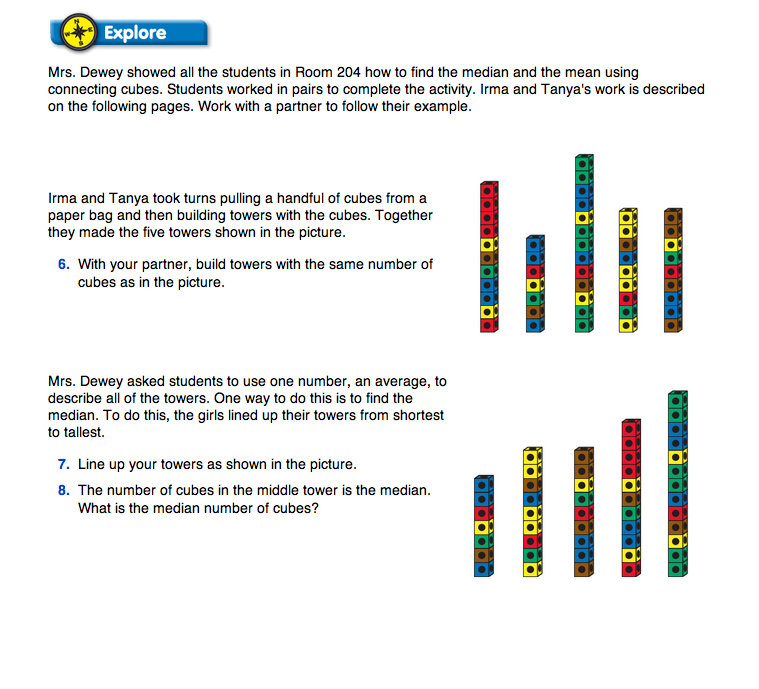
Exploring Averages. Have pairs of students imitate the actions of the two girls described in the Explore section on the Another Average Activity pages. Students should build towers with the same number of cubes as shown in the illustrations.
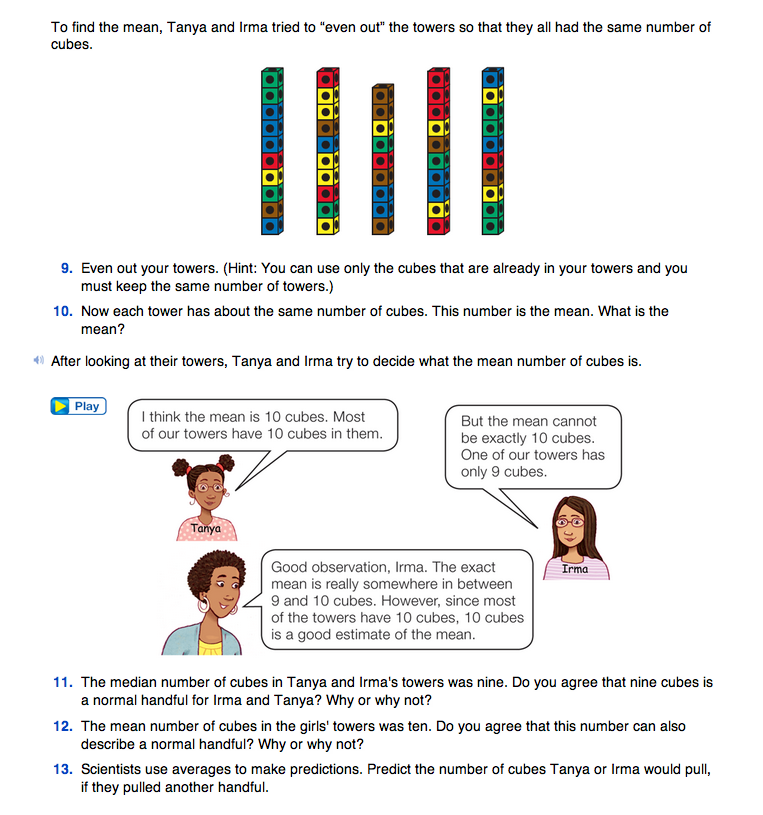
Students find the median by lining up the towers from shortest to tallest as described in Questions 6–8. Questions 9–10 guide students through finding the mean with the towers of cubes. They remove individual cubes from the taller towers and place them on the shorter towers until all the towers have about the same number of cubes.
- How many cubes are now in each tower? (Four towers have 10 cubes and one tower has 9 cubes.)
- Can we make all the towers exactly even? (No)
- However, most of the towers have the same number of cubes. Which number, 9 or 10 cubes, is the best choice for the mean? Why? (10 cubes, because there are more towers with 10 cubes than there are with 9 cubes.)
Ten cubes approximate the mean since most of the towers had 10 cubes. This is equivalent to rounding the mean to the nearest whole number. Questions 11–12 draw attention to both the median and the mean as reasonable representative numbers for Tanya and Irma's handfuls of cubes. Question 13 points out the uses of averages to make predictions.
The class may need to define “handful” since children often think bigger is better. Guidelines similar to the following may be helpful: Grab the cubes with one hand without touching the bag with the other hand; and shake your hand gently before taking it out of the bag.
Question 14 instructs students to repeat the same activity as Tanya and Irma. They pull handfuls of cubes out of a paper bag and build a tower with each handful. Each pair of students makes a total of five towers. Remind students to build each tower with just the cubes from one handful and not to supplement with more cubes from the bag. To find the median number of cubes in a tower, students line up the towers from smallest to largest. The number of cubes in the middle tower is the median. To find the mean, they “even out” the towers until each tower has about the same number of cubes. The mean is the number of cubes in each tower after the cubes have been rearranged.
- If you were to tell a friend about how many cubes you usually pull in one handful, what number would you use? (Answers vary based on students' towers. However, using the mean or median shows an understanding of the use of averages.)
- Why did you choose this number? Is this number your mean or your median?
- Is an average a good number to use to describe a normal handful? (Yes)
See the Sample Dialog to help guide class discussion in helping students clarify any confusion when determining the mean.

Use the following dialog to help students understand the ideas in Question 14.
Teacher: When I walked around the room, I saw some confusion with finding the mean, but I also heard some good conversations. Can someone share something that happened in their group? Yes, Romesh.
Romesh: We built the five towers. Then we tried to even the towers out but there wasn't enough cubes to make them all even. So Jerome wanted to go into our bag of extra cubes so we could even out the towers.
Teacher: What did you tell him?
Romesh: I told him he couldn't do that.
Teacher: Why not? Why can't Jerome even the towers out with extra cubes?
Romesh: Because we are supposed to stick with the cubes we pulled out. I told him to pretend they were cookies. We for sure wouldn't be able to go back and get more cookies from the cookie jar.
Teacher: That's right. Jerome, think back to Ming's test scores. What did the cubes in Ming's towers represent?
Jerome: They represented words he got right on his tests.
Teacher: If Ming's towers weren't even, what if he wanted to get more cubes to make them even?
Jerome: He couldn't do that because he only got that many words right.
Teacher: And if he did take more cubes from his bag, what would that do to his problem?
Jerome: It would be like him saying that he got more words right than he did.
Teacher: So when he is finding the mean, he has to stick with what he's got, doesn't he?
Jerome: Yes.
Romesh: That's what I told him. We can only use what we got in our five handfuls.
Teacher: Good. Ana, do you want to tell what happened in your group?
Ana: We built our five towers. One just had two cubes in it, so when we tried to even them out, we just took that tower apart and put the cubes onto other towers.
Teacher: So then how many towers did you have?
Ana: Four. But that wasn't right.
Teacher: Why not?
Ana: Because our towers were supposed to be like our handfuls. We had five handfuls so we had to have five towers.
Teacher: Jerome, what do you think about what Ana's group did?
Jerome: It's kind of like what I did. You have to stay with what you got. I couldn't get more cubes and Ana couldn't make four towers when she was supposed to have five.