Set Up Hundreds of Coins. This activity involves
collecting pennies, nickels, dimes, and quarters in
four coin jars. It will take part of one session to
introduce the activity and a couple of minutes during
each ensuing day. Set aside the same time each day,
such as the start of the day or the beginning of math
class, to work on the activity.
One benefit of a long-term project is to encourage students
to think of mathematics as more than a series of isolated
problems, each of which can be solved in a few minutes.
Hundreds of Coins requires the students to work on a
long-term problem each day throughout most of the year.
Place one penny in each jar every day and record the
new total in the next empty space on the displays of
the four coin charts. As the jars collect more pennies,
trades are made for coins of higher value, but the
total amount of money remains the same for the
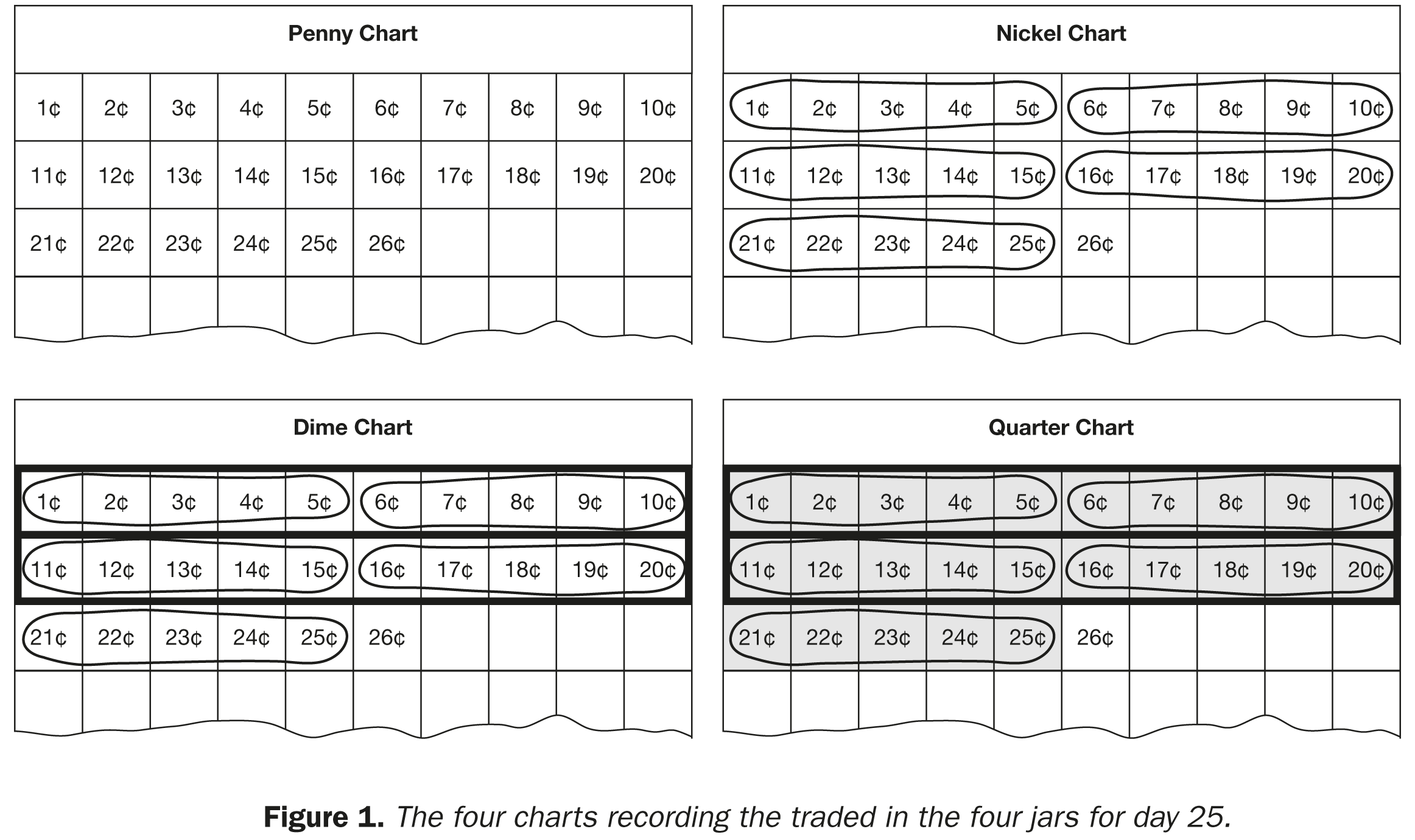
four jars. Figure 1 shows the recorded totals up to
and including Day 26 on all four charts. Make trades
as follows:
- The first jar will always contain only pennies.
- The second jar will contain only pennies and
nickels. Whenever five pennies accumulate in the
jar, make a trade for one nickel. The total value
remains the same as the Penny jar. Whenever five
pennies are traded for one nickel, draw a circle
around the five penny increments on the Nickel,
Dime, and Quarter Charts. See Figure 1.
- The third jar will contain different combinations
of pennies, nickels, and dimes. Make trades of
pennies for nickels as in the second jar, but when
two nickels accumulate, trade them for one dime.
Whenever coins are traded for a dime, you will
be at the end of a row on the charts. On the Dime
and Quarter Charts, draw a solid black line
around that row, making a rectangle as shown in Figure 1.
- The fourth jar will contain different combinations
of pennies, nickels, dimes, and quarters. Make
the trades for nickels and dimes as in the second
and third jars, but when two dimes and one
nickel accumulate, make a trade for a quarter.
Whenever coins are traded for a quarter, shade in
the 25 spaces on the Quarter Chart with a highlighter.
Day 1. To start the activity, explain that students will
work with money each day of school for 100 days.
Ask them if they can predict when the 100th day of
school will occur.
Show the classroom calendar and
ask questions like:
- What is today's date?
- What day of the week is it?
- How many days are in a week?
- How many days do we come to school this week?
- How many days are in this month?
- How many days will we come to school this
month?
- Which days of the week do we usually come to
school?
- Which days of the week do we stay at home or
which days do we usually not have school?
- What day of the week is one week from today?
- What will the date be in one week? How do you
know?
- Will we be in school on that date? Why do you
think so?
- How many days are in next month? How do you
know?
- How many days will we be in school next month?
- What patterns do you see in the calendar?
Show the four jars and tell students that they will
add one penny to each jar every day for the next
100 school days. Hold up the jar labeled "Penny."
Show students the jars labeled "Nickel," "Dime," and
"Quarter."
Have a student place a penny in each jar as shown in
Figure 2.
- How many pennies are in each jar? (1)
- How much money is in all four jars together?
(four cents)
- How much money will be in each jar after
three days? (3¢)
- How can we find out how much money that will be
in all? (Skip count by threes four times: 3, 6, 9,
12.)
- What number sentence shows the value of the
coins if there is 3¢ in each jar?
(3¢ + 3¢ + 3¢ + 3¢ = 12¢)
- How much money will be in each jar after
100 days? (one hundred cents)
- How much money will that be in all? (four hundred
cents, or four dollars)
All students' predictions should be accepted into the
conversation. You may want to record students'
ideas for comparison later in the school year.
Show the four coin charts. Tell students that these
charts are for recording the money they put in the
jars each day. As a student drops a penny in each jar,
write "1¢" in the top left space of each chart. Ask students
if these charts remind them of anything they
used in First Grade. As students respond, listen to
their ideas, but don't comment one way or another
about what the chart will eventually look like.
Day 2. As a student drops a penny in each of the
four jars, ask another student to record the value of
the money in each jar (2¢) in the next empty space
on the corresponding chart. See Figure 1.
- How much money is in each jar? How much
money is recorded on each chart? (2¢)
- How much money is in all four jars? How do you
know? (Possible response: 8¢. I skip counted by
twos: 2, 4, 6, 8.)
- What is a number sentence that shows the value
of the coins in the four jars?
(2¢ + 2¢ + 2¢ + 2¢ = 8¢)
- How many 2s do we have? (4)
- Four 2s equal how much? (8)
Day 3 and 4. Do the same as Day 2, except write
"3¢" and "4¢" in the next spaces on each of the charts.
While this unit does not introduce multiplication formally,
the repeated addition activity lends itself to the use of multiplicative
language. Saying that "four twos equal 8" is easy
for students to see and understand in this context, particularly
when they are looking at the repeated addition sentence.
Using this language with repeated addition will help
students understand what is happening mathematically
when they begin learning the multiplication operation.
Day 5. This day will take a slightly longer session.
First, repeat the activities of previous days, dropping
a penny into each jar and writing "5¢" in the next
space on all 4 charts. Then tell students that they will
be making trades in all the jars except the one that is
just for pennies.
- Who can tell me what can be traded for 5 pennies?
(a nickel)
Have three students take five pennies out of the
Nickel, Dime, and Quarter jars and trade them for a
nickel. See Figure 3. Point to the first five spaces on
the Nickel Chart. Tell students that these five pennies
have now been traded for one nickel. With a
colored marker, draw a circle (oval) around the first
five spaces as shown in Figure 1. Do the same for
the first five spaces in the Dime and Quarter Charts.
Do not draw the circle on the Penny Chart since
those pennies have not been traded for a nickel.
- Do all the jars have the same number of coins?
(no)
- Do all the jars have the same amount of money?
(yes) How do you know?
- How much money do we have in each jar now?
(5¢)
- How much money do we have altogether? (20¢)
How do you know? (Possible response: I skip
counted by fives for each jar: 5, 10, 15, 20.)
- How can we write this as a number sentence?
(5¢ + 5¢ + 5¢ + 5¢ = 20¢)
- How many 5 cents (fives) do we have now? (4)
- So, when we have 4 fives, how much do we have?
(20)
Day 6–9. Repeat the procedure as in days 3 and 4
adding a penny to each jar and writing the new total
in the next space. Ask whether any trades can be
made.
Use the Day 8 Trade for Nickels section of the Hundreds of Coins Assessment Masters to assess students' abilities to
identify the relationships between pennies and nickels [E4].
Day 10. Have students add a penny to each jar and
write the new total on the charts.
- Can we make a trade? (Yes, we can trade
five pennies for a nickel in the Nickel, Dime, and
Quarter jars.)
Have three students make the trades. Ask them to
show their trade by drawing a circle around the
five pennies on the chart corresponding to their jar.
Ask questions similar to those for Day 5.
If no one
suggests making a further trade, ask students if they
can think of anything else to do with the coins.
- Can anyone think of another trade we could make?
(Trade two nickels for one dime.)
Tell students that the nickels in the Nickel jar will not be traded—that jar is just for nickels and pennies.
Have two students come up and remove the
2 nickels from the Dime and Quarter jars and replace
them with one dime in each jar. See Figure 4. Draw
a dark rectangle around the top row (first 10 cents)
of the Dime and Quarter Charts as shown in Figure 1.
- How many coins are there in each jar now? (The
Penny jar has 10 coins, the Nickel jar has 2 coins,
the Dime and Quarter jars each have one coin.)
- Is there still the same amount of money in all
four jars? (yes) How do you know?
- How much is in each jar? (10 cents) How much is
in all four? How do you know? (Possible
responses: I started with the dimes. Two dimes is
20¢. Then there were two nickels in the nickels
jar, so I counted 25¢, 30¢. Then I counted on the
pennies 31¢, 32¢ … 40¢. A second possible
solution is to count by tens to 40¢.)
- Can you show me the amount of money in one jar
on the number line?
- Show the amount of money in all four jars together
on the number line.
- Skip count by 10s and write a number sentence.
(10, 20, 30, 40; 10 + 10 + 10 + 10 = 40)
- How many tens do you need to make 40? (4 tens
equal 40.)
- How many cents are on each row of the chart?
(10¢)
- What do these charts remind you of now?
At this point, students may recognize the 100 Chart
from First Grade.
- Can you predict how many more days it will be
before we make the next trade for nickels? (We
will trade for nickels in 5 more days.)
- What number will we add to the chart on that day?
(15)
- Can you point to where it will be on the chart?
- On the number line?
- How much money will be in each jar then? (15¢)
- Can you predict when we'll make the next trade for
dimes? (in ten days)
- Where will that be on the chart? (down one row)
- On the number line? (on number 20)
Day 11–14, 16–19, 21–24. Add a penny to each jar
and write the totals in the next spaces on the charts.
Ask a few questions as appropriate. See Figure 5.
Day 15 and 20. Repeat Days 5 and 10, trading five
pennies for one nickel, and two nickels for one dime
as appropriate. See Figure 6. Continue asking students
to skip count by 5s and 10s to find the totals
and write number sentences. As students skip count,
point to the numbers on the number line and have
student volunteers point to the numbers on the
charts. Continue using multiplicative language
where appropriate.
Use the Day 20 Coins in Pockets section of the Hundreds of Coins Assessment Masters to assess students' abilities to
use mental math strategies and reasoning strategies (e.g.,
using doubles, making ten) to solve addition and subtraction
problems within 20 [E2].
Revisit prompts similar to the following from time to time for
Days 15–100:
- When we add the pennies to the jars today, how much
money will each jar have?
- Will we make any trades?
- Which trades? Why do you think so?
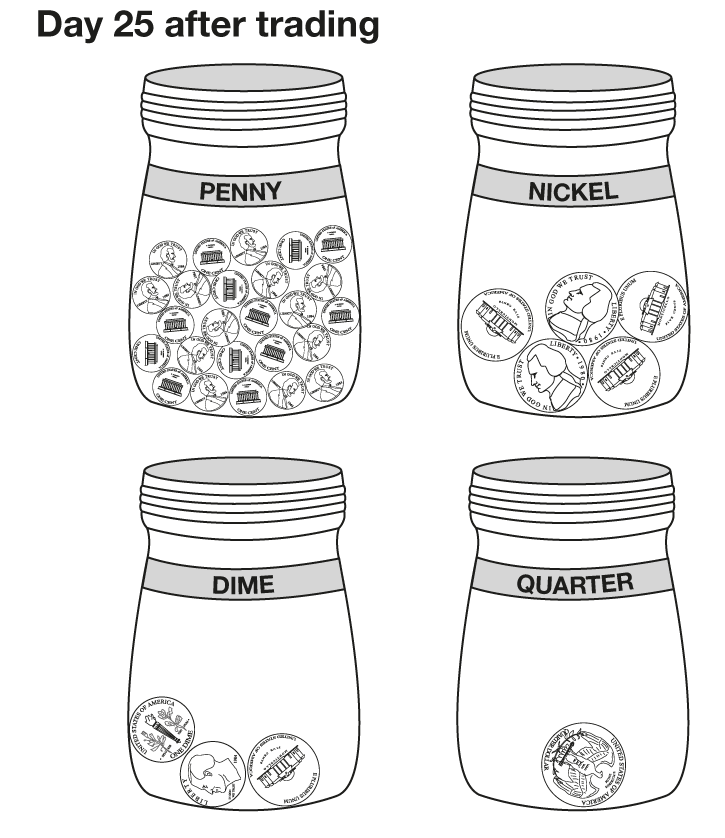
Day 25. This day will also require a slightly longer
session.
Before the coins are dropped into the jars,
ask the students to make predictions:
- When we add the pennies to the jars today, how
much money will each jar have? (25¢)
- Will we make any trades? (yes)
- Which trades? Why do you think so? (There are
4 pennies in each jar now, so one more penny
makes a trade of a nickel in the Nickel, Dime,
and Quarter jars.)
- After we make all our trades, which jar will have
the most coins? (Still the pennies because they
never get traded for a bigger coin.)
- Which will have the most money? (They will all
have the same amount.)
- How many coins will the Penny jar have?
(25 pennies)
- How many coins will the Nickel jar have?
(5 nickels)
- How many coins will the Dime jar have? (2 dimes
and 1 nickel)
- How many coins will the Quarter jar have?
Students may suggest that another trade can be made
with the coins in the Quarter jar. If not, ask probing
questions about other coins students may know
about and guide the discussion to trading the
two dimes and one nickel for one quarter.
See Figure 7.
- Which will have the fewest coins? (the Quarter
jar)
Discuss patterns that students see using the charts.
Ask them to use these patterns to make predictions.
- How many pennies will be in the Penny jar ten
days from now? (35 pennies)
- How many on the 38th day? (38 pennies)
- How many pennies will be in the Nickel jar on the
38th day? (3 pennies) How many nickels? (7 nickels)
How do you know? (If I go down one row on
the nickel chart that will be 35. 25 to 35 is ten
more, that's 2 more fives. So 5 nickels and 2
more is 7 nickels, then the 3 more pennies will be
38¢ on Day 38.)
- What trades will be made on the 40th day? (Trade
5 pennies for a nickel in the Nickel jar, a nickel
and 5 pennies for a dime in the Dime jar, and
5 pennies for a nickel in the Quarter jar.)
- What trades on the 45th day? (Trade 5 pennies for
a nickel in the Nickel and Dime jars, and 5 pennies
for a nickel then 2 nickels for a dime in the
Quarter jar.) On the 47th day? (no trades) How do
you know? (All the trades on Day 45 will be a
nickel, so Day 47 is just two more pennies in
each jar. There will not be any new nickels or
dimes yet.)
- When is the next day pennies will be traded for
nickels? How do you know? (Possible response:
Day 30. When I count by 5s, I get to 30 next.)
- When is the next time nickels will be traded for
dimes? How do you know? (Possible response:
Day 30. When you count by 10s, you get to 30
next.)
- When is the next time nickels and dimes will be
traded for a quarter? How do you know? (Possible
response: Day 50. Two dimes and one nickel
make 25¢ and 25 + 25 = 50.)

Use the Day 25 Sandy's Pennies section on the Hundreds of Coins Assessment Masters to assess students' abilities to
identify the relationship between pennies and nickels [E4]
and find the value of a collection of pennies, nickels, and
dimes [E5].
Use the Day 36 Spilled Pennies section of the Hundreds of Coins Assessment Masters to assess students' abilities to
use mental math strategies and reasoning strategies (e.g.,
using doubles, making ten) to solve addition and subtraction
problems within 20 [E2] and identify the relationship
between pennies and nickels [E4].
Use the Day 50 Coin Purses section of the Hundreds of Coins Assessment Masters to assess students' abilities to
find the value of a collection of pennies, nickels, dimes, and
quarters [E5].