Use Ten Frames, Number Lines, and Part-Whole
Diagrams. Read and show students the problem
about monkeys and lions you have written on the
display of the Show a Word Problem Master. Have
20 connecting cubes available for each student. After
students have had a chance to think about the problem,
ask different students to show how to use each
representation (ten frames, number lines, and part-whole
diagrams) to solve the problem posed. See
Figure 1.
- What is the problem? (Possible response: I need
to figure out how many animals there are altogether.)
Underline the question in the problem. Ask:
- How did you decide where to put the numbers in
the part-whole diagram? (Possible response: The
problem is about 2 parts being put together so I
put the parts in small boxes to then make a larger
whole.)
- How can the number line help you solve the problem? (Possible response: The number line helps
keep track of the numbers as I counted on.)
- How can the ten frame help you solve the problem? (Possible response: The ten frame helps me
see the fives and tens. When adding 8 and 4, I
could see the ten and knew the answer was 2
more than 10.)
- Did anyone use a different tool or strategy? What
did you use? (Possible response: I used connecting
cubes to act out the problem and then
counted all the cubes; I did not need to use any
tools. I know 8 + 4 = 12.)
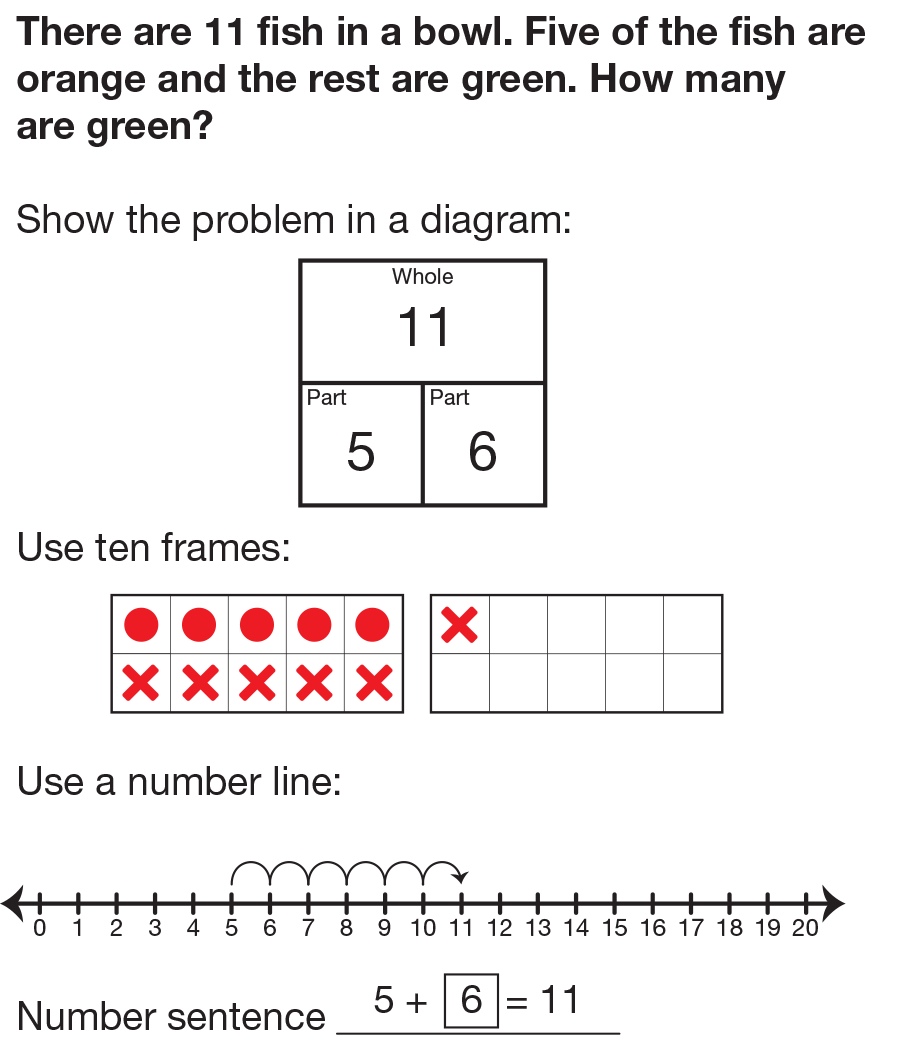
Continue the discussion with the fish bowl question
you prepared on the display of the Show a Word Problem Master. See Figure 2.
- What is the problem? (Possible response: I need
to figure out how many fish are green.)
- How did you decide where to put the numbers in
the part-whole diagram? (Possible response: In
this problem, the whole is given so I put 11 in the
"whole" box and one of the parts in the smaller
boxes. I needed to find the other part.)
- How can the number line help you solve the problem?
(Possible response: The number line helps
keep track of the numbers as I counted up from
5 to 11. I then went back and counted the hops.)
- How can the ten frame help you solve the problem?
(Possible response: I filled in 5 and could
"see" the 11 and knew that I needed 5 and 1 or 6
to make 11.)
- Did anyone use a different tool or strategy? What
did you use? (Possible response: I know
5 + 5 = 10 so 5 + 6 = 11. I did not use any of
the tools; I used connecting cubes to act out the
problem. I counted 5 and kept adding cubes to 11
and then counted the cubes that I added.)

Students used these tools throughout First Grade. This quick
review on how to use ten frames, part-whole diagrams, and
number lines to solve addition and subtraction problems may
be sufficient. These tools support student reasoning and
explanation of their reasoning. Create additional problems if
students need a more extensive review of these tools.
Explain to students that they can use more than one
tool or strategy to solve each problem and that they
are not limited to the ten frames, number lines, and
part-whole diagrams. It is important to ask students
to explain their thinking and help students who have
misconceptions about the correct ways to use tools
and strategies. Use Sample Dialog 1 to guide your
discussion of using tools to solve problems.

Use the sample dialog to guide your discussion of the sample
subtraction problem:
There are 11 fish in a bowl. Five of the fish are orange and
the rest are green. How many are green?
Teacher: Who can explain how to solve the problem?
Michelle: I used the number line. I started at 11 and counted
back 5: 11, 10, 9, 8, 7. The answer is 7.
Teacher: Does everyone agree that 7 is the answer?
Ana: I didn't get 7. I got 6. I used the number line, too, and I
counted back 5: 10, 9, 8, 7, 6. My answer is 6. When
you use the number line you have to count the hops or
the spaces. You start at 11 and the first hop is back to
10. Four more hops and you land at 6.
Teacher: Good thinking, Ana! It is important to count the
hops on the number line, so that you get the correct
answer. Did someone use a different strategy or tool?
Sam: I put 11 connecting cubes in one of the "part" boxes on
the part-whole diagram. I put 5 cubes in the other "part"
box. Then I put them together in the "whole" box and I
got 16.
Teacher: Let's think about your answer. Does it make
sense?
Roberto: That doesn't make sense. There are 11 fish in the
bowl. That number should go in the "whole" box. Then
move 5 to the "part" box because 5 of the 11 fish are
orange. The rest of the fish are green. There are 6 cubes
left, so 6 are green.
Teacher: Great, Roberto! It's important to look back at the
problem and see if your answer makes sense.
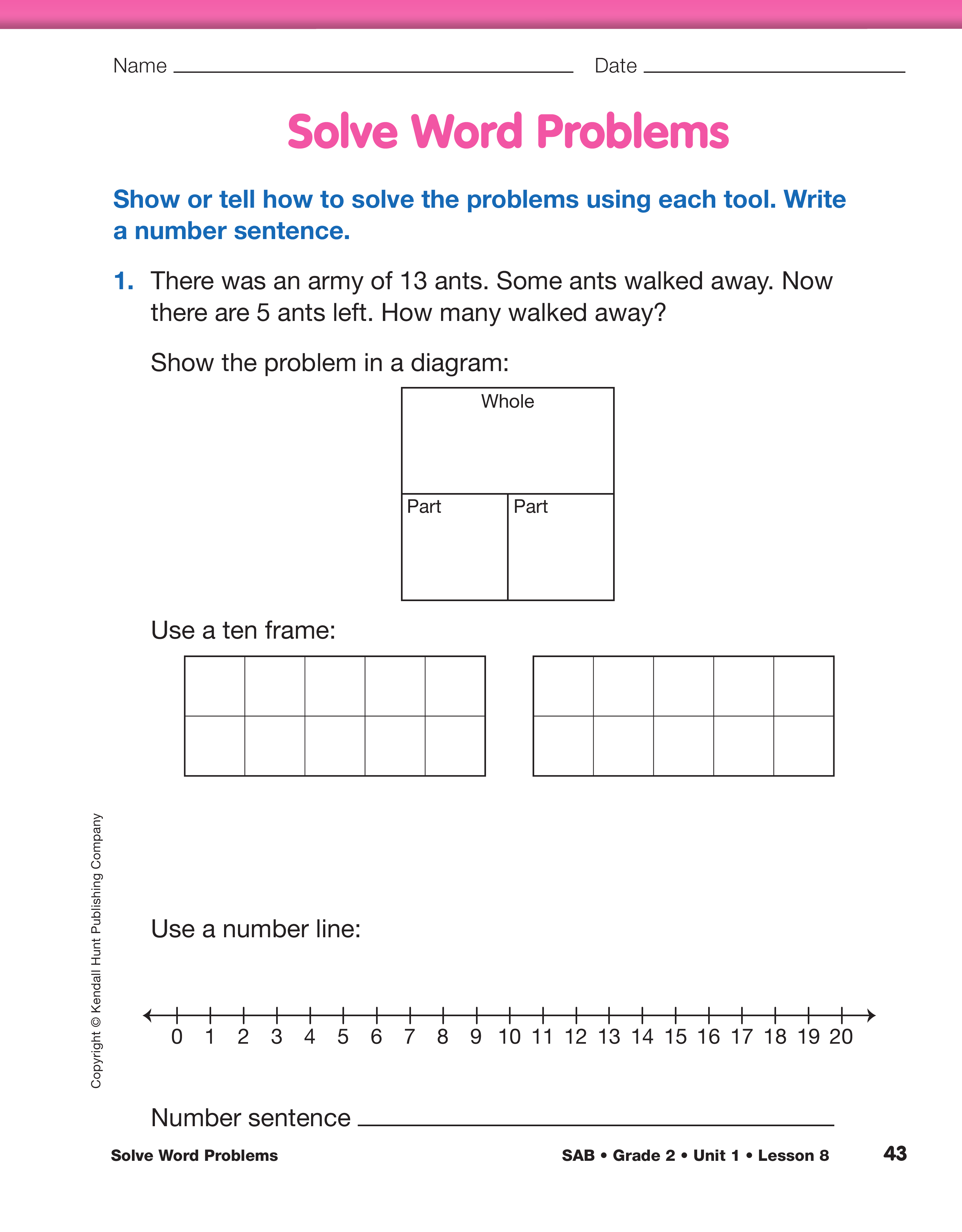
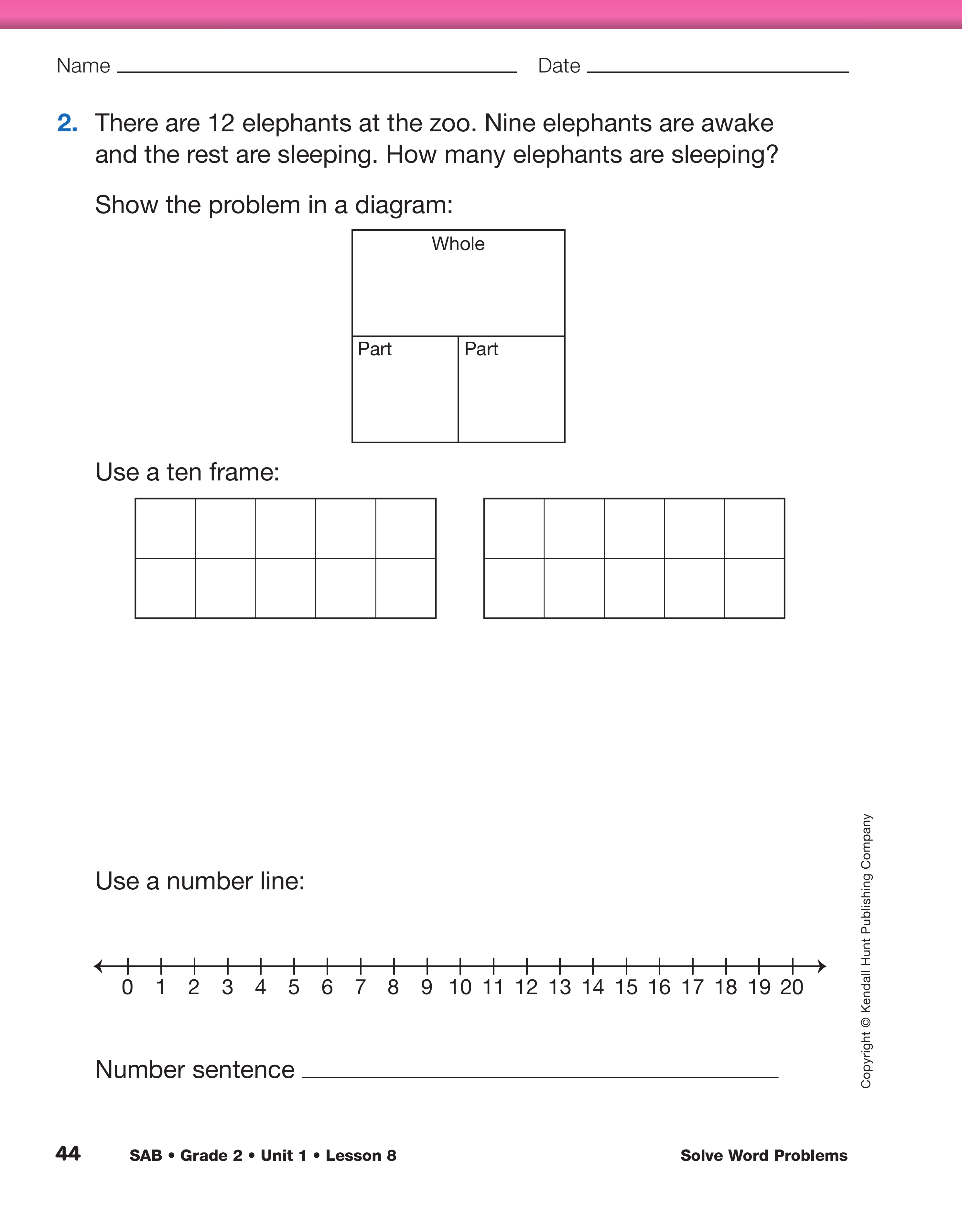
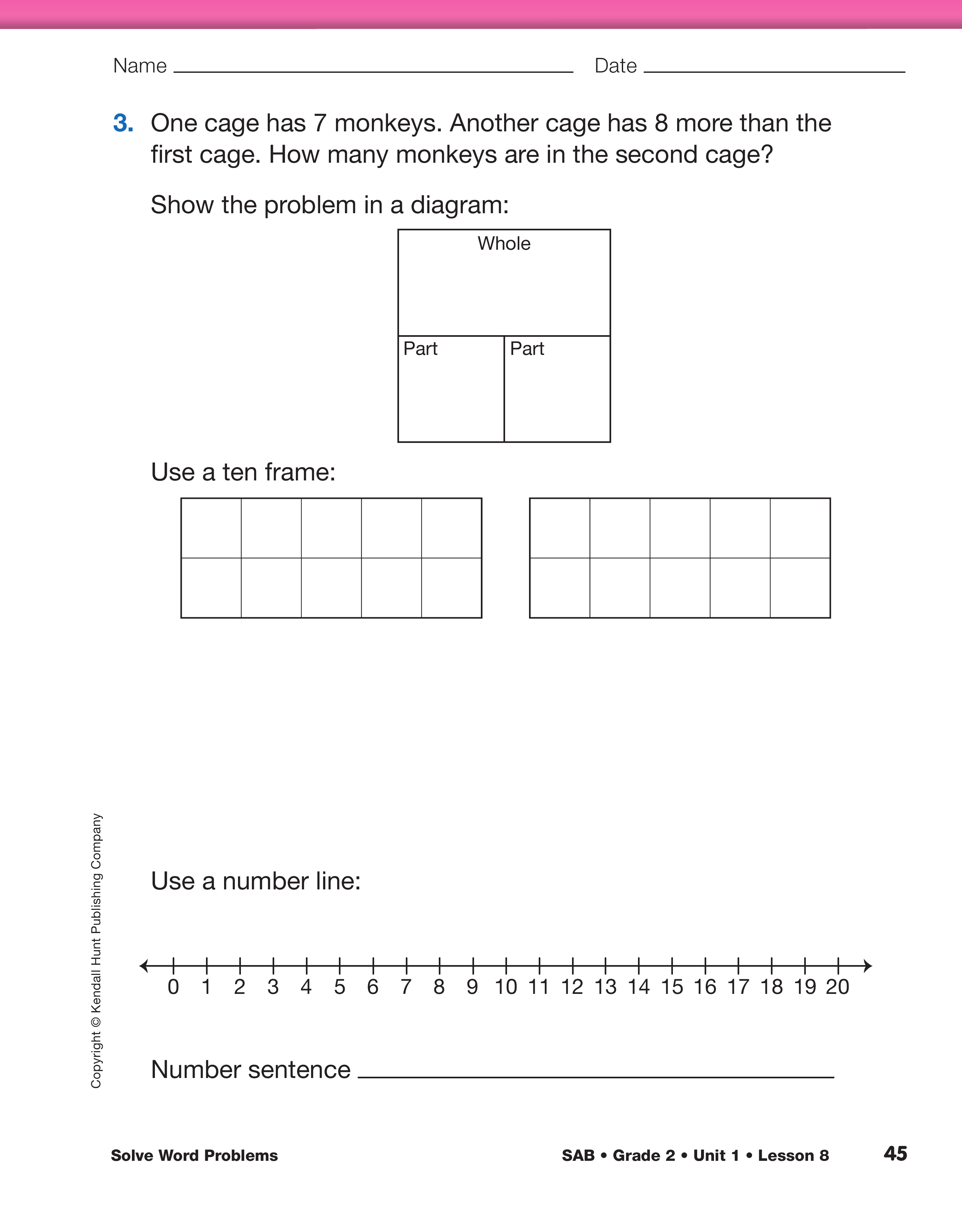
Practice Using Tools to Solve Word Problems.
Direct students to the Solve Word Problems pages in
the Student Activity Book. Tell students that they will
work with a partner to solve four problems in different
ways. Refer students to a display of the Math
Practice page.
- Which of these practices have we been focusing
on? (Possible response: Math Practice 2, Find a
strategy, and Math Practice 5, Show my work.)
Refer students to Math Practice 1, Know the problem.
- What can you do to figure out what the problem is
asking? (Possible response: Draw a picture of the
problem, act out the problem with my partner or
with the connecting cubes, underline the question,
find the information I need to answer the
question.)
- Record student ideas on the board. See Figure 3.
- These problems are about ants, elephants, monkeys,
and dogs. What can you do if you do not
know a word in a problem? (Possible response:
Read through the word and see if you can figure
out what the word is or might be; replace the
word with a word you know that makes sense;
act out the words you know to help you figure
out the words you do not know.)
Direct students to work with a partner to answer
Questions 1–4. While students are working, circulate
around the room. Listen to students' strategies
and note their confidence with various tools. Also
make note of how confidently students know the
problem they are solving. Choose one student pair to
show how to solve each of Questions 1–3. Provide
them with a display of the Show a Word Problem
Master to show their work. When most students are
ready, ask the chosen student volunteers to demonstrate
their solutions and explain how they used the
tools to solve the problem.
Use a display of the Show a Word Problem Master to
capture how Carla used the part-whole diagram in
Question 4. Ask students to use their thumbs to show
if they agree with Carla. Show a thumbs-up if they
agree and thumbs-down if they do not agree.
Ask
students to discuss this question with a partner:
- What might you say or ask Carla to help her? (Possible response: How can the whole be larger
than the part? What number would you add to 17
to make 8? Does that make sense?)
Ask students to share their ideas and then ask a student
to solve the problem on the display using each
of the tools shown.
- Does anyone have another strategy for this problem? (Possible responses: I know 8 + 8 = 16 so
8 + 9 = 17. So there were 9 dogs adopted; I
counted back from 17 to 8 and there were 9
hops.)