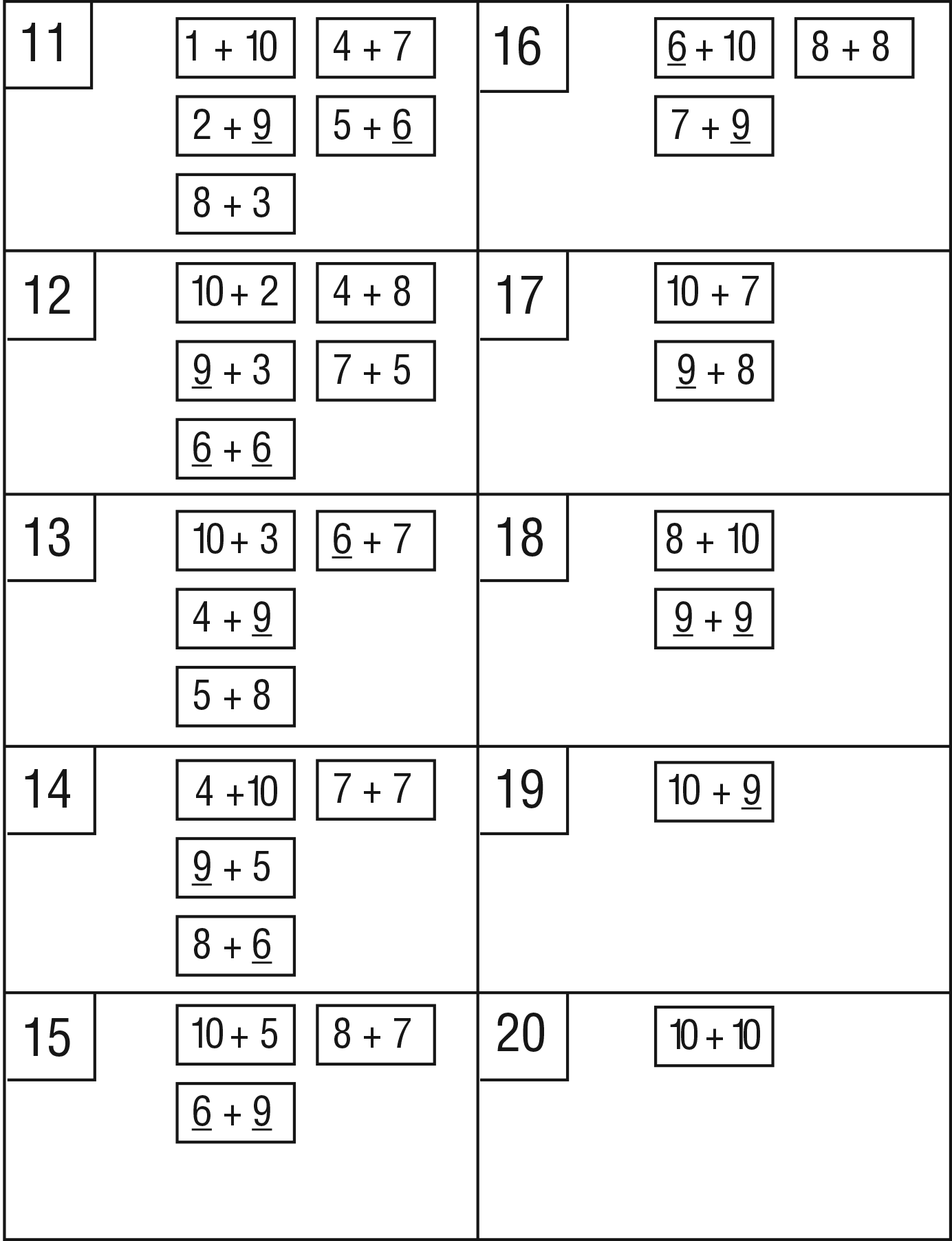
Show students the
Addition Cards for Larger Sums
1–3 and tell them they are going to work in pairs and
sort the cards by their sums as they did in Unit 6.
Remind students that a sum is the answer to an addition
problem or the result when two numbers are
combined. Use the
Sorting Mat for Larger Sums and
the prepared set of addition cards to model the activity.
See Materials Preparation. Encourage students to
choose tools (connecting cubes, number lines, ten
frames) and other strategies to help them sort the
cards.

Give students who may be overwhelmed with the task of
sorting all 30 cards a smaller set. Choose problems based on
students' needs. Here are some examples:
- For students who need practice counting on instead of
counting all, give them cards in which they will add on 1,
2, or 3.
- To review using patterns adding ten to a number, give
them cards with 10 + 1, 10 + 2, 10 + 3, etc.
- To encourage students to use doubles, give them a set of
cards that lend themselves to this strategy, i.e., 5 + 6,
6 + 6, 6 + 7, 7 + 7, 8 + 7, 8 + 8, 9 + 8, 9 + 10, and
10 + 10.
- For students who are having trouble "seeing tens"
so that they can use the making ten strategy, have the
students begin with the nines, i.e., 9 + 2, 9 + 3, etc.
Talk with student pairs as they work to assess their
reasoning and use of strategies for solving addition
problems. Use this opportunity to discuss their
strategies and prompt students to move to more efficient
strategies.
Use prompts similar to the following:
- I see that you used connecting cubes to add
9 + 2. Try using the number line to do that faster.
Where could you start? (Start at 9 and make
2 jumps.)
- You said you used doubles to add 5 + 6. What did
you mean? (Possible response: I know that
5 + 5 =10, so one more is 11.)
- [Tanya], can you use [Jacob's] doubles strategy to
solve 7 + 6?
- You said that 8 + 3 = 12. Tell me how you
solved it.
- When you solve 9 + 5, what do you see in your
head? Do you see a way to make a ten? (Possible
response: I think about the number line starting at
9. It takes one more to get to ten. Then I go 4
more to get to 14.)
When students have completed their charts, ask students
how they sorted the facts. Have individual students
place the addition cards on the class chart and
discuss the results. Use prompts to probe students'
thinking and move them toward using more efficient
strategies. Add new strategies to the Invented
Strategies chart.
- Do you see a ten in the problem? Show me.
- What is the partner number with 9 [8, 7, 6] that makes ten?
- How can you make a ten with 9 [8, 7, 6]?
- If you take [one] from _____ to make ten, what will
be left over to add to the ten?
- Does making a ten help you solve the problem? How?
- Is the fact near a double? What double?
- Is the sum more than the double or less than the double?
- Are you going to add [one] more or subtract [one] from the double?
Some students may already know the addition facts in this
lesson. As you discuss students' strategies for placing sums
in particular groups, challenge students to use the same
strategies with larger numbers and sums. For example, if
students can solve 9 + 5 or 8 + 5 and explain their strategy,
ask them to apply the same strategy to solve 29 + 5 or
18 + 5.