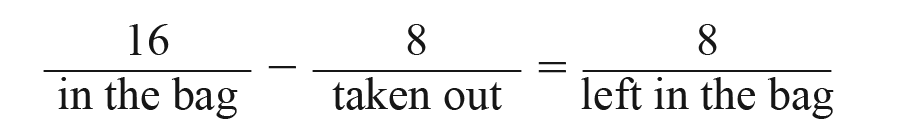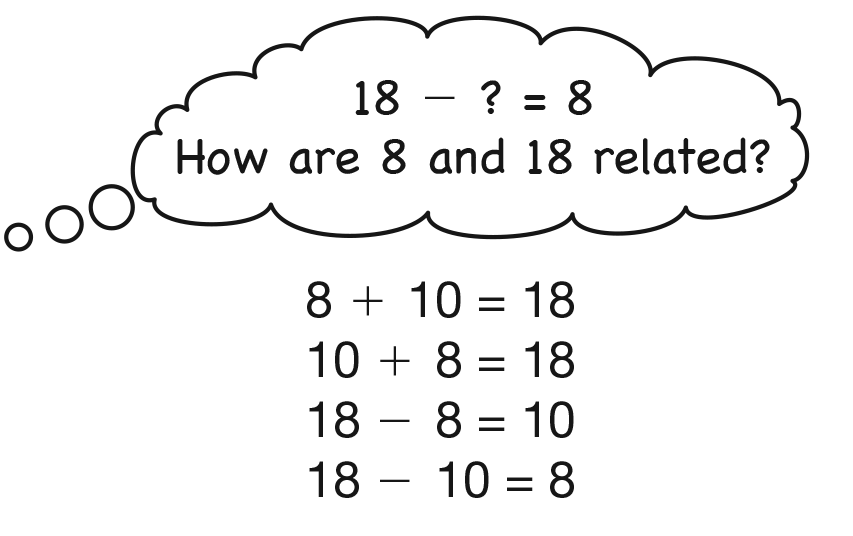Demonstrate the Game.
Tell students they are
going to play the game How Many in the Bag with a
partner. It's the same game they played in Unit 6
Lesson 8 but with larger numbers.
Review the rules of the game by playing a few
rounds with the whole class. Show students a bag
and tell them it contains 16 counters. Remove 8 and
place them on display.
- There were 16 counters in the bag. I took out 8.
How many are in the bag now? (8)
- What is your number sentence? (16 − 8 = 8)
- What was your strategy? (Possible response:
There were 8 counters outside of the bag so I put
8 in my head and counted up on my fingers: 9,
10, 11, 12, 13, 14, 15, 16. I counted 8 fingers so I
knew there were 8 counters in the bag.)
- Could you have solved it in a different way? How? (Possible response: I used the number line on my
desk. I started at 16 and went back 8 hops to
show that 8 were taken out of the bag. I landed
on 8.)
Display the Invented Strategies chart from Lesson 1 and ask:
- Should we add these strategies to the Invented Strategies chart?
Display and direct students to the first page of the
How Many in the Bag pages from the Student
Activity Book. Remind students how to complete the
number sentences by writing:
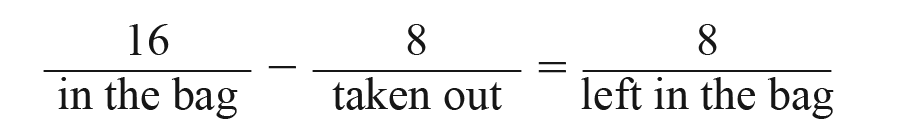
Repeat with one or two more numbers subtracted
from 16. Then change the starting number in the bag
and work one or two problems subtracting from that
number.
If no one suggests it, ask if they could use an addition strategy to solve a subtraction problem.
- Could you solve this problem using doubles? How? (Possible response: I know 8 + 8 = 16. If
there are 8 counters outside of the bag, there
must be 8 counters in the bag so that the total is
16.)
- Why do you think it works to use an addition strategy to solve a subtraction problem? (Possible response: Subtraction "undoes" addition.)
Remind students that subtraction is the reverse of
addition. Show an example with the counters on display.
Write the number sentences one above the
other.
- I have 8 counters and I add 8 more. Now I have 16. 8 + 8 = 16.
- I have 16 counters and I take away 8. I have 8 left. 16 − 8 = 8.
Play Round One.
Distribute one bag of counters per
student pair. See Materials Preparation. Students will
work with a partner to play the game and record
number sentences on the How Many in the Bag
pages. The number of cubes in the bag should be
between 11–19. If students take out the same number
of cubes that they had before, they should pull again.
Encourage students to use tools such as ten frames,
number lines, drawings, and part-whole diagrams
and any strategies they like.
Monitor students as they play and ask them to
describe their strategies as they work. Pay attention
to the variety of strategies and tools used so that you
can select some particular students to share after
round one. Use your conversations with students as
they play to note if they are using efficient strategies.
Are they using direct modeling, counting strategies,
or reasoning from known facts to solve the problems?
Because these are more challenging subtraction
situations, students may rely on counting all and
counting on. Help students who are using inefficient
strategies to try to move toward using strategies that
involve more reasoning. See Sample Dialog 1 for
examples in which Keenya used a combination of
direct modeling and counting. Michael used doubles
and reasoned from known facts.

Teacher: Who can tell me your last number sentence and
what strategy you used to solve a problem?
Keenya: Mine was 17 − 8. I started at 17 on the number line
and hopped back 8. It's 9.
Teacher: So, you counted back. That works.
Michael: I can do it faster. I used doubles. I know that 8 + 8 is
16 and 17 is one more than that. So if you took out 8 and
there were 16, then you would have 8 left. But there were
17 in the bag, so there's one more left, so it's 9.
Teacher: Good explanation, Michael. So what is your number
sentence?
Michael: 8 + 9 = 17.
Jackie: No, it's not. That's an addition sentence. It's supposed
to be subtraction. 17 − 8 = 9.
Teacher: Good point, Jackie. This is a take-away situation,
so it's subtraction. So then does that mean Michael's
number sentence is wrong?
Jackie: Well, maybe it's not wrong, but it's not right.
Michael: Yes, it is. 8 on the outside and 9 on the inside. That's
8 plus 9 and that's 17.
Teacher: Class, what do you think?
Nicholas: I think it's subtraction too, so I think Jackie's a little
more right than Michael. But Michael is right, too.
Teacher: I agree with you, Nicholas, that they are both
right. But when we're pulling counters out of the bag,
some people might think subtraction before addition,
like Jackie did. But the way Michael explained his
number sentence makes sense, too.
Let them play for about ten minutes.
Then stop the games. Have each student identify the
most recent problem he or she had to solve while
playing the game. They use these problems to complete
Question 1 on the How Many in the Bag pages
Stop and Discuss Strategies. Select students to
show or tell the strategies they used to solve their
last problem. Encourage them to show how they
used tools such as ten frames, number lines, drawings,
counters, or diagrams. Add any new strategies
to the Invented Strategies chart.
Use Fact Families.
To help students use fact families
to solve subtraction problems, ask questions
similar to the following:
- What was your last number sentence? (Possible response: 18 − 8 = )
- Show or tell how you solved the problem. (Possible response: I sketched a picture. I drew
18 marks and crossed 8 off because there were
8 cubes out of the bag. I circled the other marks
to show that they were still in the bag. I counted
10. See Figure 1.)
- What do you remember about fact families? (Possible responses: There are several number
sentences that have related facts. There are some
addition ones and some subtraction ones. They
all use the same numbers.)
- Is there a way to use fact families to solve this problem? (Possible response: Think about how 8
and 18 are related. 8 + 10 = 18, and its turn-around fact is
10 + 8 = 18. That means
18 − 8 = 10 and 18 − 10 = 8. See Figure 2.)
Ask several other students to present the last number
sentence they were working on. Work as a class to
solve the problems using fact families. Add a fact
families strategy to the Invented Strategies chart.
Play Round Two.
Have student pairs exchange bags
with another pair to change the number of counters in
their bag. They continue playing the game with the
new number of cubes. See the TIMS Tip. Monitor students
as they play and encourage them to try using the
fact family strategy to solve some of their problems
during the game.
Have students switch partners when they exchange bags.
Students will then have an opportunity to discuss strategies
with a different person.
After about another ten minutes, stop the games and
again ask students to identify the most recent problem
they had to solve. They use this problem to answer
Check-In: Question 2 on the How Many in the Bag
pages in the Student Activity Book.

Use Check-In: Question 2 on the How Many in the Bag pages
in the Student Activity Book to assess students' progress
toward representing subtraction situations using counters
and number sentences [E2]; solving separate/take away word
problems involving two whole numbers whose sum is between
10 and 20 [E3]; using mental math strategies and reasoning
strategies (e.g., using doubles, using ten, making ten)
to solve addition problems with sums between 10 and 20 and
the related subtraction problems [E5]; using strategies that
apply the properties of addition (e.g., turn-around, compose
and decompose numbers) to solve subtraction problems
[E6]; finding the unknown whole number in a subtraction
equation relating three whole numbers [E7]; choosing good
tools and efficient strategies for solving problems [MPE2];
and explaining a solution strategy [MPE5].
The Subtraction Circles Master can be customized to provide targeted practice with subtraction.
Play Final Round with 20 Counters. Ask students
to play again. This time, every group should put
20 counters in their bag.
Place more than 20 counters in the bag. Pull some out, or
have partners pull them out, and ask students to tell how
many are left in the bag. Ask them to verbalize their solution
strategies.