Lesson 3

Doubles and Halves

Est. Class Sessions: 2

Developing the Lesson

Part 2: Using Connecting Cubes to Double and Halve

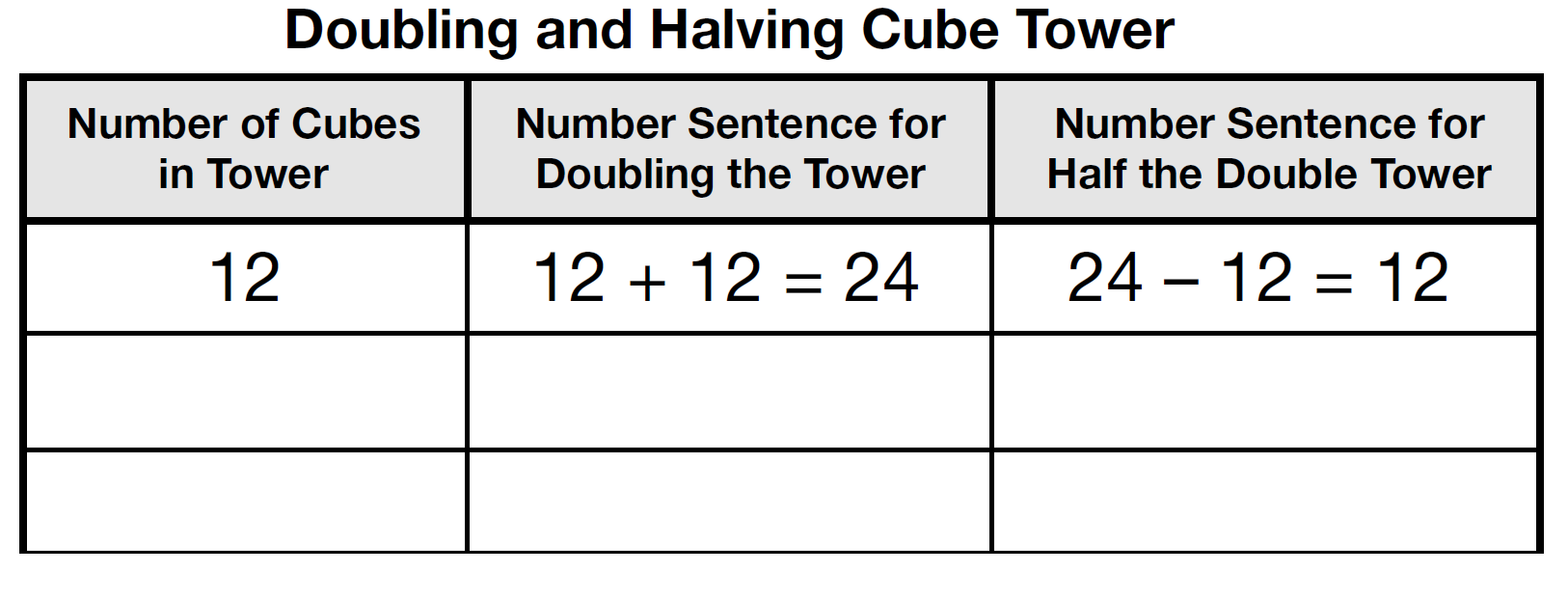
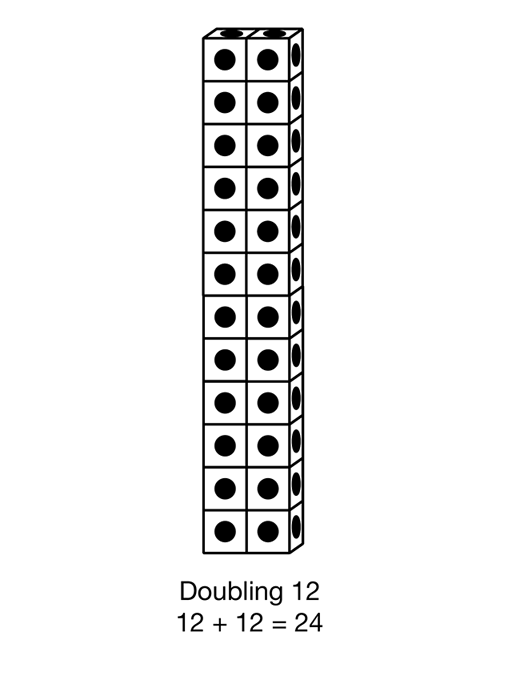
Doubling. Ask student pairs to build a single tower of 12 connecting cubes. Then ask students to build a two-column tower that has double the number of cubes in the first tower as shown in Figure 4.

Ask the following questions.

X
  • How many cubes were in your first tower? (12)
  • How many cubes are in your double tower? (24)
  • How do you know? (Possible response: I counted my cubes by twos.)
  • Give a number sentence that shows how you doubled your tower. (12 + 12 = 24)
  • Now find half of your double tower. (12)
  • Give a number sentence that shows how you halved this tower. (24 − 12 = 12)

As students respond, write their responses in the Doubling and Halving Cube Towers data table you prepared. See Figures 2 and 5.

Continue to ask student pairs to make towers with different numbers of cubes. Numbers should be 15 or less.

Halving. Ask student pairs to build a tower of 14 connecting cubes. Ask students to change the tower so the new tower has half the number of cubes. Elicit suggestions on how you might do this. A student might respond that you will need to break the tower into two towers of the same height.

Ask:

X
  • How many cubes were in your first tower? (14)
  • How many cubes are in your half tower? (7)
  • Give a number sentence that shows how you halved your tower. (14 − 7 = 7)
  • Double your half tower. How many cubes are in your double tower? (14)
  • Give a number sentence that shows how you doubled your tower. (7 + 7 = 14)

Repeat the above procedure with other even numbers less than or equal to 30 as shown in Figure 6.

Student Guide:
Student Activity Book:
Master:
Data table for doubling and halving numbers
X
+
Cube model showing doubling 12
X
+
Data table for halving and doubling 12, 14, and 8
X
+
Data table for halving and doubling
X
+