Lesson 4

Doubles +1, Doubles −1

Est. Class Sessions: 1–2

Summarizing the Lesson

Write the following on the display or board:

Doubles
Doubles +1
Doubles −1
Odd + Odd
Even + Even
Even + Odd

Ask students if they can predict whether the sum described in each statement will be even or odd.

Ask:

X
  • When you find the double of a number is the sum even or odd? (even)
  • How do you know? (Possible response: Each connecting cube in my tower had a partner.)
  • When you find a [double +1 or double −1] is the sum even or odd? (odd)
  • How did you decide? (Possible response: If the double is always even then one less or one more than an even number is always odd.)
  • Is the sum of two odd numbers even or odd? (even)
  • Is the sum of two even numbers even or odd? (even)
  • Is the sum an even and an odd number even or odd? (odd)
  • Do you think these statements are true for all numbers? (Responses will vary.)

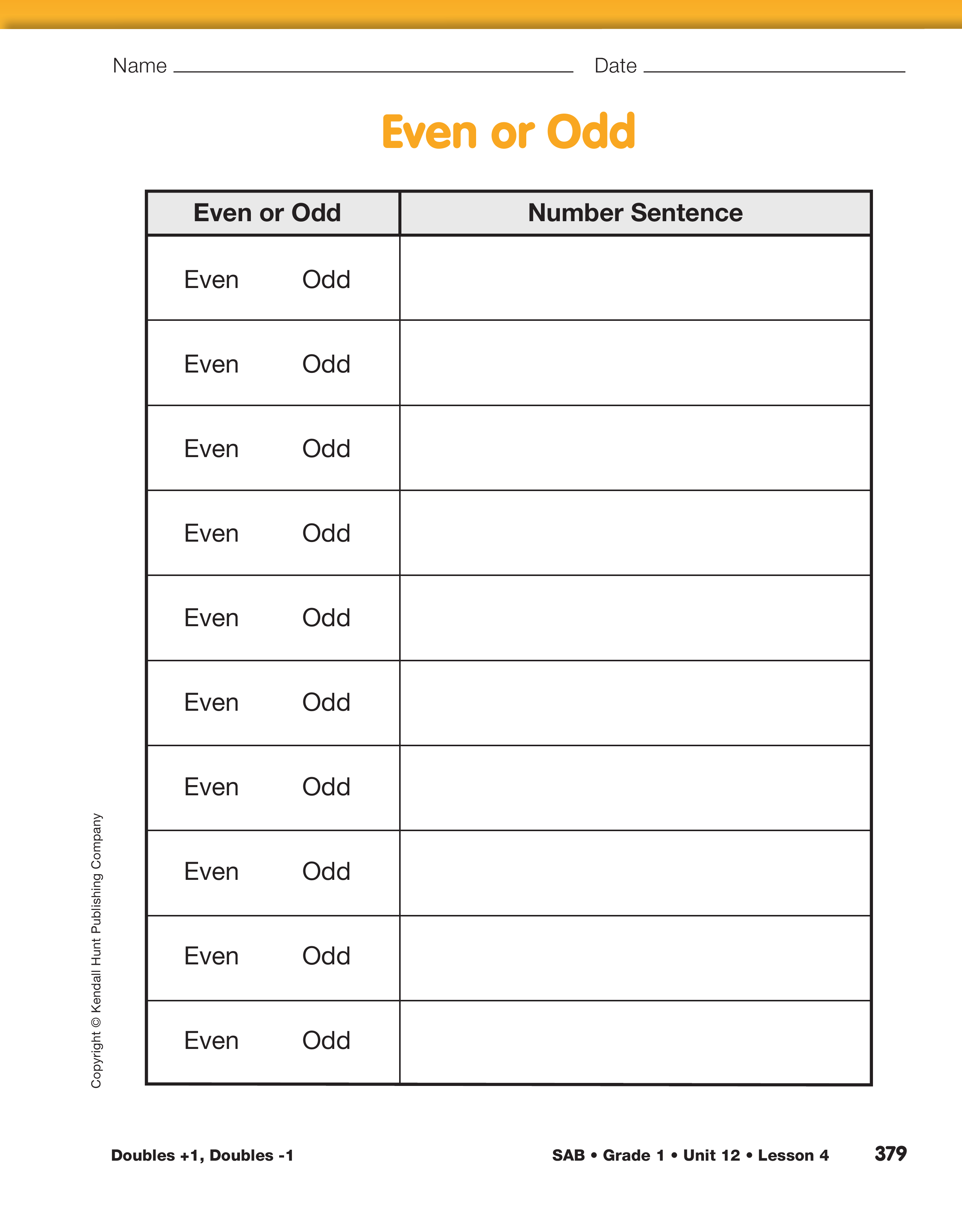
Tell students that they are going to investigate to see if this pattern is true for numbers that are not doubles or near doubles. Display and ask students to remove the first Even or Odd page from the Student Activity Book. Ask students to locate their spinners from the Doubles, Doubles +1, Doubles −1 pages and distribute one set of the Number Cards 1–10 you prepared to each student pair.

Explain that one person in each pair will spin the number spinner to find the first number in an addition number sentence; for example, nine. The second person in the pair will draw a number card to find the second number in the number sentence; for example, three. The pair will then predict if the sum of 9 + 3 will be even or odd and circle their prediction in the table on the Even or Odd page in the Student Activity Book. After making a prediction, they record the number sentence and sum to check their work. Ask a volunteer to play one or two rounds with you to demonstrate how to choose the numbers and fill in the table.

After students work with their partner to fill in several rows on the table, ask:

X
  • What did you notice about the sum in an addition sentence adding two even numbers? (The sum is always even.)
  • Why do you think the sum of two even numbers will always be even? (Possible responses: You can count even numbers by two and there will not be any left over.)
  • What do you notice about the sum of two odd numbers? (The sum is always even.)
  • Why do you think the sum of two odd numbers will be even? (Possible response: When you have an odd number you can count by twos with one left over. If you have two odd numbers they will each have one left over. The two leftovers make a pair so the number is even.)
  • What do you notice about the sum of an even number and an odd number? (The sum is always odd.)
  • Why do you think the sum of an even and odd number will be odd? (Possible response: When you count the even number by twos there won't be any left over, but when you count the odd number there will be one left without a partner, so the answer will be odd.)

This is a challenging discussion and it is not necessary for all students to come to these conclusions at this time.

Ask students to summarize their thinking by completing Check-In: Questions 1–3 on the Even or Odd pages.

X
Use Check-In: Questions 1–3 on the Even or Odd pages in the Student Activity Book to assess students' abilities to explain their thinking [MPE5].
Student Guide:
Student Activity Book: 379 380
Answers
X
SAB_Mini
+
X
SAB_Mini
+
Master: