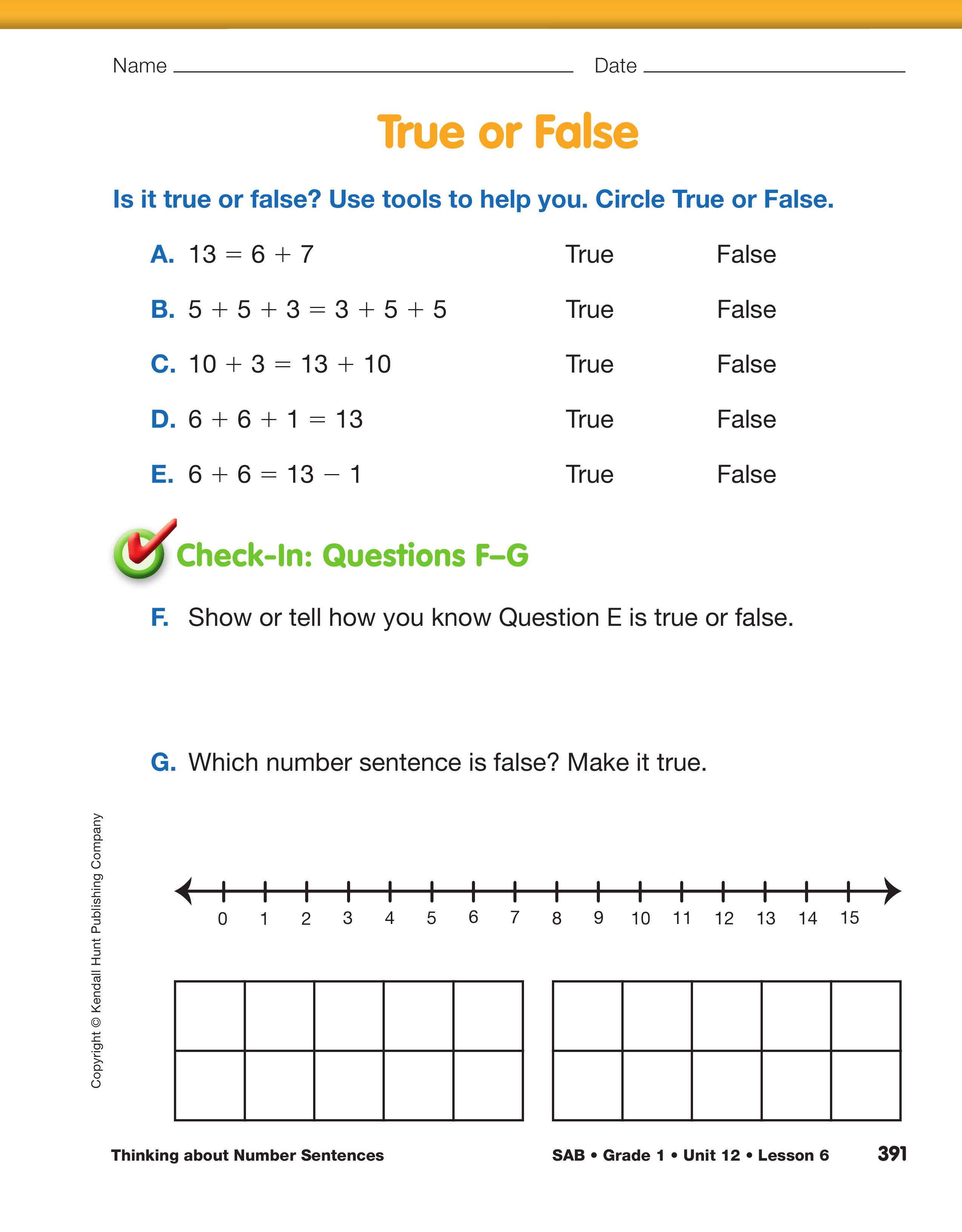
Describe Strategies for Subtraction Facts. Display
the three sheets of chart paper you prepared and display
the Show Your Strategy Cards you prepared
showing the subtraction facts in Group 1. See
Materials Preparation. Focus students' attention on
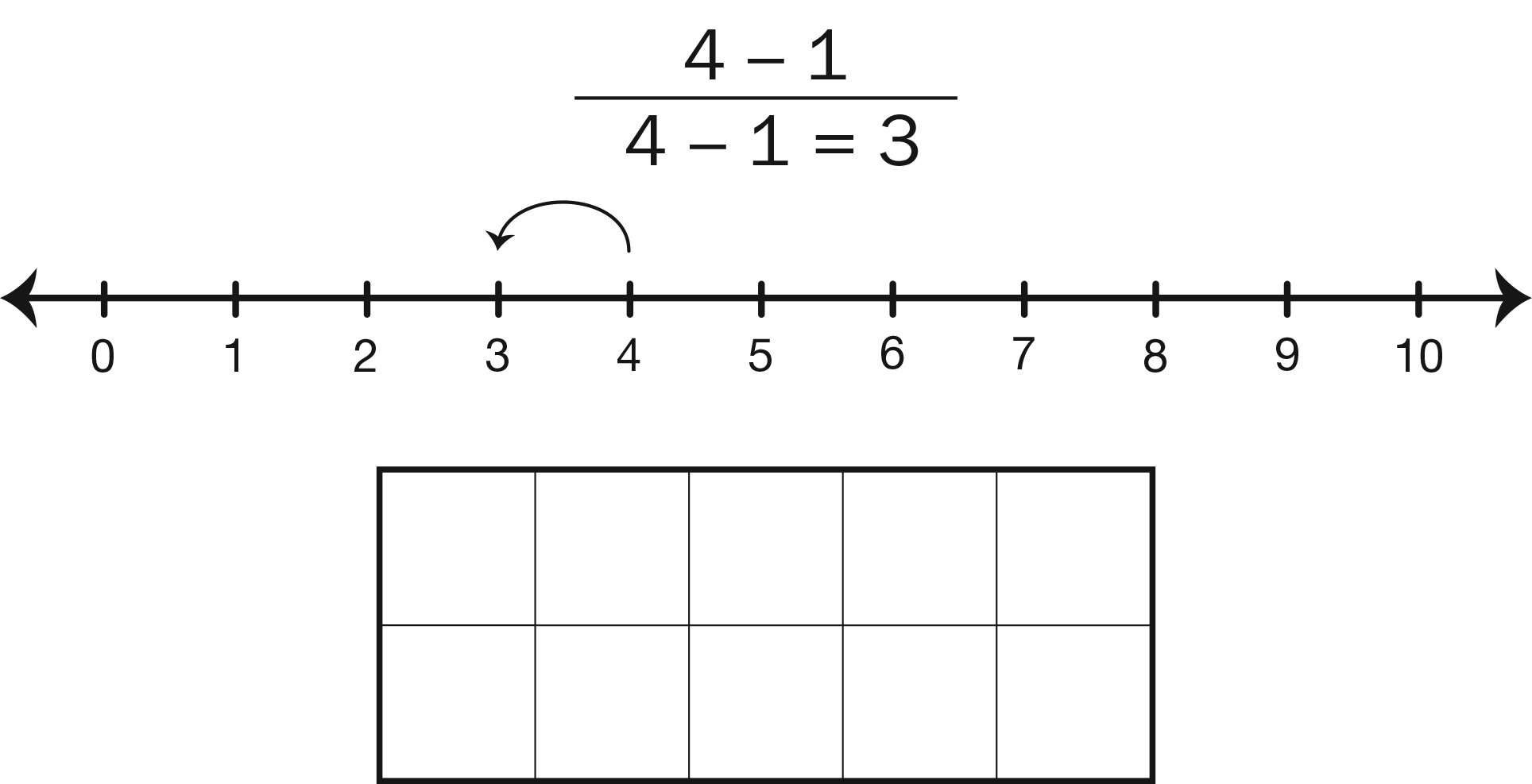
the card showing 4 – 1 and ask students to solve
4 – 1.
- Who would like to show the class how to solve
4 – 1?
As a student describes his or her thinking, record
that solution on the corresponding card using either
the number line, ten frame, or number sentences.
- How would you describe your strategy? (Possible
response: I know 3 is one less than 4 so
4 – 1 = 3; I counted up from 1 and made 3 hops
on the number line.
- Would you describe your strategy as counting up
or counting back? (Possible response: counting
back; counting up)
If a student is counting all, ask him or her if there is
another strategy that may be more efficient. Move
this card to the chart that corresponds to the student's
strategy (e.g., Counting Up or Counting Back
chart). Focus students' attention back to the Show
Your Strategy Cards for Group 1 displayed.
- Would you use a similar strategy for any of these
other subtraction problems? Which ones?
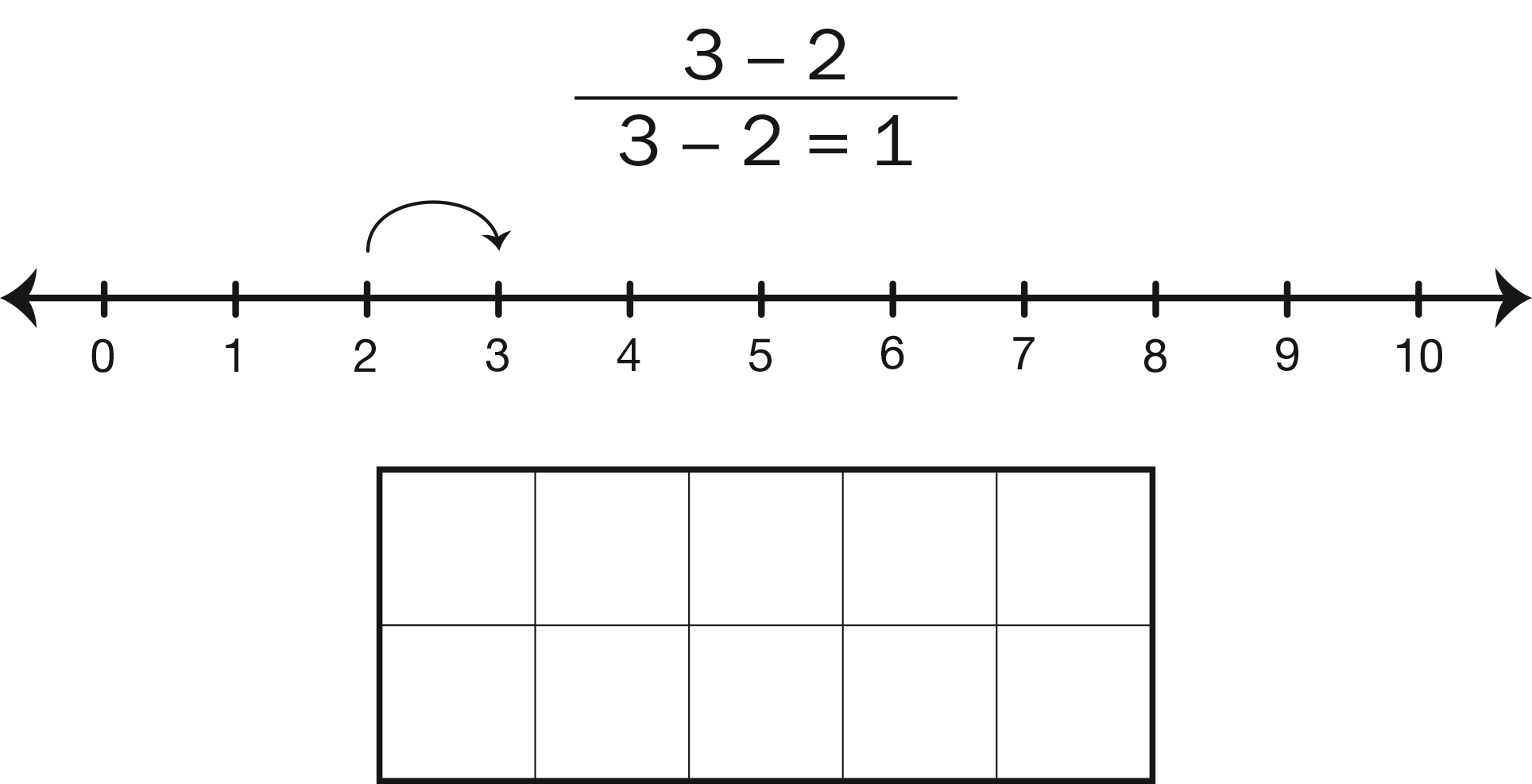
(Possible response: 2 – 1, 3 – 1, or 3 – 2)
- Share your strategy for [2 – 1].
As a student shares his or her strategy, record it on
the card and post it on the appropriate chart. See
Figures 2 and 3. Continue so 2 – 1, 4 – 1, 3 – 1,
and 3 – 2 are on the charts.
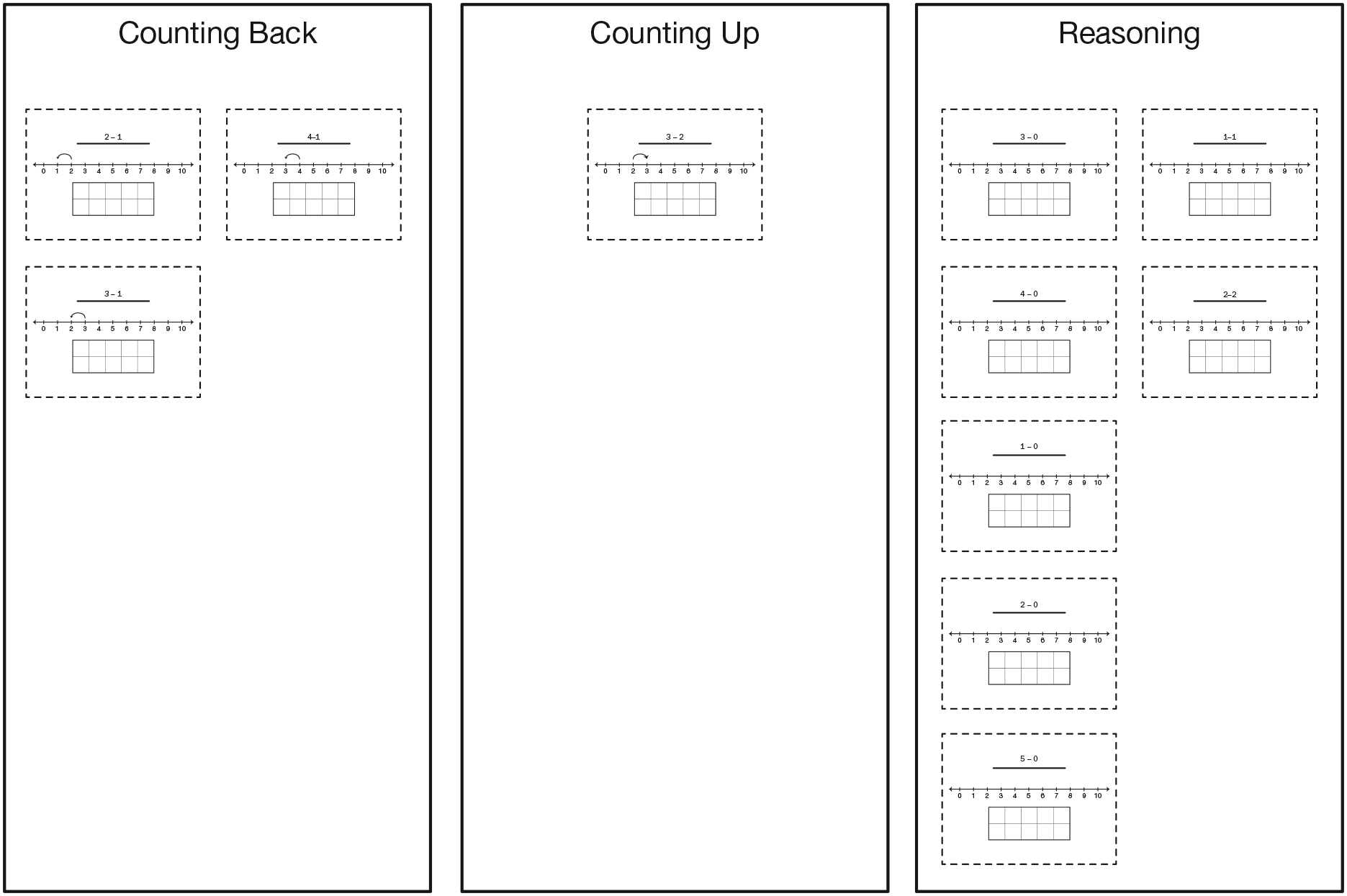
Direct students' focus to the cards showing 1 – 1
and 2 – 2.
-
What is the solution to these subtraction problems?
(zero)
-
How do you know? (Possible response: I thought
of a story. I have 1 apple and 1 is taken away so
now I have zero apples.)
-
Did you use a counting strategy? (No, I used a
reasoning strategy.)
Place these cards on the Reasoning chart. Now direct
students’ attention to the remaining five cards (e.g.,
1 – 0, 2 – 0, 3 – 0, 4 – 0, 5 – 0).
-
What is the same about all of these subtraction
facts? (Possible response: In each you are subtracting
zero from a given number.)
- What is the solution to [3 – 0]? (3)
- Would you use the same strategy for each of these
problems? (Yes.)
- Which chart should these subtraction facts be
placed on? (Possible response: the “Reasoning”
chart)
Figure 4 shows the subtraction facts in Group 1
sorted onto the three charts.

Students have been inventing and using counting strategies
to solve problems using subtraction throughout the school
year. In this lesson, students will focus on formalizing the
counting strategies and deciding which counting strategy
(counting up or counting back) is more efficient for a given
problem. Students will also explore reasoning strategies
(using ten, using doubles, making ten). This lesson provides
practice and attention to the counting strategies. These
strategies are most commonly used to solve the subtraction
problems related to addition facts with sums to ten. Students
should also be encouraged to think addition to solve a
subtraction problem. This is not teased out as a separate
strategy but is instead part of the reasoning strategies since
students are still developing proficiency with these reasoning
strategies for addition. Students that are fluent with these
subtraction facts (e.g., 9 – 2) are asked to invent and
practice strategies for facts with larger numbers (e.g.,
16 – 9). This development is supported with visual cues and
the addition strategies students are confident with.
Sort Subtraction Facts in Groups 2–3. Now that
students understand the strategy categories, distribute
the facts on the prepared Show Your Strategy
Cards in Groups 2 and 3. There are 23 facts in these
groups. Give at least one fact to each student. Ask
students to solve the subtraction problem and show
their work. Remind students that they can show their
strategy on the number line, with the ten frame, or
with number sentences. As students complete their
card, ask them to attach it to the chart that corresponds
to the strategy that they used to solve the
problem.
-
What strategy did you use?
- Did you use a counting up, counting back, or a reasoning strategy?
Some students may have trouble naming their strategy.
Allow students to place their card on the chart
they think is appropriate. If they do not choose the
appropriate chart, there will be a chance to rearrange
the cards.
If you have a small number of students, eliminate the facts
that involve zero and maybe those that involve subtracting
one, since the strategies used to subtract one are already
represented. If you have a large number of students, ask
students to work in pairs and possibly eliminate the same
facts.
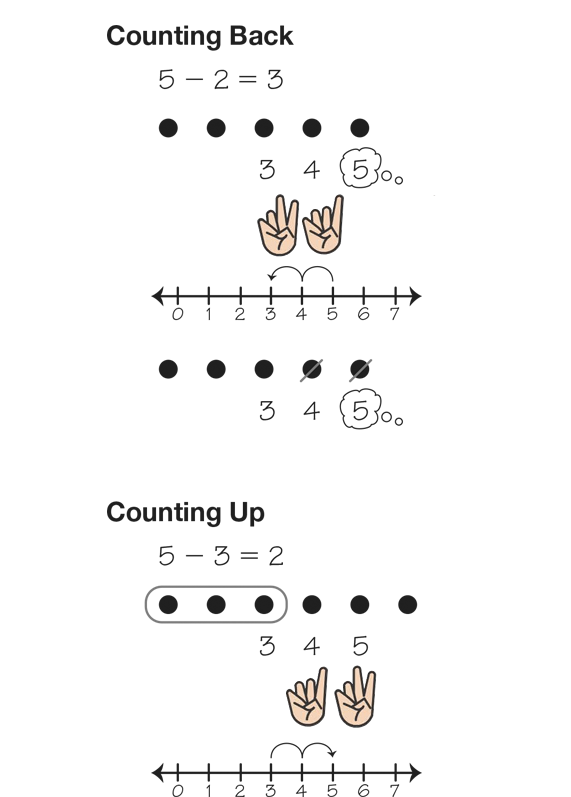
Discuss Counting Strategies for Subtraction. Now
that all the facts in Groups 1–3 are posted, discuss
the differences between the counting up and counting
back strategies. See Figure 5 for examples of
these strategies. Direct students’ attention to the facts
on the Counting Back chart.
-
Describe the counting back strategy. (Possible
response: The counting back strategy is just like
counting on. You put the one number in your
head and you count back or hop back.)
-
What are some different tools you used to show
counting back? (the number line, counters, my
fingers)
-
Are all these facts placed on the right chart?
-
Does anyone want to change where you placed
your subtraction problem?
Look at the facts shown on the cards on the
Counting Up chart.
-
Describe the counting up strategy. (Possible
response: When I count up, I start with the
smaller number and count to the larger number. I
then count the number of hops.)
-
What are some different tools you used to show
counting up? (the number line, counters, my fingers)
-
Are all these facts placed on the right chart?
-
Does anyone want to change where you placed
your subtraction problem?
Sort Subtraction Facts in Groups 4–6. Continue
this analysis by distributing the facts on the prepared
Show Your Strategy Cards in Groups 4–6. There are
31 facts in these groups. Give at least one fact to
each student. Ask students to solve the subtraction
problem and show their work. Remind students that
they can show their strategy on the number line, with
the ten frame, or with number sentences. As students
complete their card, ask them to attach it to the chart
that corresponds to the strategy that they used to
solve the problem.
- What strategy did you use?
- Did you use a counting up, counting back, or a
reasoning strategy?
As students finish showing how they solved their assigned
problem, you may give them a second fact to solve from the
remaining cards. If the class has a small number of students,
give each student two and/or eliminate facts that would use
strategies already represented.
Discuss Advantages of Each Counting Strategy.
Direct students’ attention to the subtraction facts on
the Counting Up and Counting Back charts.
- When does it make sense to use a counting back
strategy? (Possible response: when you are subtracting
a small number like 1, 2, or 3 like in
5 – 2)
- When does it make sense to use a counting up
strategy? (Possible response: when the two numbers
are close together like 9 – 8)
Discuss Reasoning Strategies. Direct students’
attention to the subtraction facts on the Reasoning
chart. Ask a few students to share their reasoning
strategies.
Use similar prompts to discuss some of
the reasoning strategies.
-
What strategy did you use to solve [9 – 4]?
(Possible response: 9 – 4 = 5 because
10 – 4 = 6 so 9 – 4 is one less or 5.)
-
Is this strategy similar to [student name]’s strategy?
-
What other subtraction problems were solved
using the same strategy? (Possible response
when comparing to 9 – 4: 9 – 6, 9 – 5, 8 – 5)
-
What tools did you use to show your reasoning?
(number lines, ten frames, pictures, number sentences)
-
What do you think this strategy should be called?
(Responses will vary.)
Continue until a few different strategies have been shared. See Figure 6.
Naming Strategies. There are many different ways to name
a strategy. Encourage students to use names that are
meaningful and will help them remember the strategy.
Names can be related to the steps in the strategy like
counting back or counting up. Names can also be selected
because students associate the strategy with a particular
student in the class who regularly uses a strategy. It is not
important that students be able to name the strategy, though
it does help them discuss and distinguish them.
Compare to Subtraction Strategies Menu.
Referring to the Counting On, Counting Back, and
Reasoning strategies charts, tell students that they
have an extensive list of strategies. Display and ask
students to refer to the Subtraction Strategies Menu
in the Student Activity Book. See Figure 6. Ask students
to compare the Subtraction Strategies Menu to
the strategies they collected on the charts.
-
What is the same?
-
What is different?
-
Are there any strategies missing that you would
like to add? (Responses will vary: Thinking
Addition, Reasoning about Zero, etc.)
-
Are there strategies on the menu that were not on
our chart?
-
Which problems make sense to solve using the
[using ten] strategy?
Ask students to remove the Subtraction Strategies
Menu from the Student Activity Book and add strategies
not represented to the box on the menu or to the
back of the menu.