Addition
Est. Class Sessions: 2–3Developing the Lesson
Part 1. Sharing Addition Strategies
Write the following problems on the board or on chart paper.
35 + 70
173 + 238
113 + 11
199 + 202
637 + 229
240 + 110
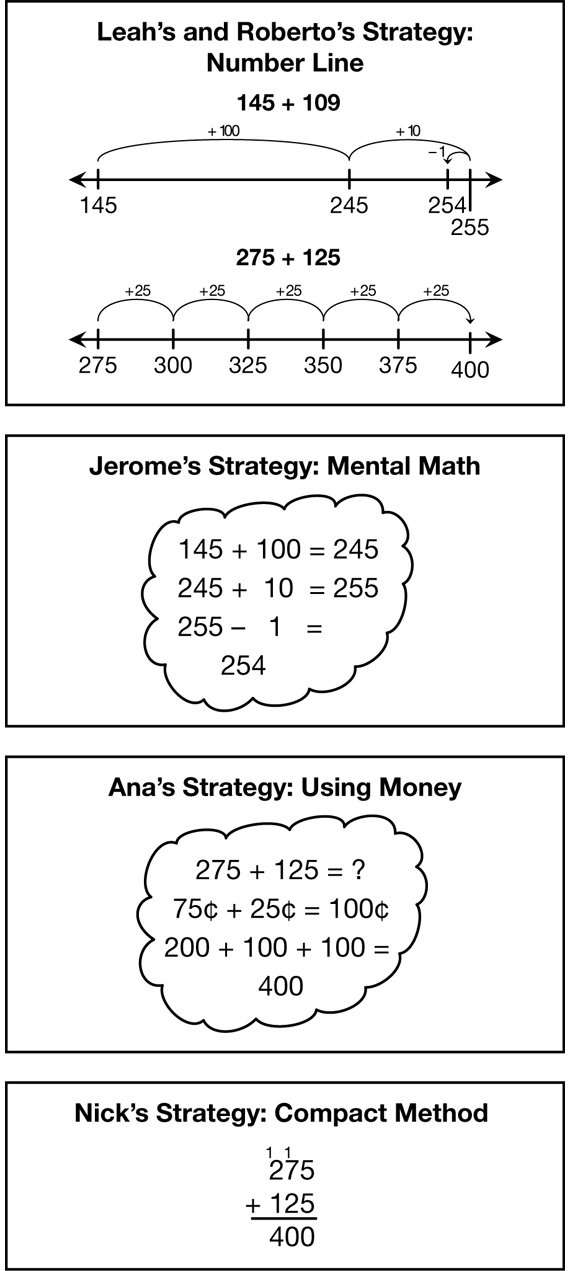
145 + 109
231 + 234
327 + 102
275 + 125
Ask:
After partners have discussed the problems, ask:
Students proficient in one of the paper-and-pencil methods may argue that all the problems are easy. In that case, use the terms paper-and-pencil methods and mental math. Make two columns on the board or on chart paper, as shown in Figure 1. Have students decide in which column they would place each problem. If students disagree about the placement of a problem, you can place it in both columns or make a third column labeled “Both.”
Once students have sorted the problems, choose a few problems and ask students to solve them. Choose problems that may lend themselves to mental math or number line strategies as well as paper-and-pencil methods. Tell students you want to make a list of possible strategies and challenge them to use as many different methods and strategies as possible. Have base-ten pieces available.
As students complete the problems, identify those who will share their strategies with the class. Some students will use strategies they have learned (e.g., expanded form) and others will invent strategies (e.g., thinking money). Try to select a variety of strategies. Ask selected students to record their strategy on a piece of chart paper and to name the strategy they used. See the sample dialog and Figure 2 for strategy examples.
Lead a class discussion about the different strategies used to solve the problems. Ask the selected students to share the solutions they recorded on chart paper. Then post the solutions around the classroom for use later in this lesson.