Round and Estimate. This set of problems serves two primary purposes. The first is to provide practice in adding and subtracting multidigit numbers in the context of real-world problems. The second is to develop students' estimation skills.
To solve the problems in the lesson, students must understand the structure of the problem and then employ the correct addition and subtraction computations to solve it. They must also understand the context of the problem to decide if an estimated answer is called for and what estimation strategies can be used.
Begin by reading with the class the opening vignette on the Adding and Subtracting in Space pages in the Student Guide.
Probe students' understanding of estimation.
- Why might Grace want to estimate rather than find an exact number? (Possible response: Perhaps she only needs to know about how many satellites, or she wants to find the answer quickly in her head.)
- How do you think Grace will estimate the number she is looking for? (Students may suggest rounding the numbers and then adding them together. They may also suggest finding an exact answer and then rounding it after the calculation.)
Finding an exact answer and then rounding that number might look like an estimate, but it is important for students to understand that this is not really estimating. It does not help us arrive at answers more quickly or easily and it does not help us check whether an answer is reasonable.
Data in the table on the first Adding and Subtracting in Space page were obtained from the Center for Space Standards and Innovation in Colorado Springs, Colorado. As a research group, this center provides data services about space assets (satellites) to the science and education community. Satellite data are updated regularly to the Celes Trak website http://celestrak.com/satcat/boxscore.asp. You may wish to use this resource to provide updated information to your class.

Round Numbers. Traditionally, students have been taught that rounding a number means replacing it with the nearest multiple of 10, 100, 1000, etc., according to a set of rules for rounding. Traditional rounding gives one type of convenient number for estimating sums and differences. For example, the sum 98 + 523 can be easily estimated as 100 + 520 if we are rounding to the nearest 10.
However, other types of convenient numbers might be chosen for estimating as well. For example, 32 + 35 can be estimated as 33 + 33, a double which is easy to mentally compute. Or, 124 + 277 can be estimated as the sum of convenient numbers such as 125 + 275. Usually, there are several different choices of convenient numbers that make sense for a problem. The emphasis of this lesson is to help students choose convenient numbers that will help them make logical estimates.
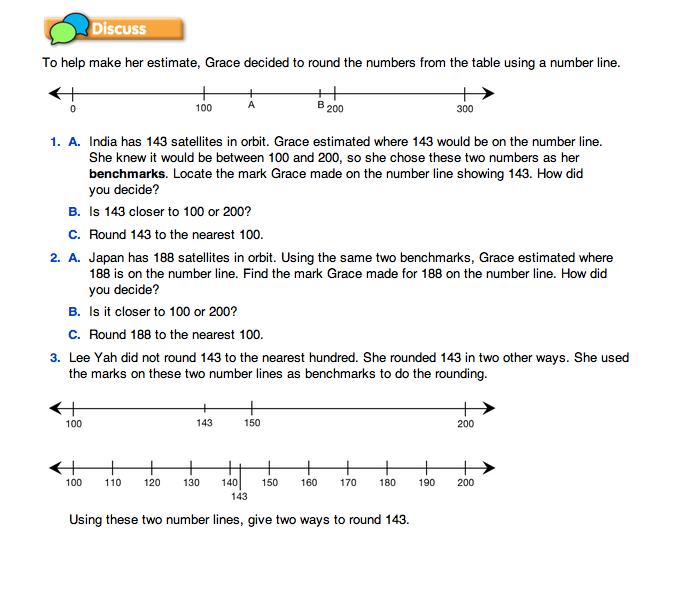
Work through Questions 1–6 together with the class. In these questions, students refer to numbers in the Artificial Satellites in Orbit table in the Student Guide, and then use number lines to round these numbers. In Question 1, students find a rounded number for 143 that is expressed to the nearest hundred. Students are asked to identify benchmarks that they use as they round 143. Explain that benchmarks are convenient numbers that are used for comparison. In this case, the benchmarks are the nearest hundreds above and below the exact value. Question 2 asks students to find a rounded number for 188 expressed to the nearest hundred. Note that the benchmarks for rounding 143 and 188 to the nearest hundred are the same for both numbers. Questions 3 and 4 ask students to round the same numbers to the nearest fifty and the nearest ten.
Have students compare the rounded numbers by asking:
- Which rounded numbers do you think Grace should use to make her calculation? (Answers may vary. Rounding to the nearest hundred is quicker and easier; rounding to the nearest 10 gives a more accurate estimate.)
Question 5 requires students to “think backwards” about how Grace and Lee Yah arrived at their estimated sums by identifying the rounded addends that lead to the different sums. Question 5C leads students to think about the nature of estimation.
As students share their answers about whose estimate is correct, ask:
- Why do you think the student you chose has the correct answer? (Possible response: Lee Yah is correct because her estimate of 330 satellites is much closer to the exact answer of 331 satellites than Grace's estimate.)
- Is either estimate exactly the same as if you added the numbers in the chart? (No. Estimates are not meant to give an exact answer.)
- Would you ever want to estimate the way Grace did, even though her answer was not as close to the exact answer as Lee Yah's? (If you did not need an answer that was very close, or if you needed to calculate an answer very quickly in your head, you would choose Grace's method.)
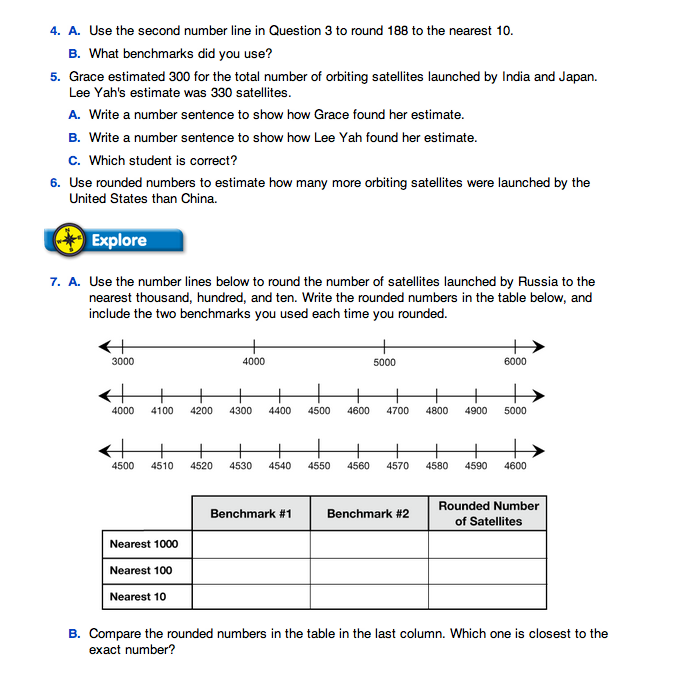
In Question 6, students use rounded numbers to estimate the difference of two large numbers. Have several students share their estimates.
- How did you round each number before subtracting?
- Why did you choose to round to the nearest (ten, hundred, thousand)?
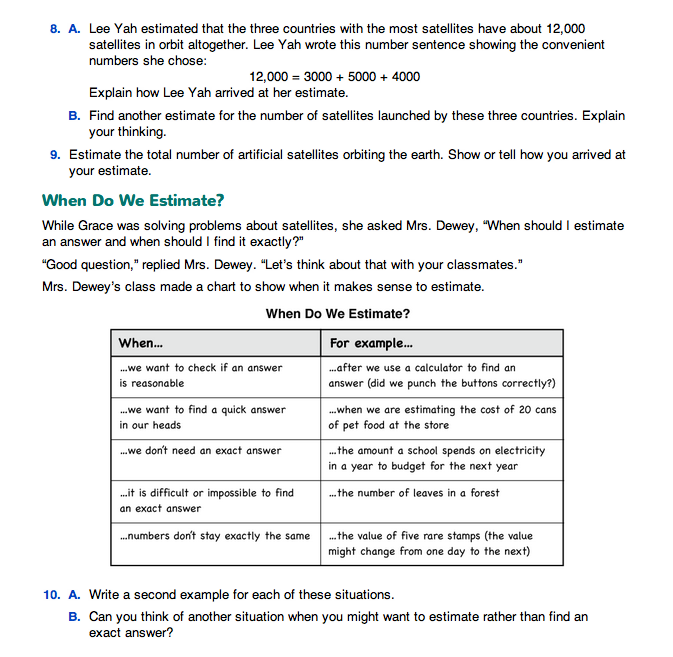
Ask students to complete Questions 7–9 with a partner. These questions involve more practice with rounding numbers and estimating sums and differences. Ask several students to explain their answers to the class.
Decide When to Estimate. Next, read the short vignette in the When Do We Estimate? section. Use a display of the When Do We Estimate? Master in the Teacher Guide to record students' examples of each situation, and of any additional situations they may think of in response to Question 10. For example, they may add that it makes sense to estimate whenever a problem asks “About how many…?”
Assign the Homework section of the Adding and Subtracting in Space pages. Students should be reminded to refer back to the When Do We Estimate? display to decide whether or not to estimate.
In Lesson 4, students were expected to choose appropriately from among mental math and paper-and-pencil strategies for solving addition and subtraction. In this lesson, estimation is added to the selection of choices. Choosing an appropriate strategy is a skill students develop over long periods of time with a variety of experiences. Some problems demand estimation because quantities are not known exactly, while others demand exact answers. Some problems are easy to do using mental math, and others are more difficult. Students develop flexibility and confidence when they have multiple strategies to choose from.