Working with base-ten pieces helps students develop an understanding of the trades involved in subtracting as a foundation for their work with the traditional paper-and-pencil algorithm. Students used base-ten pieces in third grade and they also learned the traditional algorithm used in the United States. However, they may be at different stages of development in their understanding of the connection between place value and the reasons they make trades in the algorithm. Reviewing subtraction with base-ten pieces can help deepen their understanding and thus strengthen their computation skills.
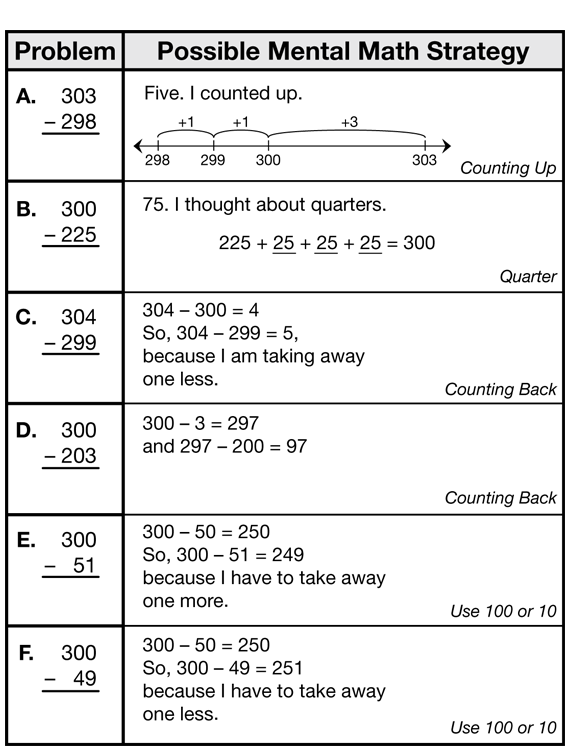
Assign each group a problem from Figure 1. Ask students to solve their assigned problem using a mental math strategy. Tell students to be prepared to share their strategy with the group.
Starting on the Right or Left. Subtracting using the traditional paper-and-pencil method is done from right to left—first the ones column, then the tens column, and so on. It could be done from left to right but that would be inefficient—you would have to go back and recalculate the answer in a column if trades need to be made in the next column.
Because we read from left to right, students naturally tend to start solving problems on the left. For some methods—such as using mental math to subtract tens and ones—starting on the left is reasonable. Subtraction with base-ten pieces can be done in any order.
Ask each group to record and share their solutions on chart paper. Display this collection of mental math strategies in the room for future reference. Ask each group to choose a name for the strategy they used. The name should help students remember something about the strategy.
For the first few subtraction problems students solve, delay mentioning the second number until it is time to subtract. This will ensure that students do not put down pieces to represent both numbers at the beginning of the problem. For example, do not begin by saying, “We are going to solve 576 − 241.” Instead, say, “Please represent the number 576 with base-ten pieces.” Then say, “Now take away pieces that
represent 241.”
Ask students to solve 634 − 271 using any strategy or method they choose. After you have given students a few minutes to work on the problem, ask students to share and record their strategies or methods with the class. Be sure all these strategies are displayed together so the class can reference and compare them. Record mental math strategies as well as paper-and-pencil methods.
Ask students to compare the strategies and ask:
- Which strategy or method do you like best for this problem? Why?
- Do you think a mental math strategy is the best fit for this problem? Why or why not?
- Show an estimation strategy that you could use to check if your answer is reasonable.
Ask students to name the new strategies the class has collected and shared for easy reference during discussions.
When solving subtraction problems, students should manage three piles of pieces—the “bank,” where they can go to trade pieces or get new pieces; the “work pile” that first shows the pieces they start with and then, after the problem is solved, shows the answer; and the “take-away pile” that contains the pieces they have taken away. They can push the take-away pile to the side of their workspace. One way for students to check their work is to add their “take-away pile” to the pieces in their answer to see if they get the minuend.