Remind students to study the multiplication facts for the 2s, 3s, and 9s using the Triangle Flash Cards.
In this unit, students learn the algorithm that is traditionally taught in the United States. To use the algorithm efficiently and accurately, students need a solid understanding of place value in the base-ten system. Therefore, students build on their previous experiences with base-ten pieces to develop meaning for the algorithm. Base-ten pieces are effective if students are given enough time to think for themselves how to compose and decompose numbers in order to take away a quantity. Then students need to connect the process with the base-ten pieces to written symbols (National Research Council, 2001, p. 198; Fuson and Burghardt, 2003).
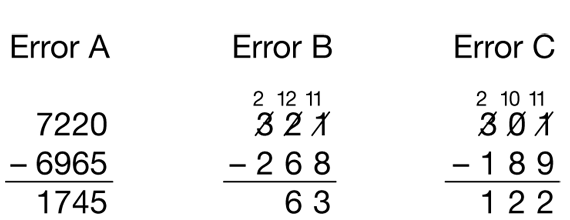
From our research we have found that students sometimes make the errors shown in Figure 18. Similar errors were found in other research studies (Fuson and Burghardt, 2002, p. 286).
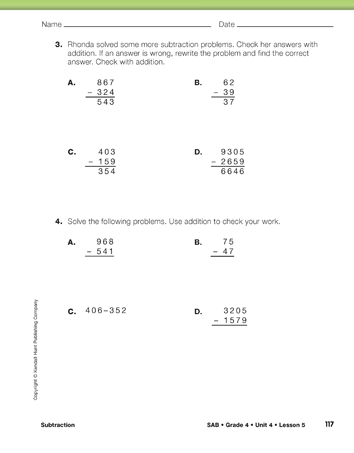
In A, the student subtracted the smaller number from the larger in each column, possibly thinking only of the individual digits instead of the value of each place. In B and C, the students did not trade correctly. Students who make these mistakes often do not understand that when they make trades the value of the top number, the minuend, should not change. Students should be able to look back at their trades and decide if the new partition represents the same number. For example, writing a number sentence for the trades recorded for B (200 + 120 + 11 = 331), shows that the new groupings equal 331 instead of 321. Asking students to think about the value of the digits and to link their written method with base-ten pieces will often help students correct their own errors (Fuson and Burghardt, 2003).
Students often make the mistake in Error C when they must trade across zeros. When students have multiple strategies to choose from, they can solve similar problems using other efficient strategies that may result in greater accuracy. For example, students can first subtract 189 from 299 and then add 2 (299 − 189 = 110; 110 + 2 = 112), or they can count up from 189 (189 + 1 = 190, 190 + 10 = 200, 200 + 101 = 301, so the answer is 1 + 10 + 101 = 112). See the Counting Up strategy in the Subtraction Strategies Menu.