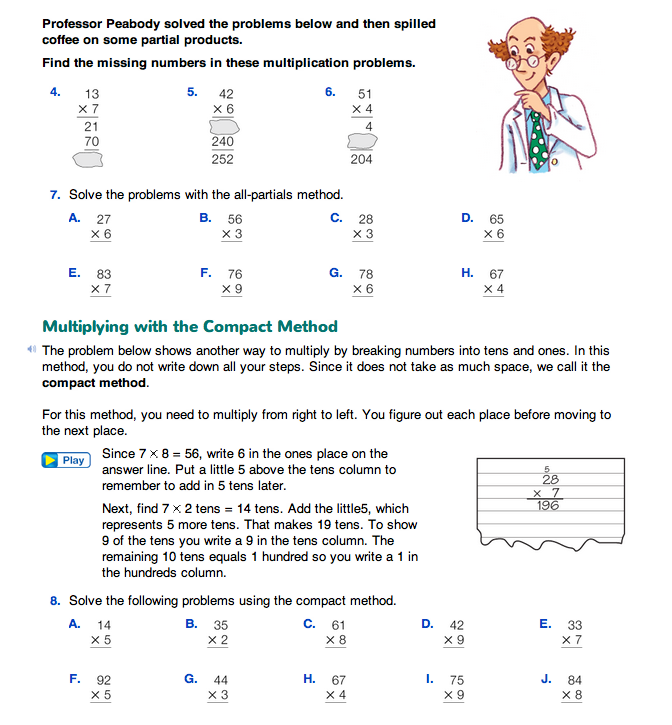
Paper-and-Pencil Multiplication

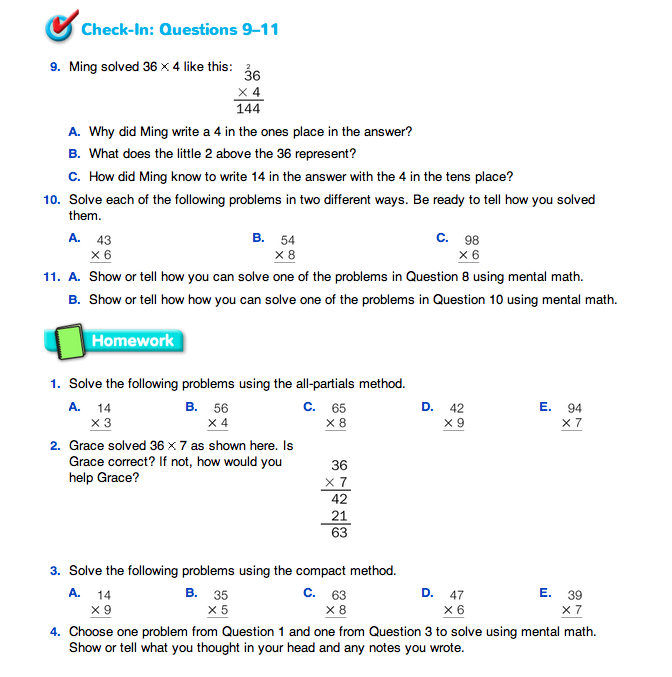
Discuss the product 28 × 7 that is solved with the compact method in the Multiplying with the Compact Method section of the Student Guide. The first step is to multiply 8 × 7 to get 56. This is 5 tens and 6 ones. Write the 6 in the ones column and place a little 5 above the tens column to remind you there are 5 tens that you will need to add in. There are more tens coming from 7 × 20. This is 7 × 2 tens, which is 14 tens. You have 14 tens, plus the 5 tens you “carried,” and that gives 19 tens. You write the 9 in the tens column and since there are no more multiplications, you write the 1 in the hundreds column (because 19 tens is 190, which is 1 hundred and 9 tens.)

Try several problems together. You can redo some of the problems from Question 7 but this time use the compact method. When you feel students are ready, ask them to solve the problems in Question 8.

After students have worked with both the all-partials method and the compact method, they should use whichever method they are most comfortable with. For 2-digit by 1-digit problems—the size of those they encounter in this lesson—the all-partials method does not involve much more writing than the compact method. The all-partials method also has the advantage that it is helpful for estimation and mental math solutions.