Workshop: Division Strategies

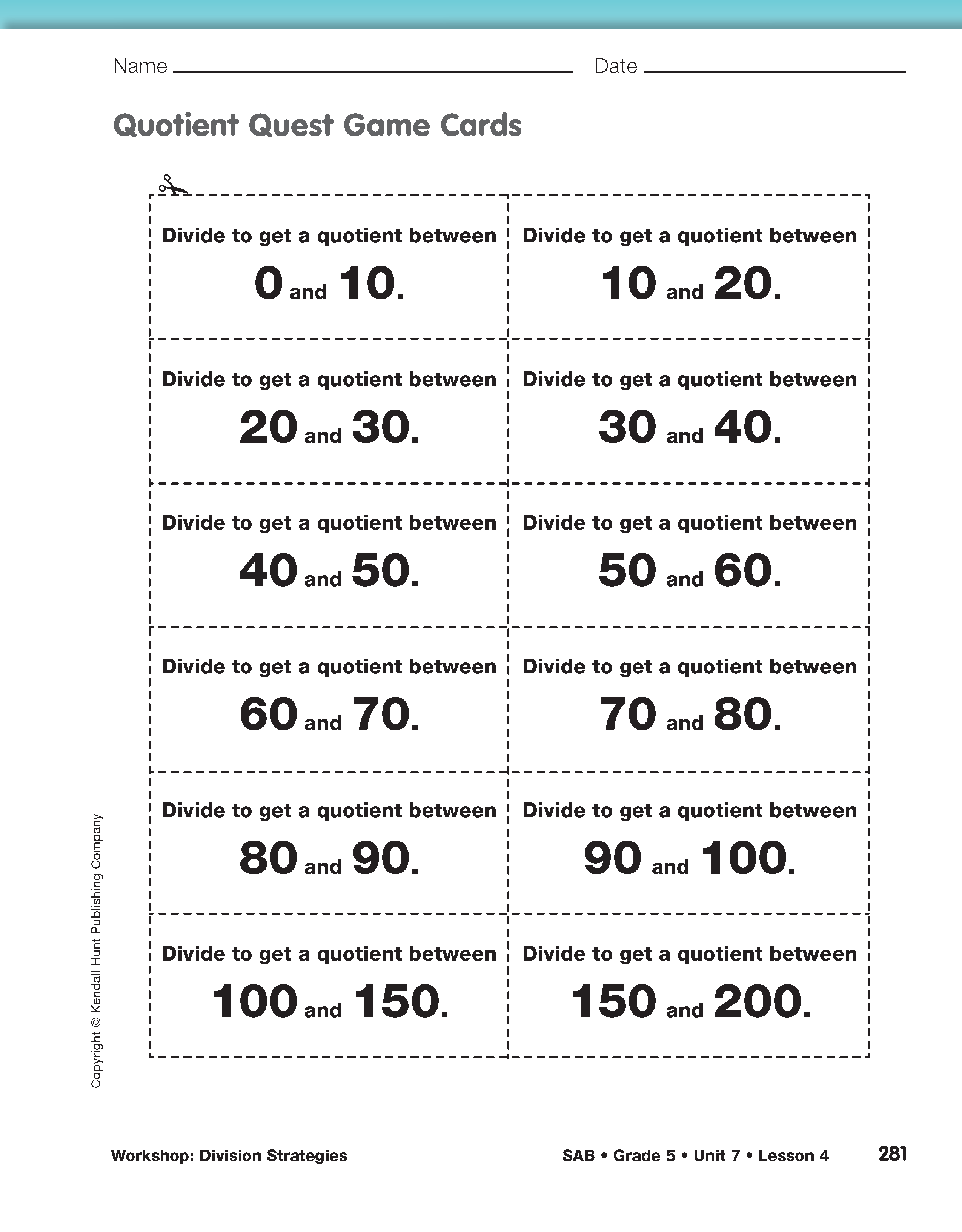
Demonstrate Game. In the Quotient Quest game, students practice estimating quotients. To play, they choose numbers to make a division problem that will have a quotient between two target numbers.

Direct students to the Quotient Quest pages in the Student Activity Book. Students should cut apart the 20 Quotient Quest Digit Cards and the 12 Quotient Quest Game Cards. During the game, they will combine these with a partner to have larger decks of cards.

Ask students to place their Quotient Quest Digit Cards in front of them as you demonstrate the game. Draw or display the game board shown in Figure 1 and ask students to play along using their own Quotient Quest Game Boards from the Student Activity Book.

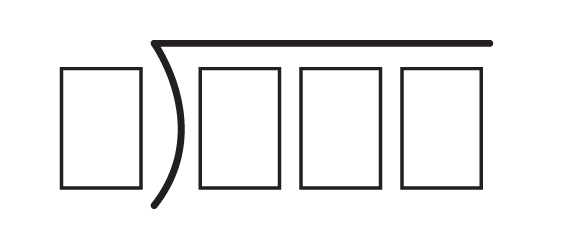
Draw a card from the display set of Quotient Quest Game Cards you prepared prior to the lesson and read the card aloud. See Materials Preparation. The goal is to place digit cards into either set of boxes to make a quotient that matches the game card. For example, if the game card reads, “Divide to get a quotient between 50 and 60,” digits may be placed into the boxes as shown in Figure 2. Demonstrate this solution using your display set of Quotient Quest Digit Cards.

This quotient matches the card because 392 ÷ 7 = 56, which lies between 50 and 60. There are many other quotients that match as well.

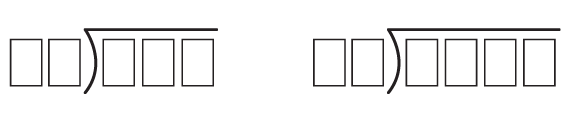
Students may use a variety of strategies for finding quotients that match a given game card. Figure 3 shows a student's possible line of thinking for finding the quotient shown in Figure 2 if they have only digit cards with 2, 3, 7, and 9.

Encourage students to use the Division Strategies Menu in the Student Guide Reference section. Students may use calculators to check quotients, but encourage them to use multiplication and mental math strategies first. See Meeting Individual Needs.

It is important to note to students that in this example, a quotient of exactly 50 or 60 would not match the card because it does not lie between 50 and 60. Once a quotient has been verified, demonstrate removing the digit cards from the board and placing them on a discard stack. Explain that the object of the game is to get rid of digit cards this way and to have the lowest sum of digits on your remaining digit cards at the end of the game. Draw one or two more game cards to further demonstrate playing the game.

Note whether students are using multiplication and estimation strategies to find quotients that match the game cards. After demonstrating a few more turns, display and review the Quotient Quest direction in the Student Activity Book with the class.

Play Quotient Quest. Have students play the game in pairs as you monitor their use of estimation and multiplication strategies. If needed, to shorten the game, players can deal 10 digit cards per player instead of 20. See Meeting Individual Needs for other modifications.

Share Estimation Strategies. After students have played the game, discuss the estimation strategies students used while playing Quotient Quest.