Modeling Division

Read with the class the introductory problem in The Rectangle Model section in the Student Guide. This problem establishes a rectangle model for solving division problems. This model is based on the rectangle model for multiplication. The key difference is that, in the case of division, the area and one of the side lengths of the rectangle are known and the other side length is the quantity to be found.

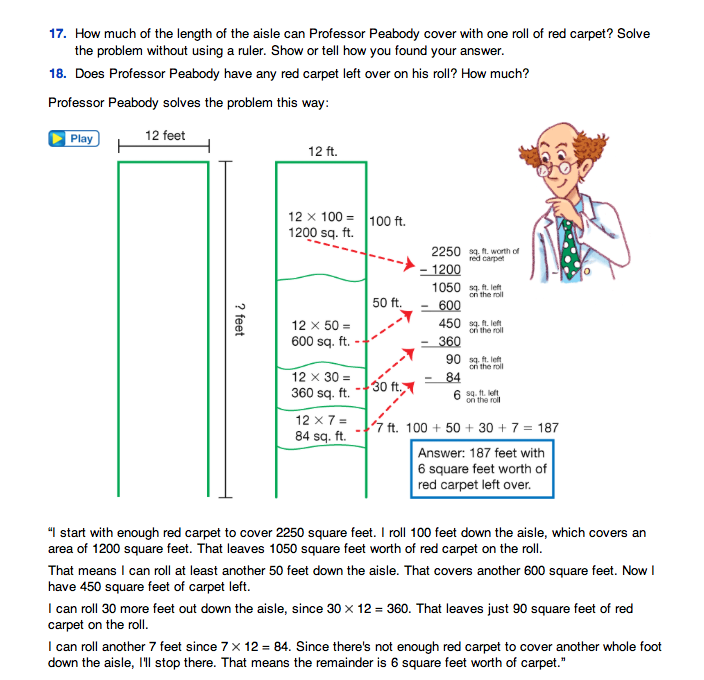
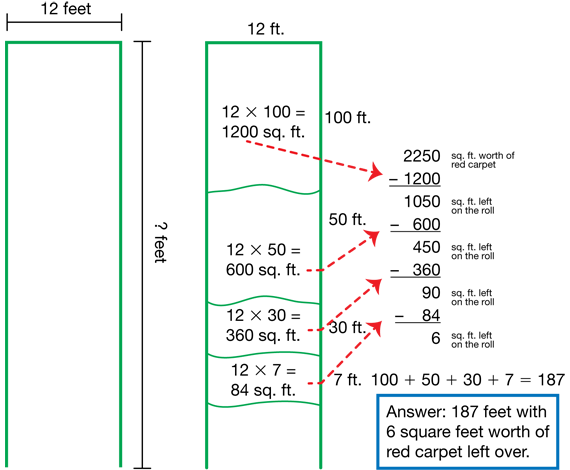
In this problem, a roll of red carpet is known to cover a given area (2250 sq. ft.) down an aisle. The width of the aisle is also known (12 ft.), but the length of aisle that can be covered is not. In this context, the area represents the dividend, the aisle width represents the divisor, and the unknown length represents the quotient. See Figure 3.

Questions 15–18 help students picture the situation in this context.

After allowing students sufficient time to work on the problem, read through Professor Peabody's solution together. Have students discuss with a partner how their solutions compared to Professor Peabody's (Question 19).

Use Questions 19–22 to help students make sense of Professor Peabody's solution. Questions 21 and 22 deal specifically with the meaning of the remainder. It is important for students to understand that for the procedure to be complete, the remainder must be less than the divisor. If it is not, that means that another partial quotient is possible and the division should continue.

One possible explanation of the remainder for Question 22 is that there is some carpet left on the roll but not enough to cover another whole foot down the aisle because that would take another 12 sq. ft. of carpet. Students may suggest that Professor Peabody use up all the rest of the roll anyway. It would get him a little further down the aisle but not a whole foot further.

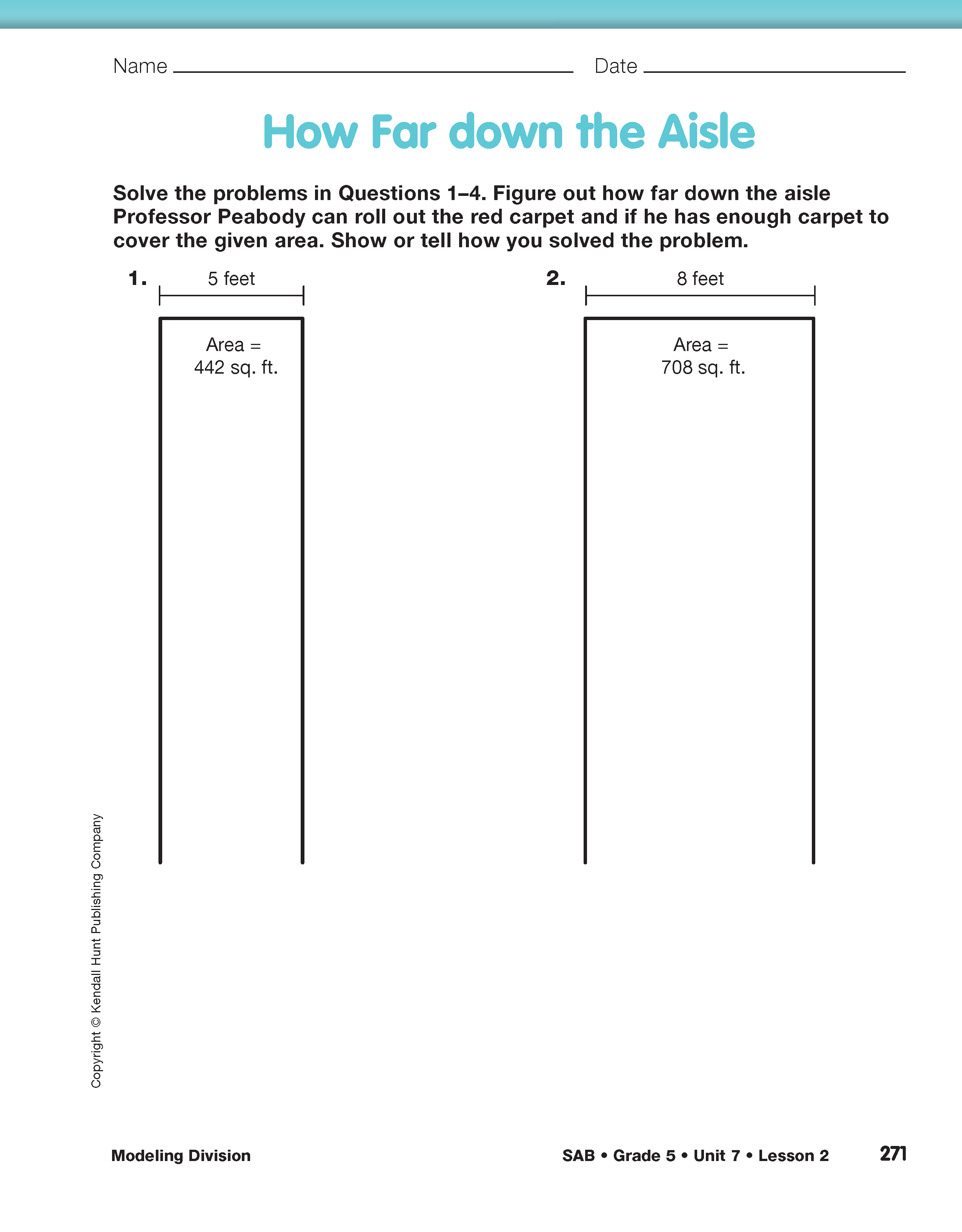
Have students complete the How Far down the Aisle pages in the Student Activity Book to practice using the rectangle model to solve division problems with one-digit divisors.